If a and bare odd numbers, then which of the following is even ?
a + b + ab
a+b-1
a + b +1
a + b + 2ab
The correct Answer is:D
To determine which of the given expressions is even when a and b are both odd numbers, we can analyze each option step by step.
1. Understanding Odd Numbers:
- An odd number can be expressed in the form 2n+1, where n is an integer.
- For example, if a and b are odd, we can write:
a=2m+1andb=2n+1
for some integers m and n.
2. Calculating a+b:
- The sum of two odd numbers:
a+b=(2m+1)+(2n+1)=2m+2n+2=2(m+n+1)
- This result is even since it is divisible by 2.
3. Calculating ab:
- The product of two odd numbers:
ab=(2m+1)(2n+1)=4mn+2m+2n+1=2(2mn+m+n)+1
- This result is odd since it is of the form 2k+1.
4. Evaluating Each Option:
- Option 1: a+b+ab
- We know a+b is even and ab is odd.
- Even + Odd = Odd.
- Option 2: a+b−1
- a+b is even, and subtracting 1 from an even number gives us an odd number.
- Option 3: a+b+1
- a+b is even, and adding 1 to an even number gives us an odd number.
- Option 4: a+b+2ab
- We know a+b is even and ab is odd.
- 2ab is even (since it is 2×(odd)).
- Even + Even = Even.
5. Conclusion:
- The only expression that results in an even number is:
a+b+2ab
- Therefore, the correct answer is Option 4.
- An odd number can be expressed in the form 2n+1, where n is an integer.
- For example, if a and b are odd, we can write:
a=2m+1andb=2n+1
for some integers m and n.
- The sum of two odd numbers:
a+b=(2m+1)+(2n+1)=2m+2n+2=2(m+n+1)
- This result is even since it is divisible by 2.
- The product of two odd numbers:
ab=(2m+1)(2n+1)=4mn+2m+2n+1=2(2mn+m+n)+1
- This result is odd since it is of the form 2k+1.
- Option 1: a+b+ab
- We know a+b is even and ab is odd.
- Even + Odd = Odd.
- Option 2: a+b−1
- a+b is even, and subtracting 1 from an even number gives us an odd number.
- Option 3: a+b+1
- a+b is even, and adding 1 to an even number gives us an odd number.
- Option 4: a+b+2ab
- We know a+b is even and ab is odd.
- 2ab is even (since it is 2×(odd)).
- Even + Even = Even.
- The only expression that results in an even number is:
a+b+2ab
- Therefore, the correct answer is Option 4.
Similar Questions
Knowledge Check
If k2−25 is an odd integer then which one of the following values gives the even number?
Aa. k2k−1Bb. (3k−3)(2k−2)Cc. (k+5)(k−5)Dd. any two of theseIf p∵'4 is an odd number'' and q : ''43 is an even number'' are two statements, then which of the following statements is equivalent to ~(p⇒q)?
A'4 is an odd number and 43 is an even number''BThe negation of the statement ''4 is not an odd number of 43 is not an even number''CBoth (''4 is an odd number and 43 is an even number'') and (The negation of the stateement ''4 is not an odd number of 43 is not an even number'')D'4 is an odd number and 43 is not an even number''If each of the odd digits in the number 54638 is decreased by 1 and each of the even digits is increased by 1, then which of the following will be the sum of the digits of the new number?
A25B26C28DNone of these
Cube of an odd number is even.
View SolutionWhich of the following statements are true (T) and which are false (F)?
The sum of two whole numbers, one of which is odd and the other is even is an even number.
View SolutionOdd and Even Numbers
View SolutionHow many 9 are followed by an even number and preceded by an odd number in the following series?
469259339467924894
View SolutionStudy the following infromation carefully to answer these questions:
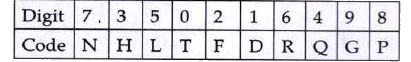
Following condtions are to be observed :
Following conditions are to be observed :
(i) If the first digit is even and the last digits is odd, they are to coded as $ and ∘ respectively.
(ii) If the first digit is odd the last digit is even , they are to be coded as # and ξ respectively.
(iii) If ) is precded as well as followed by an odd digit , then 0 is to be coded as ↑
(iv) If 0 is preceded as well as followed by and even digit, then o is to be coded as ↓.
(v) 0 is not considered as either even or odd.
$QRL↑H∘ could be the code for which of the following number ?
View Solution
