Many tall building have mass dampers, which are antisway devices to prevent them from oscillating in a wind. The device might be a block oscillating at the end of a spring and on a lubricated track. If the building sways, say eastward, the block also moves eastward but delayed enough so that when it finally moves, the building is then moving back westward. Thus, the motion of the oscillator is out of step with the motion of the building. Suppose the block has mass and is designed to oscillate at frequency f= 10.0 Hz and with amplitude .
What is the block's speed as it passes through the equilibrium point?
To find the block's speed as it passes through the equilibrium point, we can use the principles of simple harmonic motion (SHM). Here’s a step-by-step solution:
Step 1: Understand the parameters given
We have the following parameters:
- Mass of the block, m=2.72×105kg
- Frequency, f=10.0Hz
- Amplitude, xm=20.0cm=0.2m
Step 2: Calculate the angular frequency
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Substituting the value of f:
ω=2π×10.0≈62.83rad/s
Step 3: Use the formula for maximum speed in SHM
The maximum speed vmax of a block in simple harmonic motion can be calculated using the formula:
vmax=ωxm
Substituting the values of ω and xm:
vmax=62.83×0.2=12.566m/s
Step 4: Conclusion
Thus, the block's speed as it passes through the equilibrium point is approximately:
v≈12.57m/s
---
We have the following parameters:
- Mass of the block, m=2.72×105kg
- Frequency, f=10.0Hz
- Amplitude, xm=20.0cm=0.2m
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Substituting the value of f:
ω=2π×10.0≈62.83rad/s
The maximum speed vmax of a block in simple harmonic motion can be calculated using the formula:
vmax=ωxm
Substituting the values of ω and xm:
vmax=62.83×0.2=12.566m/s
Thus, the block's speed as it passes through the equilibrium point is approximately:
v≈12.57m/s
Topper's Solved these Questions
- OSCILLATIONSBOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Checkpoint
8 Videos
- OSCILLATIONSBOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Problems
39 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)
10 Videos
- PHOTONS AND MATTER WAVESBOOK - RESNICK AND HALLIDAYCHAPTER - PHOTONS AND MATTER WAVESEXERCISE - PRACTICE QUESTIONS(Integer Type)
4 Videos
Similar Questions
Many tall building have mass dampers, which are antisway devices to prevent them from oscillating in a wind. The device might be a block oscillating at the end of a spring and on a lubricated track. If the building sways, say eastward, the block also moves eastward but delayed enough so that when it finally moves, the building is then moving back westward. Thus, the motion of the oscillator is out of step with the motion of the building. Suppose the block has mass m=2.72×105kg and is designed to oscillate at frequency f= 10.0 Hz and with amplitude xm=20.0cm.
What is the total mechanical energy E of the spring block system?
View SolutionIf a car moves eastward, the force of friction acts northward.
View Solution
Knowledge Check
Block diagram of oscillator consists of
ATank circuitBamplifier circuitCfeed back circuitDall of theseComparing the L-C oscillations with the oscillations of a spring-block system
AL is equivalent to mBC is equivalent to KCcurrent is equivalent to speedDrate of change of current is equivalent to accelerateLogic gates are the building blocks of a :-
Aabacus systemBanalog systemCdigital systemDnone of these
Many builidings have other types of mass dampersas antisway devices ( Refers of Example 14) some like the Johns Honacock building in Boston,have a larger block oscillating at the end of spring and an a lubricated track. .Suppose the block has m=5.44×105kg and is designed to oscillate at a frequency of5√2 Hz and with amplitude xm=0.40m . Find the total mechanical energy E of the spring block system.
Hint :K=mω2=m(2πv)2
E=K+U=12mv2+12kx2
View SolutionWho is the building block of nucleic acid :
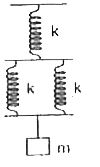
View SolutionCalculate the period of oscillations of block of mass m attached with a set of springs as shown
View SolutionWhen the point of suspendion of pendulum is moved, its period of oscillation
View SolutionAmino acids are the building block of
View Solution
