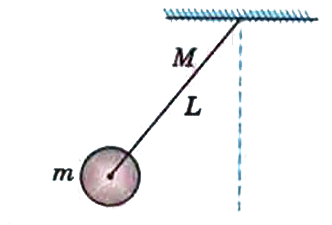
One end of a metal rod of length L and mass M is pivoted to a fixed support and to its free end, a thin disc of mass m and radius r (L) is attached at its centre. In one case, the disc is attached to the rod in such a way that it is not free to rotate about its centre and in another case, teh disc is free to rotate about its centre. When the rod-disc system is displaced slightly from its equilibrium position and released. it peforms S.H.M. in vertical plane. Compare the restoring torque acting on the system and angular frequency in both the cases.
We Know that, torque τ=Iα
where I is the moment of inertia of the system and α is the angular acceleration
for case I : When the disc is not free to rotate about its centre
τ1=I1α1
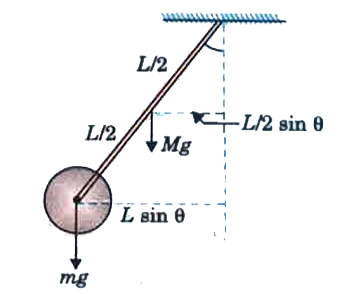
or Mg(L2sinθ)+mg(Lsinθ)
=(ML23+mR22+mL2)α1 ...(i)
for case : II when the rod is free to rotate
or Mg(L2sinθ)+mg(Lsinθ)=(ML23+mL2)α2 ...(ii)
Comparing (i) and (ii), we get
τ1=τ2andI1>I2. so
α1<α2
∴ωA<ωB
Topper's Solved these Questions
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - NCERT FILE (Textbook Exercises)
19 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - NCERT (Additional Exercises)
6 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Conceptual Questions
18 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
Similar Questions
A disc of mass M = 2m and radius R is pivoted at its centre. The disc is free to rotate in the vertical plane about its horizontal axis through its centre O. A particle of mass m is stuck on the periphery of the disc. Find the frequency of small oscillations of the system about its equilibrium position.
View SolutionTwo equal and opposite forces are allplied tangentially to a uniform disc of mass M and radius R as shown in the figure. If the disc is pivoted at its centre and free to rotate in its plane, the angular acceleration of the disc is :

View Solution
Knowledge Check
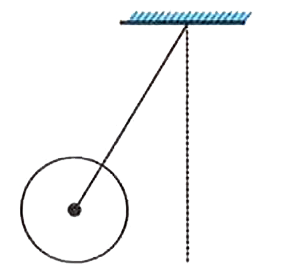
A metal rod of length 'L' and maws 'm' is pivoted at one end A thin disc of mass 'M' and radius 'R' ( Which of the folowing statement (s) is (are) true ?
 ARestoring torque in case A = Restoring torque in case BBRestorings torque in case A lt Restoring torque in case BCAngular frequency for case A gt Angular frequency for case B.DAngular frquency for case A lt Angular frequency for case B.
ARestoring torque in case A = Restoring torque in case BBRestorings torque in case A lt Restoring torque in case BCAngular frequency for case A gt Angular frequency for case B.DAngular frquency for case A lt Angular frequency for case B.A metal rod of length 'L' and mass 'm' is piovted at one end. A thin disk of mass 'M' and radius 'R'(<L). Is attached at its centre to the free end of the rod. Consider two ways the disc is attached: (case A). The disc is not free to rotate about its centre and (case B) the disc is free to rotate about its center. The rod-disc sustem perform SHM in vertical plane after being released from the same displaced potion. Which of the following statement(s) is (are) true?
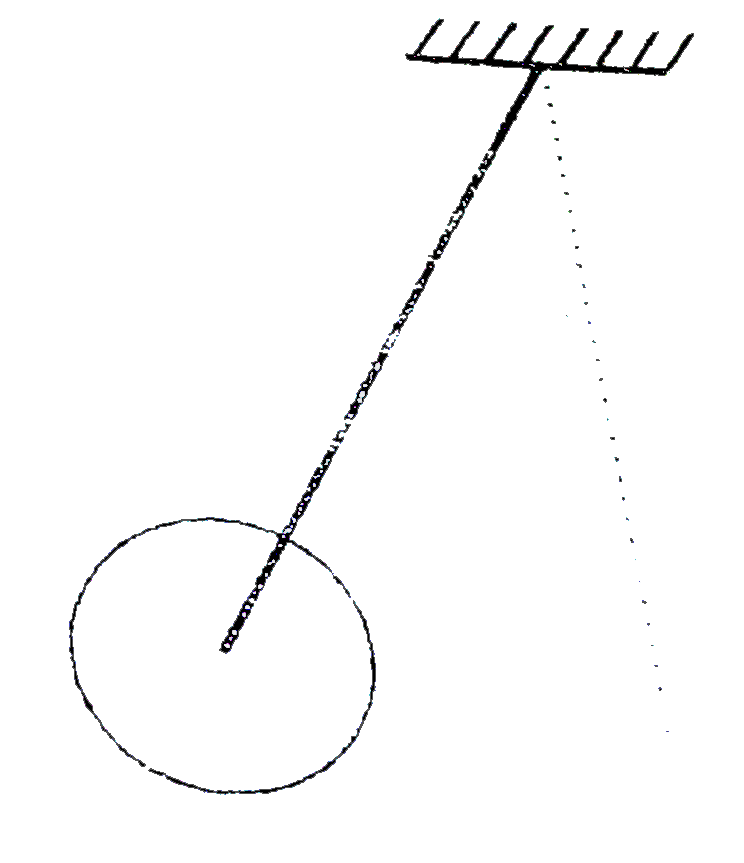 ARestoring torque in case A = Restoring torque in caseBBRestoring torque in case A< Restoring torque in caseBCRestoring frequency case A> Angular frequency for caseBDRestoring frequency case A< Angular frequency for caseB
ARestoring torque in case A = Restoring torque in caseBBRestoring torque in case A< Restoring torque in caseBCRestoring frequency case A> Angular frequency for caseBDRestoring frequency case A< Angular frequency for caseBA uniform thin rod of length 1m and mass 3 kg is attached to a uniform thin circular disc ois
A0.375 mB0.25mC0.125mD0.475m
Find the moment of inertia A of a spherical ball of mass m and radius r attached at the end of a straight rod of mass M and length l, if this system is free to rotate about an axis passing through the end of the rod (end of the rod opposite to sphere).

View SolutionA uniform circular disc of mass M and radius R is pivoted at distance x above the centre of mass of the disc, such that the time period of the disc in the vertical plane is infinite. What is the distance between the pivoted point and centre of mass of the disc ?
View SolutionA disc of mass m and radius r is free to rotate about its centre as shown in the figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. The speed of the block as it descends through a height h, is :-

View SolutionA uniform thin rod of length 1m and mass 3kg is attached to a uniform thin circular disc of radius 30cm and mass 1kg at its centre perpendicular to its plane. The centre of mass of the combination from the centre of disc is
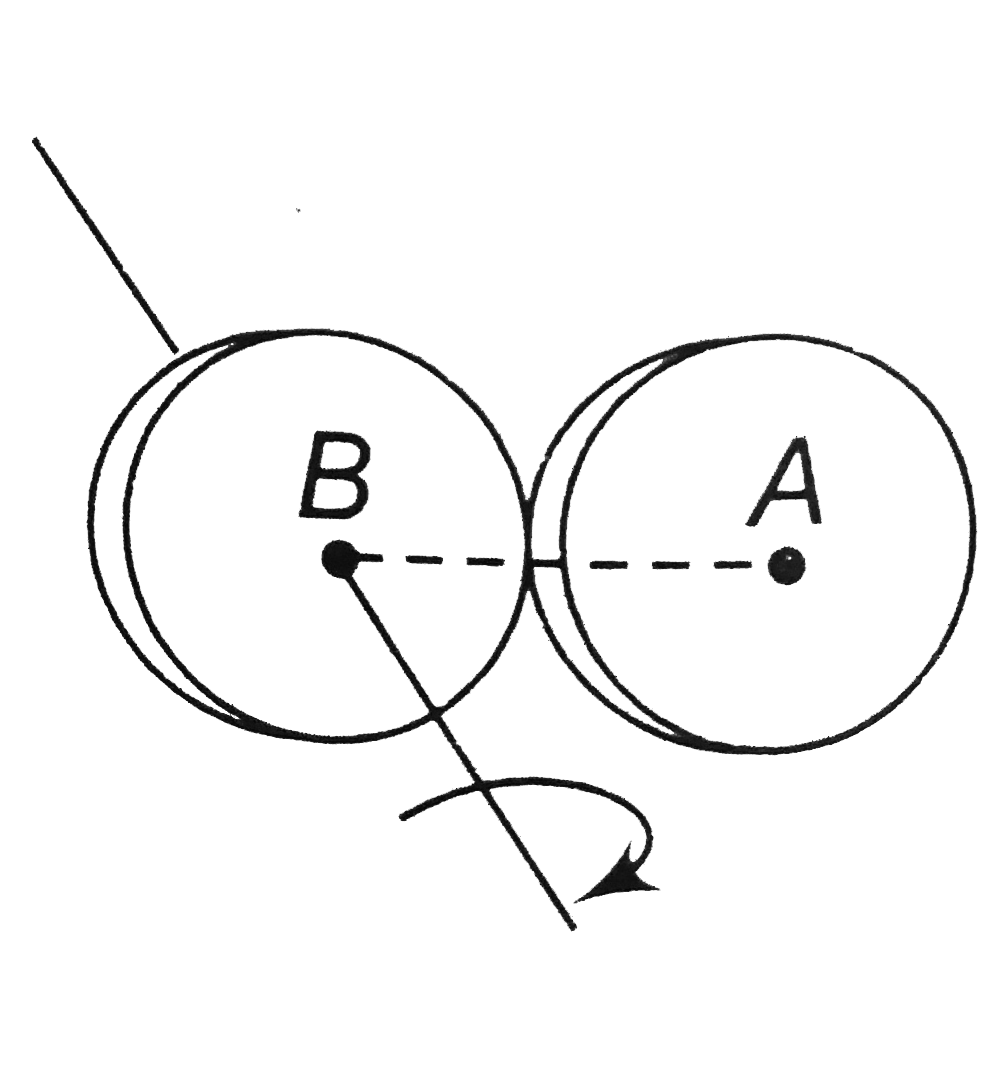
View SolutionTwo thin discs each of mass M and radius r are attached as shown in figure, to from a rigid body. The rotational inertia of this body about an axis perpendicular to the plane of disc B and passing through its centre is :
 .
.
View Solution
