In the Polo hospital some pateints who were suffering from the Hepatites-B were admitted for the treatment, but 9% of the patients were died within half an hour. After treatment, the percentage of patients cured out of the remaining was only 80%. Out of these patients only 70% are completely cured out and the remaining were partially cured out which were equal to 153 patients. The no. of patients (approx.) who were admitted for the treatment for the same was :
400
678
560
700
The correct Answer is:D
To solve the problem step by step, let's break it down:
Step 1: Define the total number of patients admitted
Let the total number of patients admitted to the hospital be x.
Step 2: Calculate the number of patients who died within half an hour
According to the problem, 9% of the patients died within half an hour. Therefore, the number of patients who died is:
Patients died=0.09x
Step 3: Calculate the number of remaining patients
The remaining patients after the deaths are:
Remaining patients=x−0.09x=0.91x
Step 4: Calculate the number of patients cured
Out of the remaining patients, 80% were cured. Thus, the number of patients cured is:
Patients cured=0.80×0.91x=0.728x
Step 5: Determine the number of completely and partially cured patients
Out of the cured patients, 70% were completely cured and 30% were partially cured. Therefore:
- The number of completely cured patients is:
Completely cured=0.70×0.728x=0.5096x
- The number of partially cured patients is:
Partially cured=0.30×0.728x=0.2184x
Step 6: Set up the equation based on the number of partially cured patients
According to the problem, the number of partially cured patients equals 153. Thus, we can set up the equation:
0.2184x=153
Step 7: Solve for x
To find x, we divide both sides of the equation by 0.2184:
x=1530.2184≈700
Conclusion
The approximate number of patients who were admitted for treatment was:
700
Let the total number of patients admitted to the hospital be x.
According to the problem, 9% of the patients died within half an hour. Therefore, the number of patients who died is:
Patients died=0.09x
The remaining patients after the deaths are:
Remaining patients=x−0.09x=0.91x
Out of the remaining patients, 80% were cured. Thus, the number of patients cured is:
Patients cured=0.80×0.91x=0.728x
Out of the cured patients, 70% were completely cured and 30% were partially cured. Therefore:
- The number of completely cured patients is:
Completely cured=0.70×0.728x=0.5096x
- The number of partially cured patients is:
Partially cured=0.30×0.728x=0.2184x
According to the problem, the number of partially cured patients equals 153. Thus, we can set up the equation:
0.2184x=153
To find x, we divide both sides of the equation by 0.2184:
x=1530.2184≈700
The approximate number of patients who were admitted for treatment was:
700
Topper's Solved these Questions
- PERCENTAGESBOOK - ARIHANT SSCCHAPTER - PERCENTAGESEXERCISE - Final round
50 Videos
- PERCENTAGESBOOK - ARIHANT SSCCHAPTER - PERCENTAGESEXERCISE - Exercise (Level - 1)
51 Videos
- PERCENTAGEBOOK - ARIHANT SSCCHAPTER - PERCENTAGEEXERCISE - Higher Skill Level Questions
27 Videos
- PERMUTATIONS & COMBINATIONS BOOK - ARIHANT SSCCHAPTER - PERMUTATIONS & COMBINATIONS EXERCISE - FINAL ROUND
38 Videos
Similar Questions
Knowledge Check
Which of the following computers are used in hospitals to measure the heartbeat of patients?
A400B678C560D700The first in the world to provide firee medicine to the patients were the
AGreeksBChineseCRomansDIndiansIn neurasthenia, the adolescent patient suffers from
AMental and physical weaknessBInability to concentrate and enjoyCInsomnia and depressionDAll the above
Following are the ages (in years) of 300 patients, getting medical treatment in a hospital.
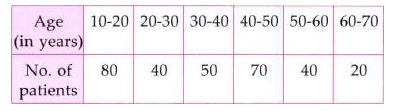
One of the patients is selected at random. Find the probability that the age of the selected patient is
70 years or less.
View SolutionThe specific test which ensures that patient takes the full course of treatment is
View SolutionOut of 100 articles 25 articles were sold at 25% profit and the remaining articles were sold at 25% loss. What will be the total loss percentage?
View SolutionIn a college, 40% of the students were allotted group A, 75% of the remaining were given group B and the remaining 12 students were given group C. Then the number of students who applied for the groups is
View SolutionIn a college, 40% of the students were allotted group A, 75% of the remaining were given group B and the remaining 12 students. were given group C. Then the number of students who applied for the group is
View Solution
