(i) The positive value of k for which kex−x=0 has only one root is

A

1e

B

1

C

e

D

log 2

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

Consider the function f(x)=kex−x.
We have,
f'(x)=kex−1
∴F'(x)=0⇒kex=1⇒ex=1k⇒x=−logk.
The signs of f' (x) for different values of x are as shown below.
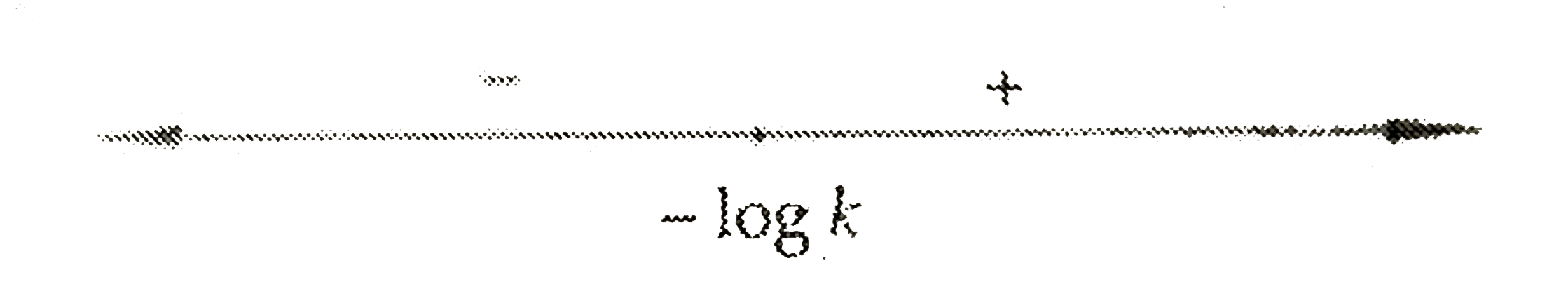
ocr_image
So, f(x) attains its minimum value at x=-logk
Also, the minimum value of f(x) is f(-logk)=1+logk.
Thus, f(x)=0 will have exactly one root if its minimum value 1+log k is zero.
i.e. 1+logk=0⇒k=1/e

Updated on:21/07/2023
|
Class 12MATHSMISCELLANEOUS EQUATIONS AND INEQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If a continous founction of defined on the real line R, assumes positive and negative values in R, then the equation f(x)=0 has a root in R. For example, if it is known that a continuous function f on R is positive at some point and its minimum values is negative, then the equation f(x)=0 has a root in R. Considetr f(x)=kex−x for all real x where k is real constant.
    The positive value of k for which kex−x=0 has only root is

    A1e
    B1
    Ce
    Dloge2

  • If a continous founction of defined on the real line R, assumes positive and negative values in R, then the equation f(x)=0 has a root in R. For example, if it is known that a continuous function f on R is positive at some point and its minimum values is negative, then the equation f(x)=0 has a root in R. Considetr f(x)=kex−x for all real x where k is real constant.
    For k>0, the set of all values of k for which kex−x=0 has two distinct, roots, is

    A(0,1e)
    B(1e,1)
    C(1e,∞)
    D(0,1)

  • Let us consider an equation f(x)=x3−3x+k=0.then the values of k for which the equation has
    Exactly one root which positive , then k belongs to

    A(−∞,−2)
    B(2,∞)
    C(0,2)
    D(−2,0)