a. Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in the figure.
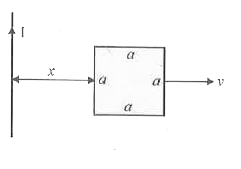
b. Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v=10m/s. Calculate the induced emf in the loop at the instant when x=0.2m. Take a=0.1m and assume that the loop has a large resistance.
 Solution in Tamil
Solution in Tamil
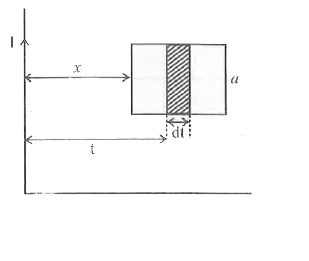
a. Consider a small rectangular element of the square, of thickness dt at a distance .t. from the conductor carrying a current I.
Magnetic field experienced by this element =B=μ0.I2πt
Area of the element = dA=a.dt
∴ Flux, dϕB=B.dA=μ0Ia2πtdt
Hence net flux, ϕB=t=a+x∫t=xμ0Ia2πtdt=μ0Ia2πloge(1+ax)
But ϕB=MI, M - Mutual inductance
∴M=μ0a2πloge(1+ax)
b. ɛ=μ0Ia2v2πx(a+x)=4π×10−7×50×(0.1)2×102π×0.2(0.1+0.2)=1.66×10−5=1.7×10−5V
Topper's Solved these Questions
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - CONTINUOUS EVALUATION (ASSIGNMENTS)2videos
ELECTROMAGNETIC INDUCTION
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - PREVIOUS YEAR QUESTIONS2videos
ELECTROMAGNETIC INDUCTION
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - PRACTICE PROBLEMS FOR SELF ASSESSMENT3videos
ELECTROMAGNETIC INDUCTION
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Competitive Exam Corner20videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - COMPETITIVE EXAM CORNER16videos
ELECTROMAGNETIC WAVES
Similar Questions
Discuss briefly the various methods of producing induced e.m.f.
12:28View SolutionA current carrying wire produces a magnetic field in its surrounding space
A. The S.I. unit of magnetic flux density is
a. henry
b. tesla
c. Am2
d. A-m
B. With the help of a diagram, derive an expression for the magnetic field at the point on the axis of a circular current loop.
C. Consider a tightly wound 100 turn coil of radius 10 cm, carrying a current of 1 A. What is the magnitude of the magnetic field at the centre of the coil?02:54View SolutionA long solenoid with 15 turns per cm has a small loop area 2.0cm2 placed inside the solenoid normal to its axis. If the current carried by the solenoid changed steadiy from 2.0A to 4.0A in 0.1 s, what is the induced emf in the loop while the current is changing?
12:28View SolutionTwo charged spherical conductors of radii R1andR2 are connected by a wire. Then the ratio of surface charge densitites of the spheres σ1/σ2 is
07:04View SolutionIf the total electromagnetic energy falling on a surface is U, then the total momentum delivered (for complete absorption) is
04:06View Solution(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig. (b) Evaluate the induced emf in loop if the wire carries a current of 50 A and the loop has an instantaneous velocity upsilon = 10 ms^(-1) at the location x = 0.2 m, as shown. Take a = 0.1m and assume that the loop has a large resistance.
04:02View SolutionFind the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.
 View Solution
View Solution(a) चित्र 6.21 में दर्शाए अनुसार एक लंबे, सीधे, तार तथा एक वर्गाकार लूप जिसकी एक भुजा की लंबाई a है, के लिए अन्योन्य प्रेरकत्व का व्यंजक प्राप्त कीजिए |
(b) अब मान लीजिए फिर सीधे तार में 50 A की धारा प्रवाहित हो रही है तथा लूप एक स्थिर वेग v=10m/s से दाई ओर को गति कर रहा है | लूप में प्रेरित विद्युत वाहक बल का परिकलन उस क्षण पर कीजिए जब x=0.2 m हो | लूप के लिए a=0.1 m लीजिए तथा यह मान लीजिए कि उसका प्रतिरोध बहुत अधिक है |
 07:56View Solution
07:56View Solution(a) चित्रानुसार एक लंबे, सीधे तार तथा एक वर्गाकार लूप जिसकी एक भुजा की लम्बाई a है के लिए अन्योन्य प्रेरकत्व का व्यंजक प्राप्त कीजिए ।
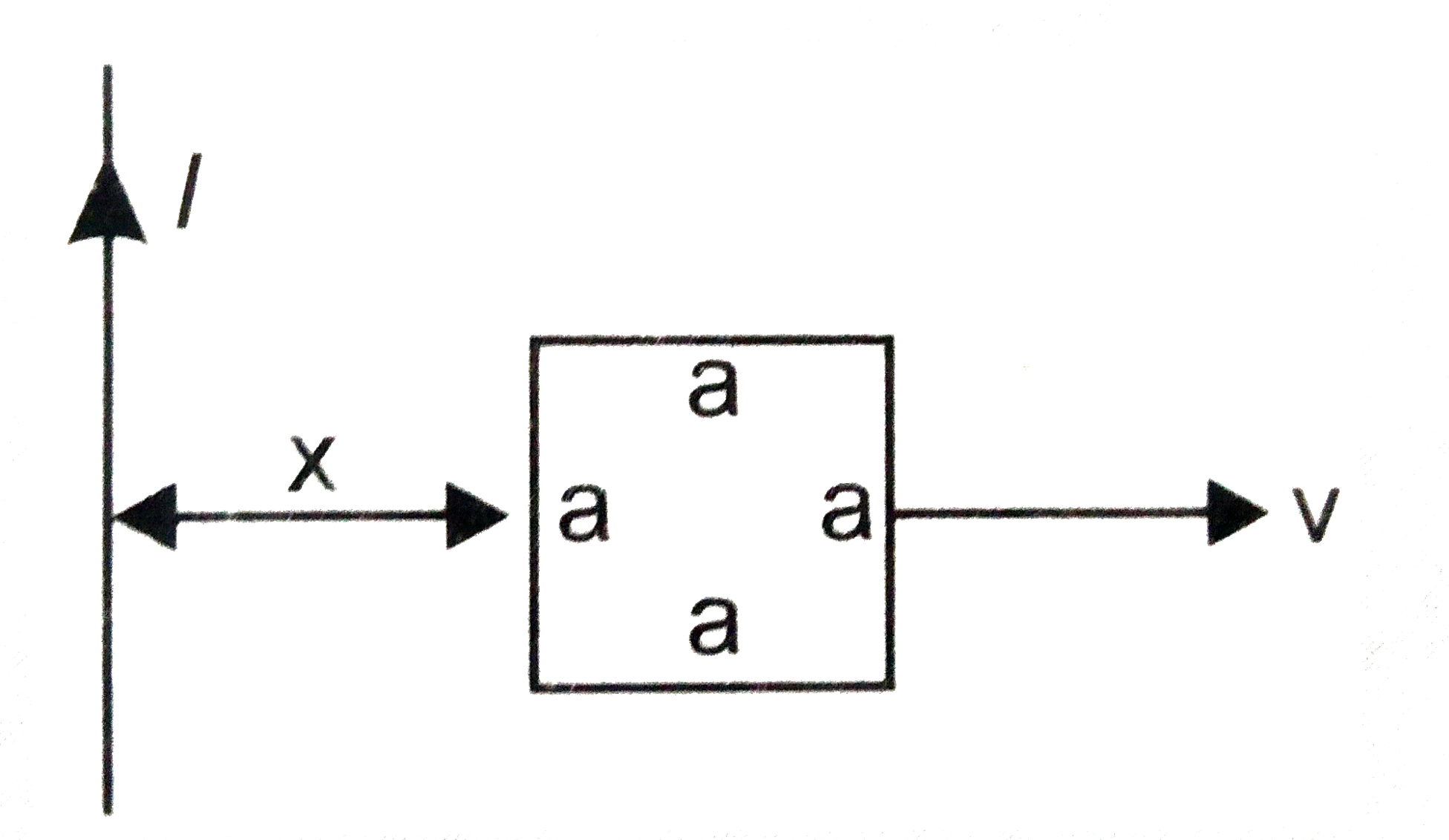
(b) अब मान लीजिए कि सीधे तार में 50 A की धारा प्रवाहित हो रही है तथा लूप एक स्थितर वेग v=10 मीटर/सेकण्ड से दायीं और गति कर रहा है लूप में प्रेरित वि. वा. बल का परिकलन उस क्षण पर कीजिए जब x=0.2 मीटर हो लूप के लिए a=0.1 मीटर लीजिये तथा यह मान लीजिए कि उसका प्रतिरोध बहुत अधिक है05:58View SolutionObtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in the figure.
 06:03View Solution
06:03View SolutionNow assume that the straight wire carries a current of 50A and the loop is moved to the right with a constant velocity, upsilon=10m//s . Calculate the induced emf in the loop at the instant when x=0.2m. Take a=0.1m and assume that the loop has a large resistance.
06:08View Solution(b ) अब मान लीजिये की सीधे तार में 50 A की धारा प्रवाहित हो रही है तथा लूप एक स्थिर वेगv=10 m/s से दायी और को गति कर रहा है । लूप में प्रेरित विद्युत वाहक बल का परिकलन उस क्षण पर कीजिए जब x = 0.2 मी हो । लूप के लिए a = 0.1 मी लीजिये तथा यह मान लीजिए की उसका प्रतिरोध बहुत अधिक है ।
06:08View SolutionObtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in fig.
01:28View SolutionNow assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity v=10m.s−1. Calculate the induced emf in the loop at the instant when x = 0.2 m. Take a = 0.1 m and assume that the loop has a large resistance.
View Solution(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig. (b) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v = 10 m/s. Calculate the induced emf in the loop at the instant when x = 0.2 m. Take a = 0.1 m and assume that the loop has a large resistance.
02:12View Solution
