Derive an expression for the potential energy of an electric dipole placed in a uniform electric field.
The torque τ acting on an electric dipole of magnetic moment p placed in uniform electric field E is given by
τ=pEsinθ . . (i)
Where θ is the angle made by the dipole with the electric field E. the torque tends to align the magnetic dipole along the direction of the field. if the dipole is rotated through a small angle dθ against the torque, work has to be done, which is stored in the form of potential energy of the dipole.
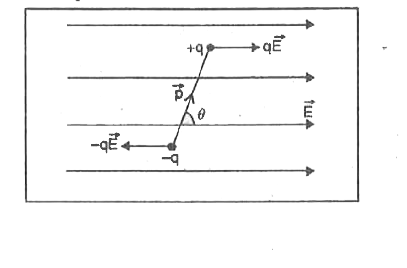
Work done in rotating the dipole through an angle dθ against the torque τ is given by
dW=τdθ=pEsinθdθ
If the dipole is rotated from θ1 to θ2, then
Total work done,
W=∫dW=pE∫θ2θ2sinθdθ
=−pE|(cosθ)|θ2θ1
W=−pE(cosθ2−cosθ1)
this work done is stored as potential energy U.
∴U=−pE(cosθ2−cosθ1)
if the dipole is rotated from θ1=π2 to θ2=θ, then
U=−pE(cosθ−0)=−pEcosθ=−→p.→E.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - LONG ANSWER TYPE QUESTIONS
14 Videos
- ELECTROSTATICSBOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - OBJECTIVE TYPE QUESTIONS
23 Videos
- ELECTROSTATICSBOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - OBJECTIVE TYPE QUESTIONS
23 Videos
- Electromagnetic WavesBOOK - MBD -HARYANA BOARDCHAPTER - Electromagnetic WavesEXERCISE - Example
7 Videos
- MAGNETISMBOOK - MBD -HARYANA BOARDCHAPTER - MAGNETISMEXERCISE - OBJECTIVE TYPE QUESTIONS
17 Videos
Similar Questions
Derive an expression for the torque acting on an electric dipole placed in a uniform electric field.
View SolutionDerive an expression for potential energy of an electric dipole in a uniform electric field. In which situation, the potential energy fo dipole is (i) maximum and (ii) minimum.
View Solution
Knowledge Check
An electric dipole placed in a nonuniform electric field experience
Aa force but not torqueBa torque but no forceCa force as well as a torqueDneither a force nor a torqueAn electric dipole placed in a non-uniform electric field experiences
AOnly force but not torqueBOnly torque but not forceCNo torque and no net forceDBoth torque and net forceAn electric dipole placed in a non uniform electric field may experience
Aonly a forceBonly a torqueCboth force and torqueDneither force nor torque
Self Potential energy and Electric dipole
View SolutionFind the expression of torque acting on an electric dipole placed in a uniform electric field . Using the above obtained expression of torque find the expression for potential energy of electric dipole kept in the uniform electric field . Assume potential energy of electric dipole to be zero when its axis is perpendicular to the externally applied electric field .
View SolutionDerive an expression for the potential energy of an electric dipole of dipole moment →p in an electric field →E.
View Solutiona) Define an ideal electric dipole. Give an example.
b) Derive an expression for the torque experienced by an electric dipole in a uniform electric field. What is net force acting on this dipole.
c) An electric dipole of length 2cm is placed with its axis making an angle of 60∘ with respect to uniform electric field of 105N/C. If it experiences a torque of 8√3 Nm, calculate the (i) magnitude of charge on the dipole, and its potential energy
View SolutionAn electric dipole is placed in a uniform electric field. It may experience
View Solution
