Question

Solve $x{(x+2)}^2{(x-1)}^5(2x-3){(x-3)}^4\ge 0.$

Explanation

Let `E=x(x+2)^(2)(x-1)^(5)(2x-3)(x-3)^(4)`.
Here for x, (x-1), (2x-3), exponents are odd, hence, the sign of E changes while crossing x = 0, 1, 3/2. Also for (x + 2), (x - 3) exponents are even, hence, the sign of E does not change while crossing x = -2 and x = 3.
Further for x gt 3, all factors are positive, hence, the sign of the expression starts with a positive sign from the right hand side.
The sign scheme of the expressioon is as follows:

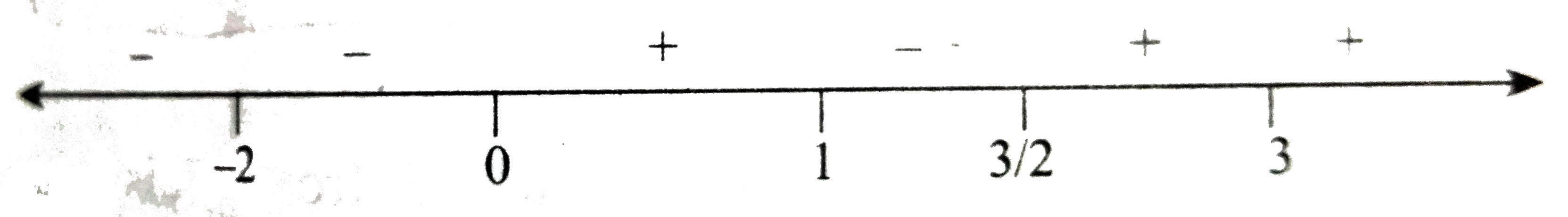
Hence, for `E ge 0, ` we have `x in [0,1] cup [3//2, oo)`