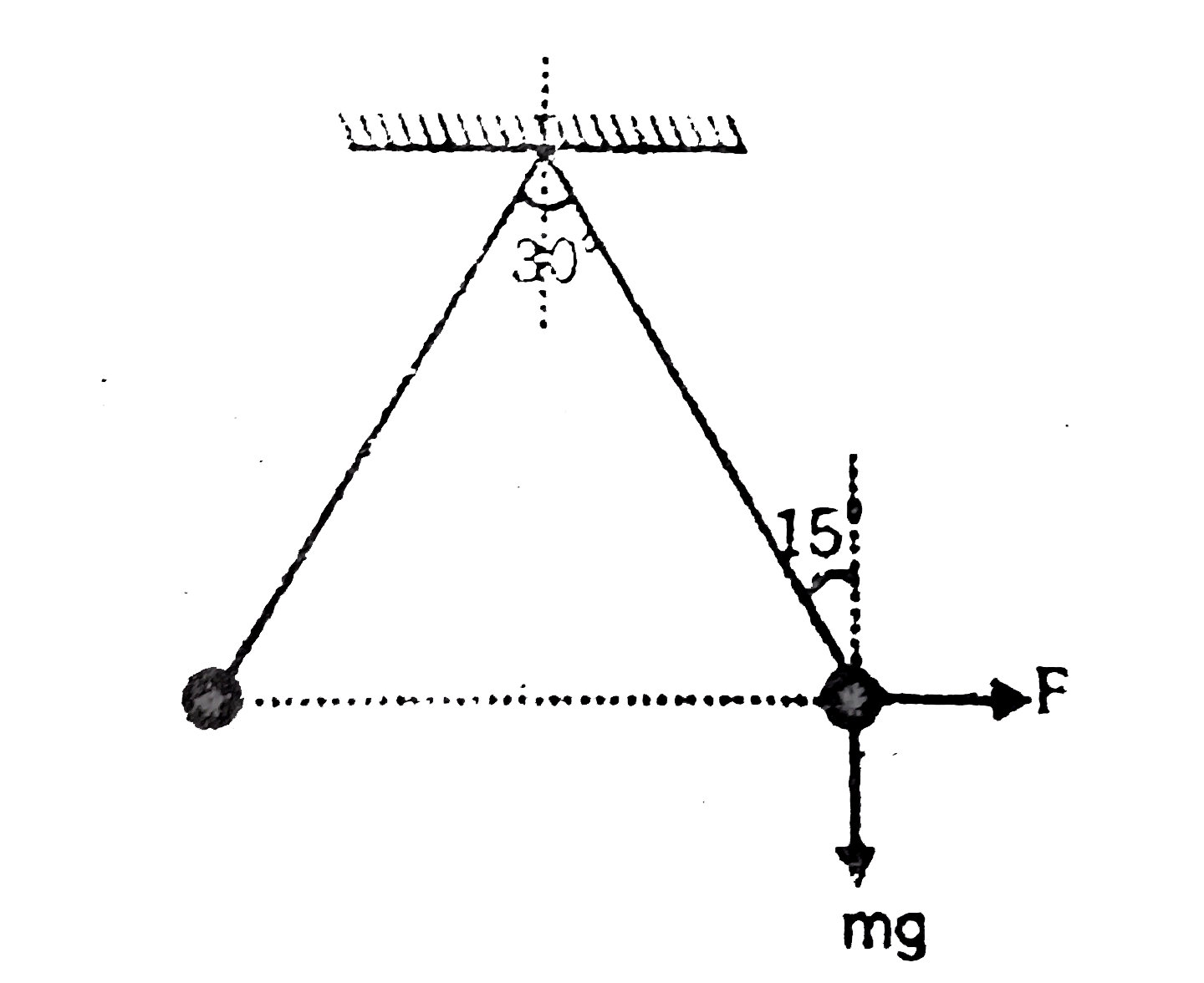
Two identical charged spheres are suspended by strings of equal lengths. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8gcm−3, the angle remains the same. If density of the material of the sphere is 1.6gcm−3, the dielectric constant of the liquid is
1
4
3
2
The correct Answer is:D
Before the spheres are dipped in a liquid , we can write the following equation for equilibrium :
T1cosθ=mg…(i)
T1cosθ=Fel ….(ii)

On dividing the above equations we can write the following :
tanθ=Felmg ....(iii)
When the spheres are dipped into a liquid then due to force of buoyancy effective weight charges to
mg(1−density of liquiddensity of sphere)=mg(1−0.80.6)=mg2
and if dielectric constant of the liquid is K then the force between the charges changes to FelK.
Let the tension changes to T2. Now , we can write the following equations for the equilibrium of the spheres :
T2cosθ=mg/2...(iv)
T2sinθ=FelK...(v)
Dividing the above two equations we get the following :
tanθ=2FelKmg ...(vi)
On equating the results of equation (iii) and (vi) we get K=2
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - COMPETITION FILE ( Objective Question (BC.MCQ))
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - COMPETITION FILE ( Objective Question (C.MCQ))
19 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - COMPETITION FILE ( Objective Question (BA.MCQ))
3 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
Similar Questions
Two identically charged spheres are suspended by strings of equal length. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8g/cc the angle remains same. What is the dielectric constant of liquid. Density of sphere=1.6g/cc
View SolutionTwo identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View Solution
Knowledge Check
Two identical charged spheres of material density ρ, suspended from the same point by inextensible strings of equal length make an angle θ between the string. When suspended in a liquid of density σ the angle θ remains the same. The dielectric constant K of the liquid is
Aρρ−σBρ−σρCρρ+σDρ+σρTwo small identical balls are suspened from a common point by two identical strings. When they are given identical charges, they move apar and the strings make with the vertical an angle of q. Now the system is immersed in a liquid and as a result the angle q does not change. If r is the density of the material of the balls and s is the density of the liquid, the dielectric constant of the liquid is
App−σBp−σpCσp−σDp−σσTwo identical charged spheres are suspended in air from a ceiling using strings of equal length. The point of suspension is common to both the spheres. Neglect the density of air. The density of material of spheres is 2g/cm3.
Both spheres repel each other and the strings are inclined at a certain angle with the vertical when the system is in equilibrium. Now both the sphere are dipped into a liquid of specific gravity 1 and dielectric constant K, but angle that the strings are making with the vertical remains unchanged. Let T1 be the tension in both the strings when the spheres are in air and T2, be the tension in strings when spores are dipped in liquid. Let F1 be the electrostatic force between charges when they were kept in air and F2, be the electrostatic force when spheres are dipped in liquid.
The magnitude of F2/F1 isA3B2C1/2D1/3
Two identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View SolutionA hollow sphere of inner radius 8.0 cm and outer radius 9.0 cm floats half-submerged in a liquid of density 820 kg/m3. (a) What is the mass of the sphere? (b) Calculate the density of the material of which the sphere is made.
View SolutionTwo identical charged spheres are suspended in air from a ceiling using strings of equal length. The point of suspension is common to both the spheres. Neglect the density of air. The density of material of spheres is 2g/cm3.
Both spheres repel each other and the strings are inclined at a certain angle with the vertical when the system is in equilibrium. Now both the sphere are dipped into a liquid of specific gravity 1 and dielectric constant K, but angle that the strings are making with the vertical remains unchanged. Let T1 be the tension in both the strings when the spheres are in air and T2, be the tension in strings when spores are dipped in liquid. Let F1 be the electrostatic force between charges when they were kept in air and F2, be the electrostatic force when spheres are dipped in liquid.
The magnitude of T2/T1 is
View SolutionIn a liquid medium of dielectric constant K and of spherical gravity 2 two identically charged spheres are suspended from a fixed point by threads of equal length .The angle between them is 90∘ .In another medium of unknown dielectric constant K1 and specific gravity 4 the angle between them become 120∘ if density of material of spheres is 8gm/cc thenK1 is
View SolutionIn a liquid medium of dielectric constant K and specific gravity 2 two identically charged spheres are suspended from a fixed point by threads of equal length .The angle between them is 90∘ .In another medium of unknown dielectric constant K1 and specific gravity 4 the angle between them become 120∘ if density of material of spheres is 8gm/cc thenK1 is
View Solution
