If f:R→Randg:R→R are defined by f(x)=x5andg(x)=x4 then check if f, g are one-one and fog is one-one?Solution in Tamil
The correct Answer is:It is an one-one function
Step by step video, text & image solution for If f: R to R and g: R to R are defined by f(x)=x^(5) and g(x)=x^(4) then check if f, g are one-one and fog is one-one? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RELATIONS AND FUNCTIONS BOOK - FULL MARKSCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - EXERCISE 1.6
15 Videos
- RELATIONS AND FUNCTIONS BOOK - FULL MARKSCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - UNIT EXERCISE
10 Videos
- RELATIONS AND FUNCTIONS BOOK - FULL MARKSCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - EXERCISE 1.4
12 Videos
- NUMBERS AND SEQUENCESBOOK - FULL MARKSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - ADDITIONAL QUESTION SOLVED (III. ANSWER THE FOLLOWING).
12 Videos
- SAMPLE PAPER - 10 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER - 10 (UNSOLVED)EXERCISE - PART - IV
2 Videos
Similar Questions
If n(A×B)=6 and A = {1,3} then n (B) .............. .
View SolutionIf the ordered pairs (x2−3x,y2+4y) and (-2,5) are equal , then find x and y .
View SolutionDetermine whether the graph given below represents functions. Give reason for your answers concering each graph
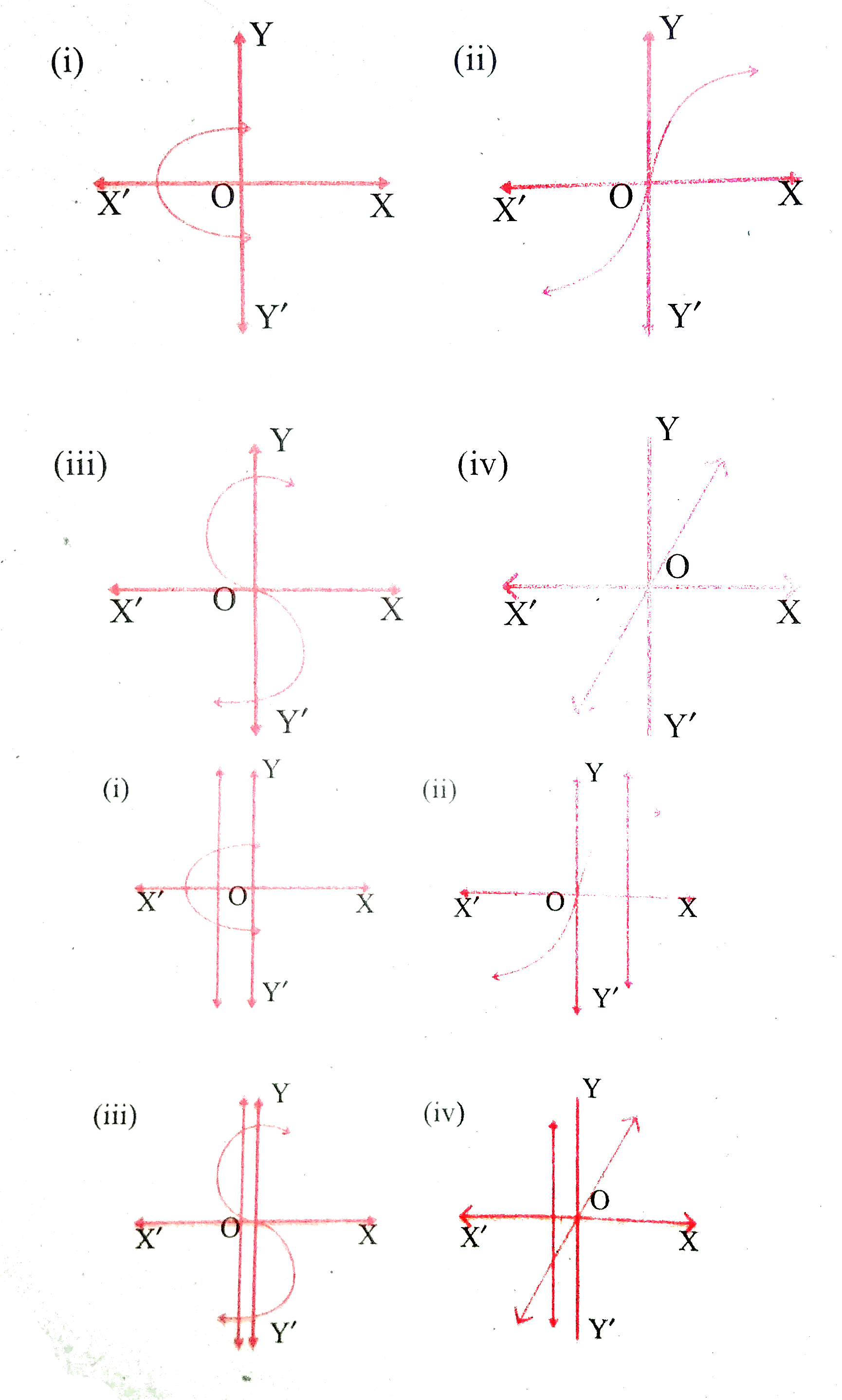
View SolutionUse Euclid's division algorithim to find the HCF of 867 and 255.
View SolutionConstruct a ΔPQR such that QR= 6.5 cm, ∠P=60∘ and the altitude from P to QR is of length 4.5 cm.
View SolutionFind gof and fog when f:R→R and g:R→R is defined by f(x)=x and g(x)=|x|
View SolutionLet f:R→R and g:R→R be defined by f(x)=x2 and g(x)=x+1 . Show that fog≠gof.
View SolutionIf f:R→R and g:R→R be functions defined by f(x)=x2+1 and g(x)=sinx, then find fog and gof .
View SolutionIf f:R→Randg:R→R are defined by f(x)=x5andg(x)=x4 then check if f, g are one-one and fog is one-one?
View SolutionFind fog and g o f when f : R → R and g : R → R is defined by f ( x ) = 5 x + x2 and g ( x ) = 2x
View Solutionयदि फलन f:R→R,f(x)=x2 तथा g:R→R,g(x)=sinx,x∈R से परिभाषित हो , तो (gof ) (x) और (fog)(x) ज्ञात कीजिए तथा सिद्ध कीजिए कि gof≠fog
View SolutionLet f:R→Tandg:R→R be the function defined by f(x)=x1+x2,x∈R,g(x)=x21+x2,x∈R
Then, the correct statement (s) among the following is/are:
(A) both f, g are one-one
(B) Both f, g are onto
(C ) both f, g are not one-one as well as not onto
(D) f and g onto but not one-one
View Solutionयदि f:R→R तथा g:R→R निम्न प्रकार परिभाषित हों कि f(x)=x2 तथा g(x)=√x तो (fog) ज्ञात कीजिए।
View Solutionf:R→R and g:[0,∞)→R is defined by f(x)=x2 and g(x)=√x. Which one of the following is not true?
View SolutionFind gof and fog when f:R→R and g:R→R is defined by f(x)=2x+3 and g(x)=x2+5
View SolutionFind gof and fog when f:R→R and g:R→R is defined by f(x)=2x+x2 and g(x)=x3
View Solution
