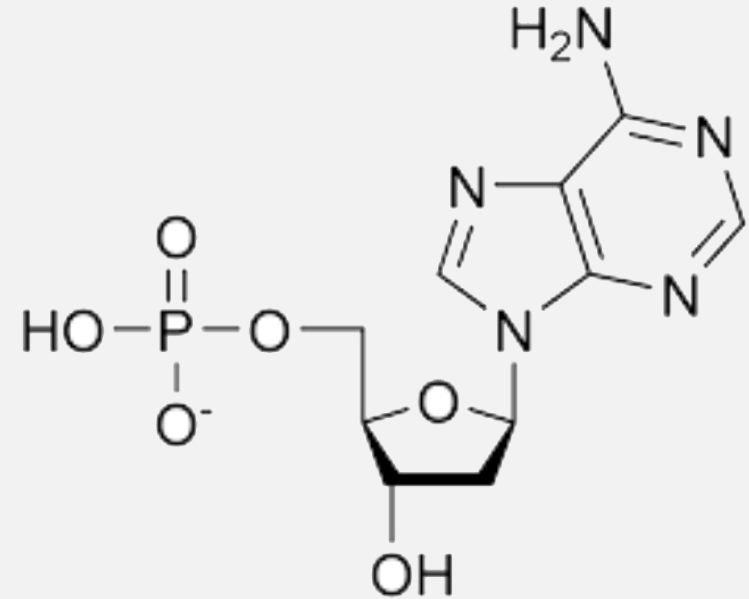
Observe the given population growth formula
Now selecte the correct option.
As population gets larger its rate of growth increases
Represents growth as a continuous process
r is constant and N is variable
all of these
The correct Answer is:D
To solve the question regarding the population growth formula dNdt=rN, we will analyze the components of the equation and the implications of the growth model it represents.
1. Understanding the Formula:
The formula dNdt=rN describes how the population size (N) changes over time (t). Here, dNdt represents the rate of change of the population size, r is the intrinsic rate of natural increase (birth rate minus death rate), and N is the current population size.
Hint: Identify what each component of the formula represents.
2. Exponential Growth:
This formula indicates exponential growth. Exponential growth occurs when resources are unlimited, allowing the population to grow without constraints. The population size increases continuously and does not level off.
Hint: Consider the conditions under which exponential growth occurs.
3. Population Size and Growth Rate:
As the population size (N) increases, the rate of growth (dNdt) also increases because r is constant. This means that the larger the population, the more individuals there are to reproduce, leading to a faster growth rate.
Hint: Think about how population size affects the growth rate in this model.
4. Continuous Process:
The growth described by this formula is continuous, meaning that there are no discrete jumps in population size; it grows smoothly over time.
Hint: Reflect on the nature of growth in biological populations.
5. Identifying the Correct Option:
Based on the analysis:
- The population growth is indeed exponential.
- The growth rate increases as the population size increases.
- The growth is continuous.
- r is constant, while N varies.
Therefore, the correct option would be the one that states: "R is constant and N is variable."
Final Answer:
The correct option is that "R is constant and N is variable."
The formula dNdt=rN describes how the population size (N) changes over time (t). Here, dNdt represents the rate of change of the population size, r is the intrinsic rate of natural increase (birth rate minus death rate), and N is the current population size.
This formula indicates exponential growth. Exponential growth occurs when resources are unlimited, allowing the population to grow without constraints. The population size increases continuously and does not level off.
As the population size (N) increases, the rate of growth (dNdt) also increases because r is constant. This means that the larger the population, the more individuals there are to reproduce, leading to a faster growth rate.
The growth described by this formula is continuous, meaning that there are no discrete jumps in population size; it grows smoothly over time.
Based on the analysis:
- The population growth is indeed exponential.
- The growth rate increases as the population size increases.
- The growth is continuous.
- r is constant, while N varies.
The correct option is that "R is constant and N is variable."
Similar Questions
Knowledge Check
Go through the population growth formula
dNdt=rN
Now select the correct option -AAs population gets larger, its rate of growth increasesBRepresents growth as a continuous processCr is constant and N is variableDAllStudy the population growth curves (A and B) in the given graph and select the incorrect option.
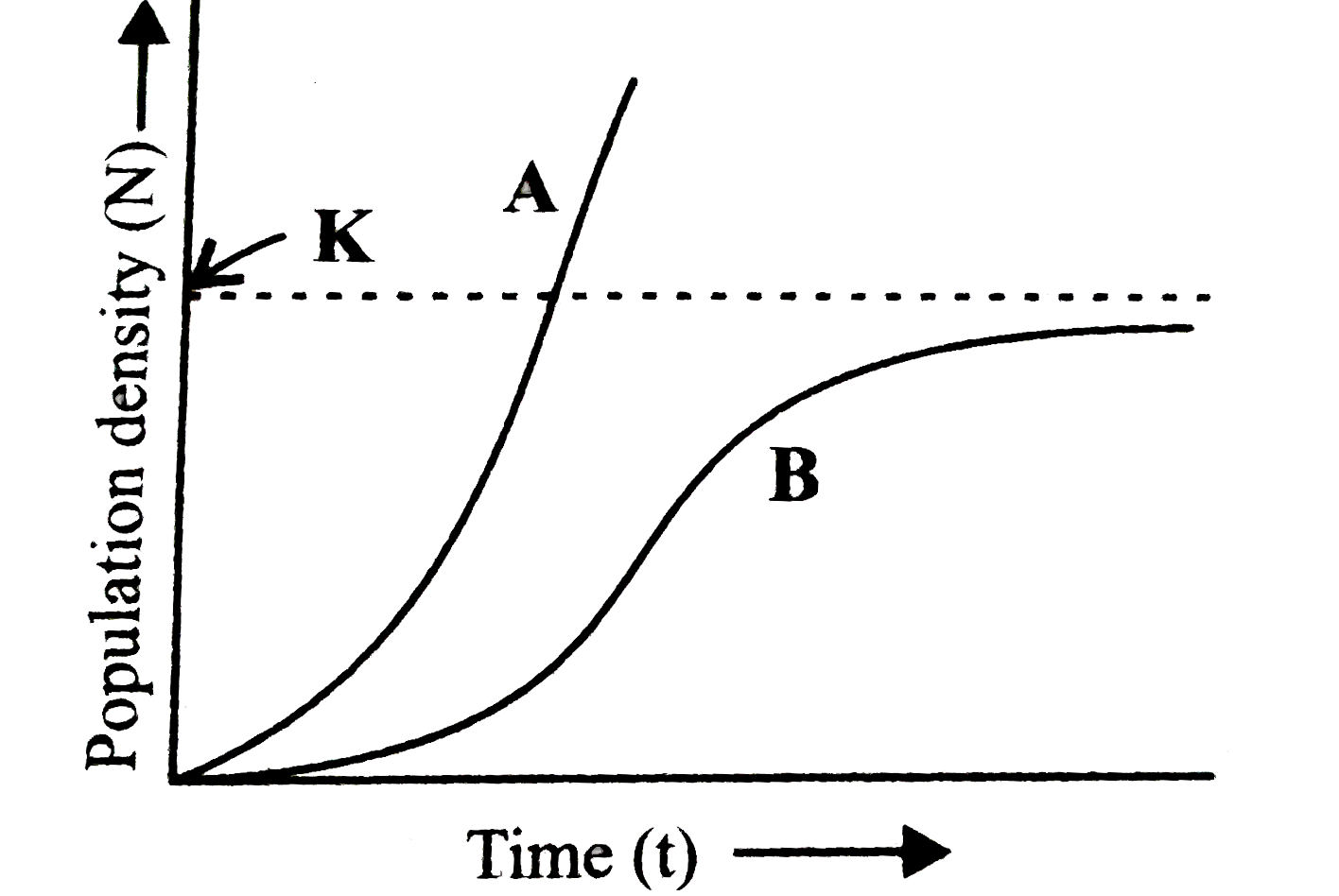 ACurve 'A' shows exponential growth, represented by equation dNdt=rN.BCurve 'B' shows logistic growth, represented by equation dNdt=rN(K−NK)CExponential growth curve is considered as more realistic than the logistic growth curve.DCurve 'A' can also be represented by equation Nt=N0ert.
ACurve 'A' shows exponential growth, represented by equation dNdt=rN.BCurve 'B' shows logistic growth, represented by equation dNdt=rN(K−NK)CExponential growth curve is considered as more realistic than the logistic growth curve.DCurve 'A' can also be represented by equation Nt=N0ert.Identify the given structural formulae and select the correct option.
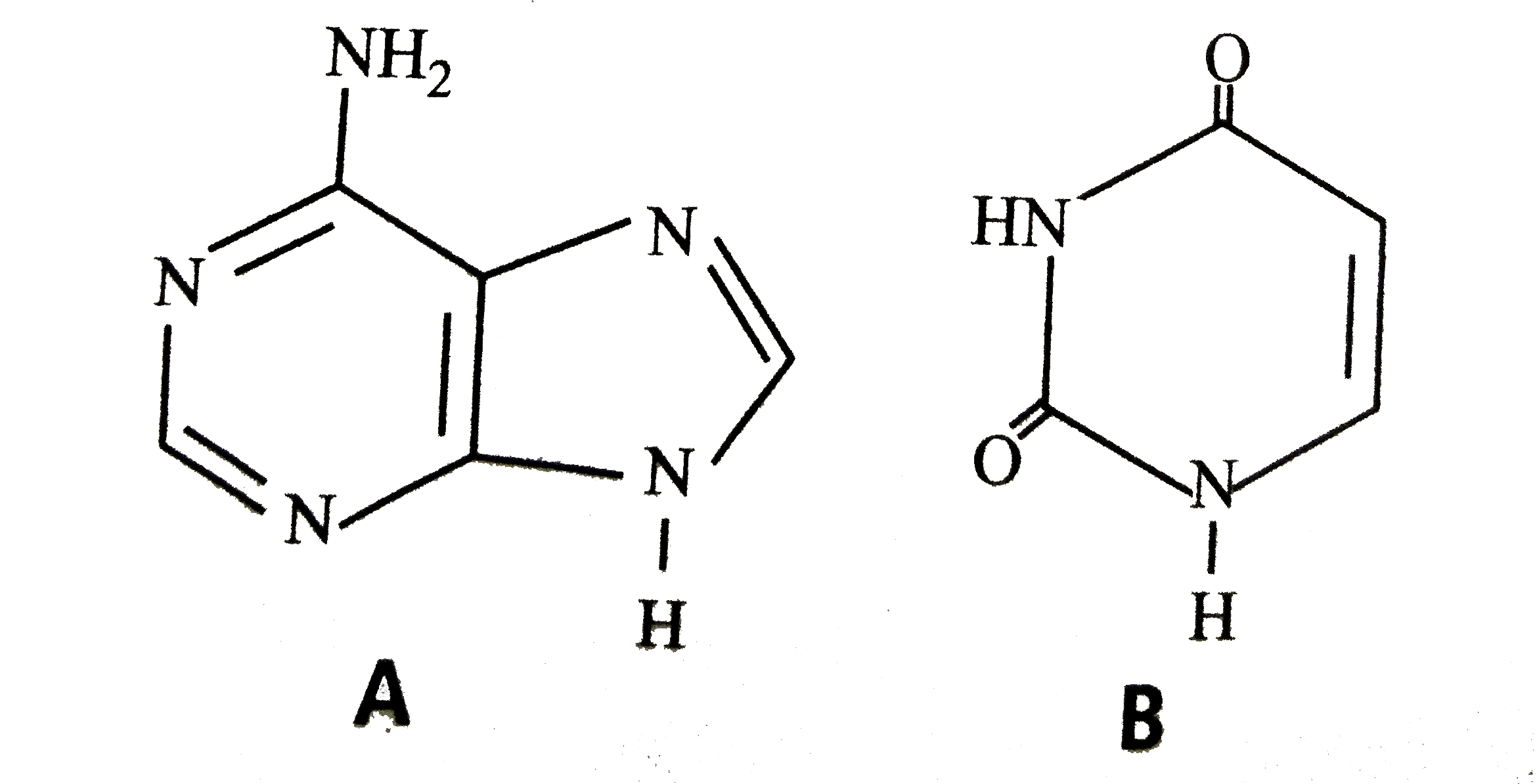 A A BAdenineUracilB A BGuanineThymineC A BAdenineGuanineD A BCytosineThymine
A A BAdenineUracilB A BGuanineThymineC A BAdenineGuanineD A BCytosineThymine
Study the given table and select the correct option :

View SolutionLook at the figure given below and select the correct option.
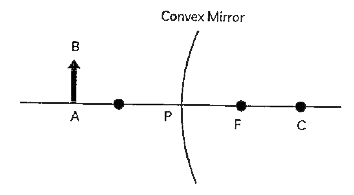
View SolutionThe formula, dN/dt = g represents
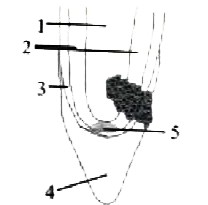
View SolutionGo through the given diagram and select the correct option.
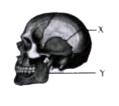
View SolutionRefer to given figure and select the correct option.
View Solution