xA+yB→zC. If −d[A]dt=−d[B]dt=1.5d[C]dt, then x, y and z are :
1,1,1
3,2,3
3,3,2
2,2,3
The correct Answer is:C
−13d[A]dt=−1d[B]3dt=12d[C]dt
Topper's Solved these Questions
- CHEMICAL KINETICS BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EXERCISE -2 (H.W) (ORDER OF REACTION)
16 Videos
- CHEMICAL KINETICS BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EXERCISE -2 (H.W) (HALF LIFE)
13 Videos
- CHEMICAL KINETICS BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EXERCISE -2 (C.W) COLLISION THEORY
2 Videos
- CARBOXYLIC ACIDBOOK - NARAYNACHAPTER - CARBOXYLIC ACIDEXERCISE - All Questions
288 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - NARAYNACHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - ASSERTION & REASON TYPE QUESTIONS
10 Videos
Similar Questions
Rate expression for Xa+Yb→ products is Rate =K[A]m[B]n. Units of K w.r.t A and B respectively are s−1 and M−1s−1, when concentrations of A and B are increased by 4 times, then
View SolutionThe half life for the reaction N2O5⇔2NO2+12O2 in 24hr at 30∘C. Starting with 10g of N2O5 how many grams of N2O5 will remain after a period of 96 hours ?
View SolutionFor A+B→C+D,ΔH=−20kJmole−1. The activation energy for the forward reaction is 85 kJ. Then the activation energy for the backward reaction is ___
View SolutionProvide IUPAC name of the compound given below.
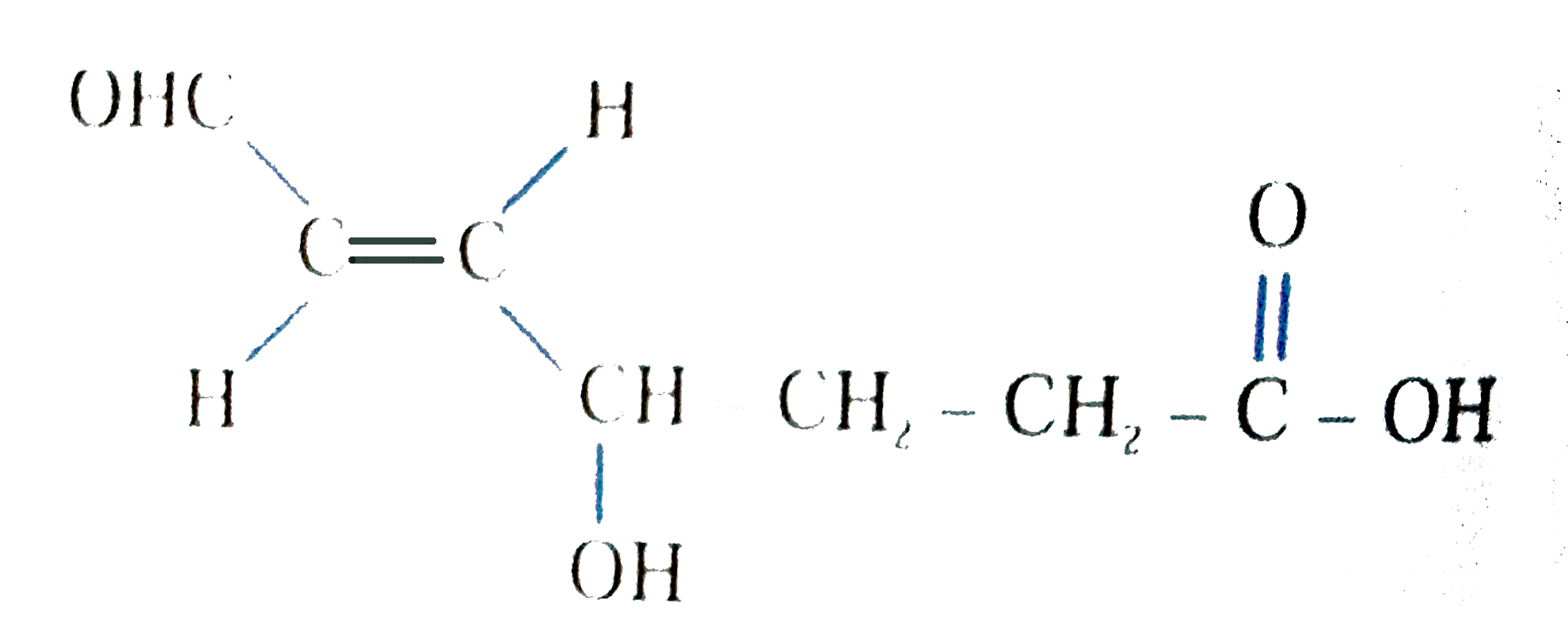
View Solution(A) Analgesics bring down body temperature during high fever.
(R ) Tetracyclin is a tranquillizer.
View SolutionThe second derivative of a single valued function parametrically represented by x=φ(t)andy=ψ(t) (where φ(t)andψ(t) are different function and φ′(t)≠0 ) is given by d2ydx2=⎛⎜ ⎜⎝(dxdt)(d2ydt2)−(d2xdt2)dydt(dxdt)2 d2ydx2=⎛⎜ ⎜⎝(dxdt)(d2ydt2)−(d2xdt2)dydt(dxdt)3 d2ydx2=⎛⎜ ⎜⎝(d2xdt)(dydt2)−(dxdt□)d2ydt2(dxdt)3 d2ydx2=⎛⎜ ⎜⎝(d2xdt2)(dydt□)−(d2xdt2)dydt□(dxdt)3
View SolutionFor the reaction: aA + bB rarr cC+dD Rate = (dx)/(dt) = (-1)/(a)(d[A])/(dt) = (-1)/(b)(d[B])/(dt) = (1)/( c)(d[C])/(dt) = (1)/(d)(d[D])/(dt) In the following reaction, xA rarr yB log.[-(d[A])/(dt)] = log.[(d[B])/(dt)] + 0.3 where negative isgn indicates rate of disappearance of the reactant. Thus, x:y is:
View SolutionFor the reaction aA+bB→cC, if −3d[A]dt=−d[B]dt=+1.5d[C]dt, then a, b and c respectively are
View SolutionxA+yBrarrzC . If -(d[A])/(dt)=-(d[B])/(dt)=1.5(d[C])/(dt) , then x, y and z are :
View SolutionxA + yB to zC. (Here x, y, z are simplest whole number rations) If -(d[A])/(dt) =- (d[B])/(dt) = 1.5 (d[C])/(dt)="then"x+y+z is :
View SolutionIn the reaction; A+2B→3C+D which of the following expressions does not describe changes in the concentration of various species as a function of time?
(A) d[c]dt=−3d[A]dt
(B) 3d[D]dt=d[C]dt
(C) 3d[B]dt=−2d[C]dt
(D) 2d[B]dt=d[A]dt
View SolutionFor a reaction of the type Aa+bB→products
(−)d[A]dt is equal to
(A) −d[B]dt
(B) −bdd[B]dt
(C) −ab×d[B]dt
(D) −ba×d[B]dt
View SolutionFor the reaction,2NO2→2NO+O2 ,rate is expressed as :
(a) −12d[NO2]dt=12d[NO]dt=d[O2]dt
(b) −12d[NO2]dt=−12d[NO]dt=d[O2]dt
(c) −2d[NO2]dt=2d[NO]dt=d[O2]dt
(d) −d[NO2]dt=d[NO]dt=d[O2]dt
View SolutionThe second derivative of a single valued function parametrically represented by x=φ(t)andy=ψ(t) (where φ(t)andψ(t) are different function and φ′(t)≠0 ) is given by d2ydx2=⎛⎜ ⎜⎝(dxdt)(d2ydt2)−(d2xdt2)dydt(dxdt)2 d2ydx2=⎛⎜ ⎜⎝(dxdt)(d2ydt2)−(d2xdt2)dydt(dxdt)3 d2ydx2=⎛⎜ ⎜⎝(d2xdt)(dydt2)−(dxdt□)d2ydt2(dxdt)3 d2ydx2=⎛⎜ ⎜⎝(d2xdt2)(dydt□)−(d2xdt2)dydt□(dxdt)3
View SolutionFor the reaction ,XA+YB→ZC, if
−d[A]dt=−d[B]dt=1.5d[C]dt, then the correct statement among the following is……..
View SolutionFor the reaction: aA + bB rarr cC+dD Rate = (dx)/(dt) = (-1)/(a)(d[A])/(dt) = (-1)/(b)(d[B])/(dt) = (1)/( c)(d[C])/(dt) = (1)/(d)(d[D])/(dt) In the following reaction, xA rarr yB log.[-(d[A])/(dt)] = log.[(d[B])/(dt)] + 0.3 where negative isgn indicates rate of disappearance of the reactant. Thus, x:y is:
View Solution
