When number of particles of masses are at distances ,units from origin on the X-axis, then find the distance of centre of mass of the system from origin.
If then
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 1
9 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 4
11 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
When 'n' number of particles of masses m,2m,3m,….nm are at distances x1=1,x2=2,x3=3…xn=n units respectively from origin on the X-axis, then find the distance of centre of mass of the system from origin.
View SolutionWhen 'n' number of particles of masses m,2m,3m,….nm are at distances x1=1,x2=4,x3=9…xn=n2 units respectively from origin on the X-axis, then find the distance of centre of mass of the system from origin.
View Solution
Knowledge Check
Two points masses of 2 kg and 3 kg lie at 5 m and 9 m away from origin O respectively. Find out the distance of centre of mass of these point masses from the origin.
A14.2 mB7.4 mC20 mD6 mA large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
A=RB≤RC>RD≥RA number of particles each of mass 0.75kg are placed at distances 1m, 2m, 4m, 8m etc. from origin along positive X axis. the intensity of gravitational field at the origin will be -
AGB34GC12GDzero
A large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
View SolutionFor a group of particles of different masses,if all the masses are placed at different points at equal distance R from the origin, then the distance of the centre of mass from the origin will be
View SolutionO is the origin and B is a point on the x-axis at a distance of 2 units from the origin. Match the following lists.
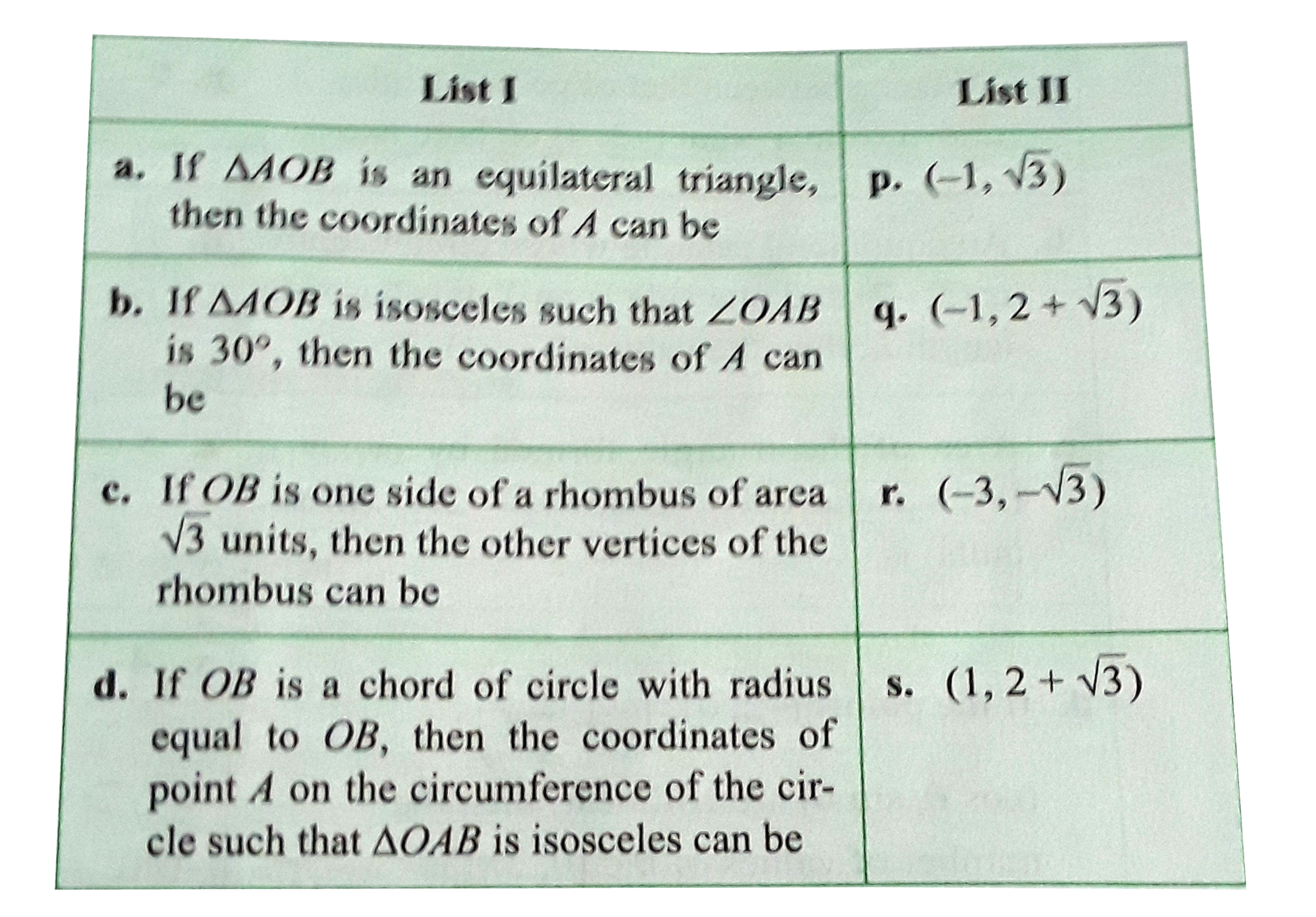
View SolutionAll the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
View SolutionFind the distance of the intercept at point of x-axis and the line 5x+9y−45=0 from origin.
View Solution
