A particle executing SHM along a straight line has zero velocity at point A and B whose distance from 0 on the same line OAB are a and b respectively if the velocity at the mid point between A and B is v then its time period is Solution in Telugu
π(b+a)v
π(b−av)
(b+a2v)
(b−a2v)
The correct Answer is:B
According to the question
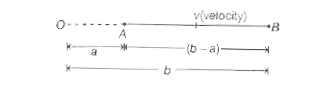
Amplitude of particles executing
Simple harmonic motion (SHM) along a straigh line AB is (a)=b−a2
Velocity of particle v= Amplitude xx Oscillation
∴,v=aε
v=(b−a2)ε or ε=2vb−a
Putting the value of ε from Eq (i ) to above formula
⇒T=2π2v×(b−a)⇒T=b−avπ
So the time period of a particle executing SHM along a straight line from points A to B is
T=b−aVπ
Topper's Solved these Questions
- AP EAMCET ( ONLINE QUESTION PAPER 2018 SOLVED)BOOK - TS EAMCET PREVIOUS YEAR PAPERSCHAPTER - AP EAMCET ( ONLINE QUESTION PAPER 2018 SOLVED)EXERCISE - PHYSICS
40 Videos
- AP EAMCET ENGINEERING ENTRANCE EXAM ONLINE QUESTION PAPER 2019 (SOLVED)BOOK - TS EAMCET PREVIOUS YEAR PAPERSCHAPTER - AP EAMCET ENGINEERING ENTRANCE EXAM ONLINE QUESTION PAPER 2019 (SOLVED)EXERCISE - Physics
40 Videos
Similar Questions
If A represents Boltzmann constant B represents Planck's constant and C represents speed of light in vacuum then the quantity having the dimensions of A4B−3C−2 is
View SolutionN divisions on the main scale of a vernier calipers coincide with (N +1) division of the vernier scale. If each division of main scale is a units, then the least count of the calipers is :
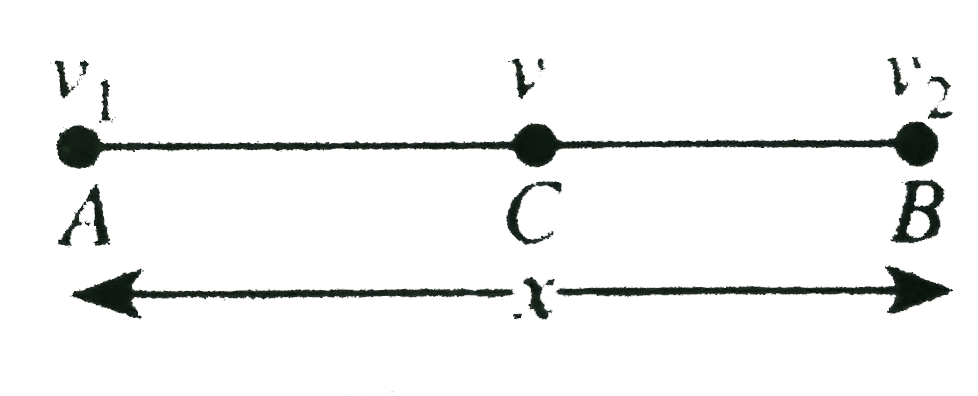
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
 .
.
View SolutionThe time taken by a particle performing SHM on a straight line to pass from point A to B where its velocities are same is 2 seconds .After another 2 seconds it return to B The time period of oscillation is (in seconds)
View SolutionA simple pendulum executing SHM in a straight line has zero velocity at 'A' and 'B' whose distances form 'O' in the same line OAB are 'a' and 'b'. If the velocity half wat between them is 'v' then its time period is
View SolutionA particle executes SHM along a straight line 4cm long. When the displacement is 1cm its velocity and acceleration are numerically equal. The time period of SHM is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x_(1)andx_(2) from the mean position are V_(1)andV_(2) , respectively. Its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distance X_(1) and X_(2) from mean position are V_(1) and V_(2) , respectively. Its time period is :
View SolutionThe time taken by a particle performing SHM on a straight line to pass from point A to B where its velocities are same is 2 seconds. After another 2 seconds it returns to B. The time period of oscillation is in seconds)
View SolutionThe time taken by a particle performing SHM on a straight line to pass from point A to B where its velocities are same is 2 seconds. After another 2 seconds it returns to B. The time period of oscillation is in seconds) ……...
View SolutionA simple pendulum executing S.H.M in a straight line has zero velocity at .a. and .b. whose distances from .o. in the same line OAB are .a. and .b.. If the velocity half -way between them is V then its time period is
View SolutionA simple pendulum excuting S.H.M in a straight line has zero velocity at 'a' and 'b' whose distances from 'o' in the same line OAB are 'a' and 'b'. If the velocity half-way between them is 'v' then its time period is
View SolutionA simple pendulum excuting S.H.M in a straight line has zero velocity at 'a' and 'b' whose distances from 'o' in the same line OAB are 'a' and 'b'. If the velocity half-way between them is 'v' then its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x_(1) and x_(2) from the mean position are v_(1) and v_(2) , respectively. Its time period is
View Solution
