Consider the arrangement shown. The system is released from rest and the string connecting two blocks is burnt, simultaneously. Maximum extension in the spring (initially releaxed) during the subsequent motion is
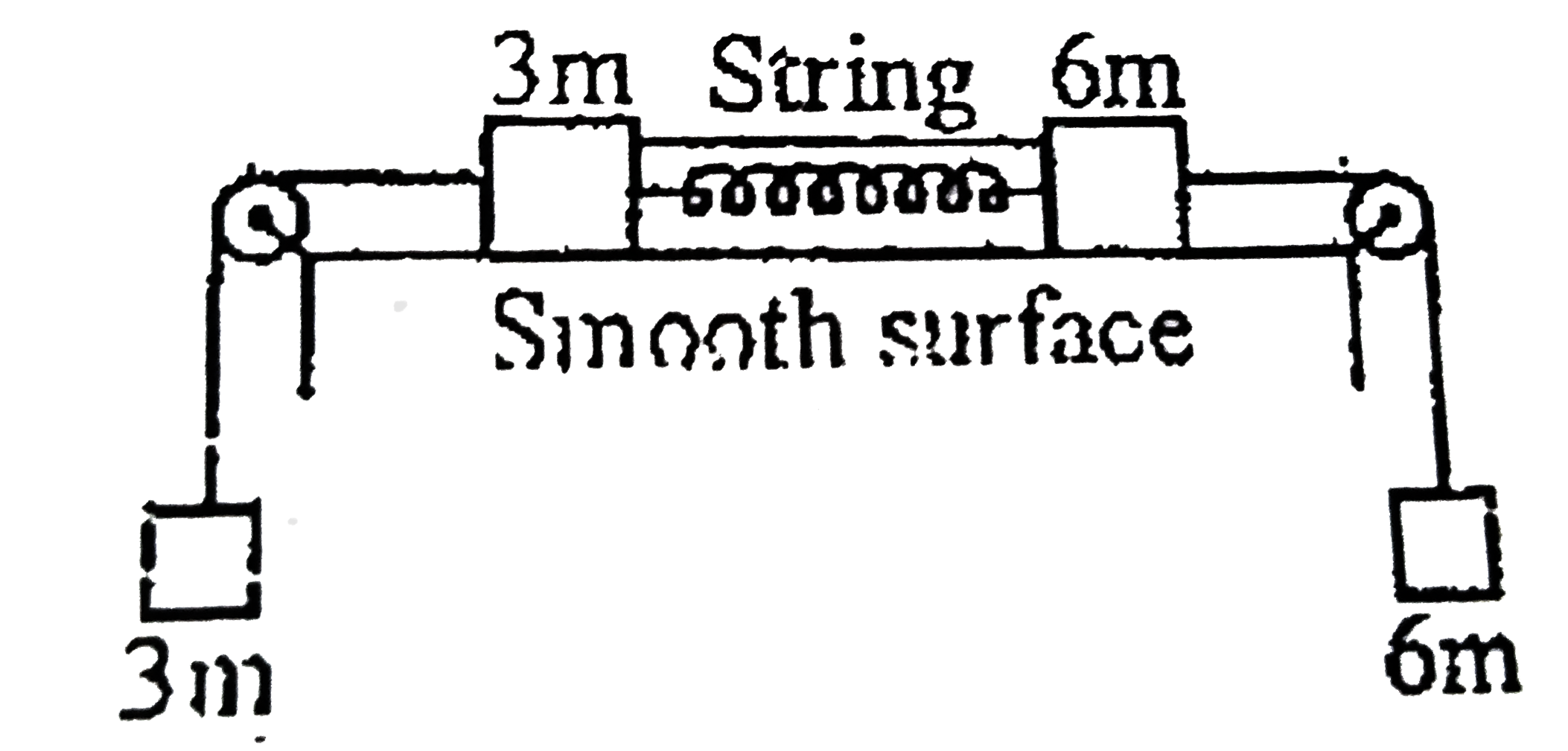
2mgk
4mgk
8mgk
16mgk
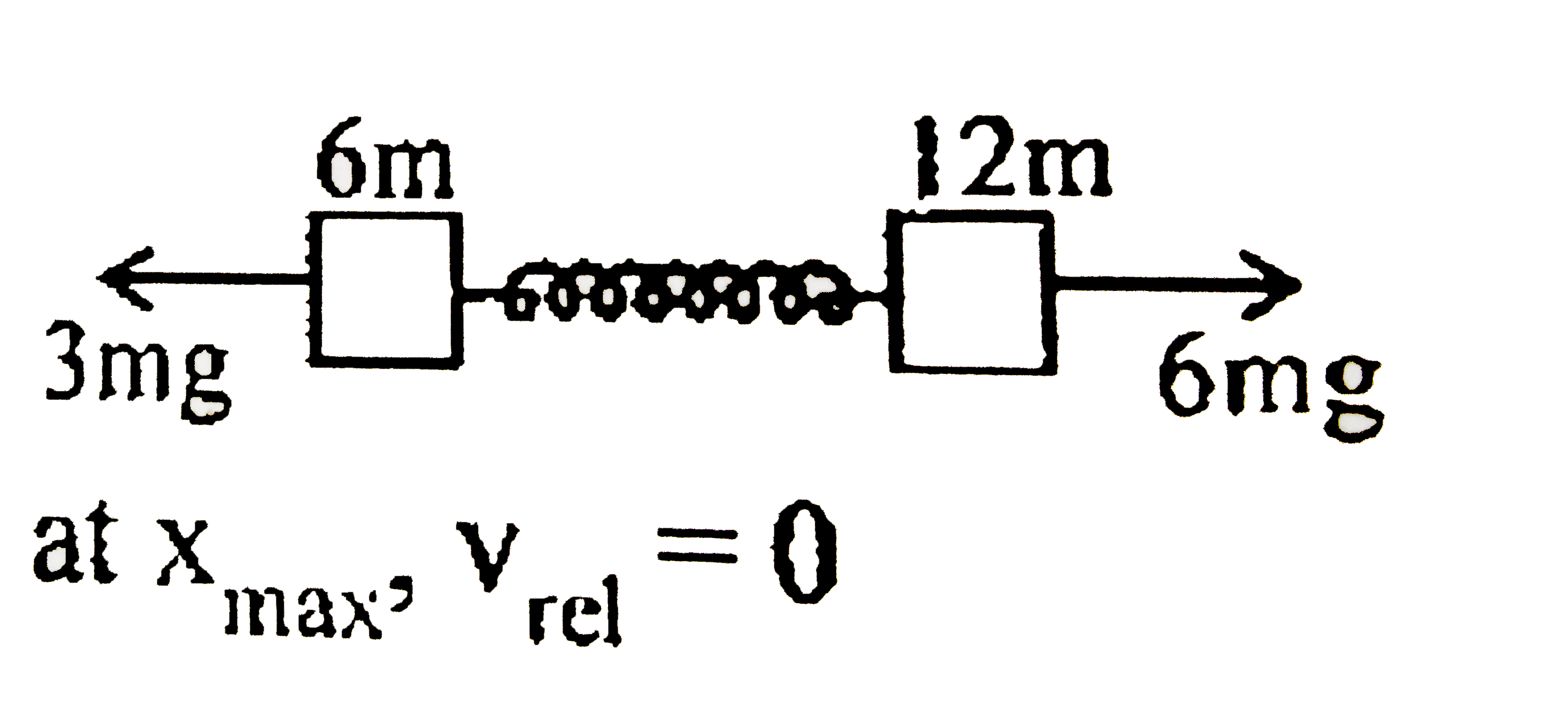
acceleration from cm frame =3mg18m=g6
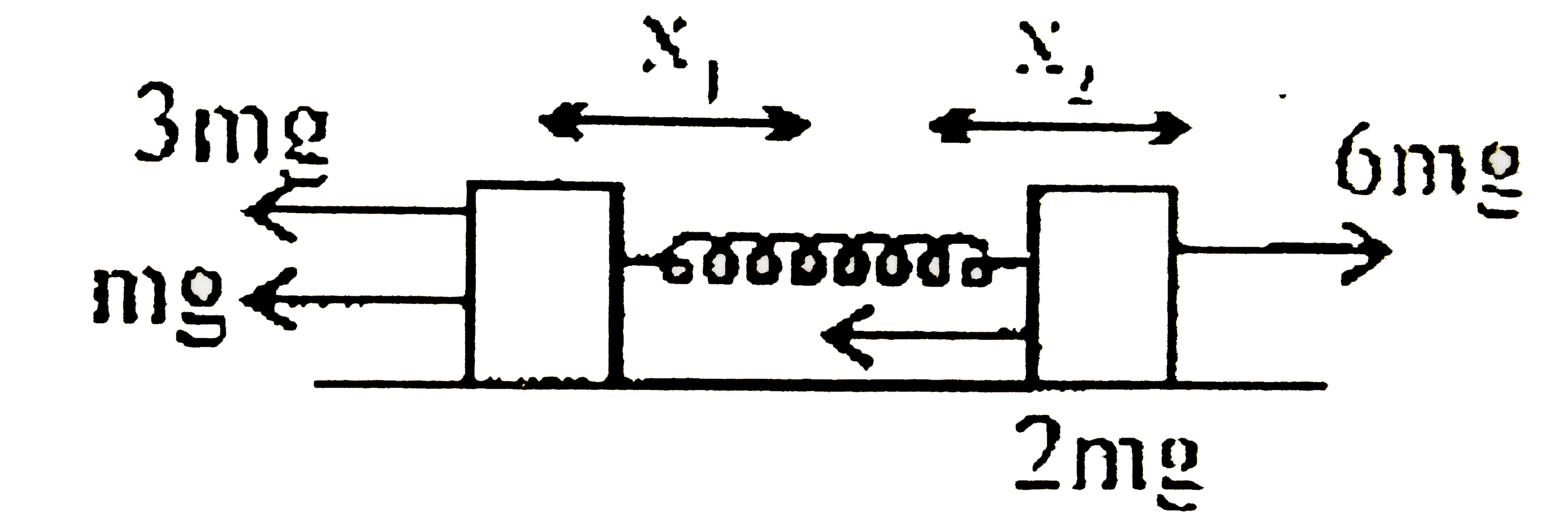
4mgx2+4mgx1−12k(x1+x2)2=0
⇒x1+x2=16mgk
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)32videos
TEST PAPERS
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)3videos
TEST PAPERS
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C4videos
TEST PAPERS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
Consider the system shown in fig. the system os released from rest, find the tension in the cord connected between 1kg and 2 kg blocks.
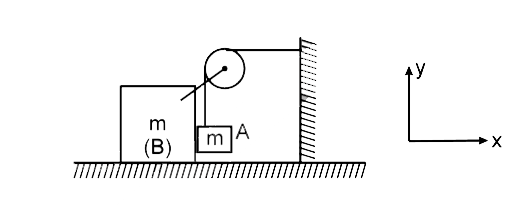
View SolutionBlocks A and B shown in the figure are having equal masses m. The system is released from rest with the spring unstretched. The string between A and ground is cut, when there is maximum extension in the spring. The acceleration of centre of mass of the two blocks at this instant is
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 2M is attached to a massless spring with spring-constant k. This block is connected to two other blocks of masses M and 2M using two massless pulleys and strings. The accelerations of the blocks are a1a2 and a3 as shown in the figure. The system is released from rest with the spring in its unstretched state. The maximum extension of the spring is x0 Which of the following option(s) is(are) correct? [g is the acceleration due to gravity. Neglect friction]
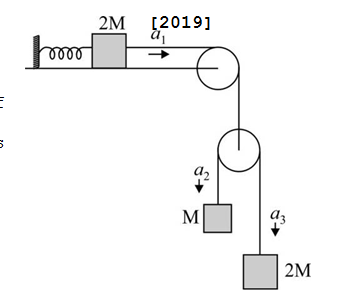Aa2−a1=a1−a2BAt an extension of x04of the spring, the magnitude of acceleration of the block connected to the spring is 3g10Cx0=4MgkDWhen spring achieves an extension of x02 for the first time, the speed of the block connected to the spring is 3g(M)5K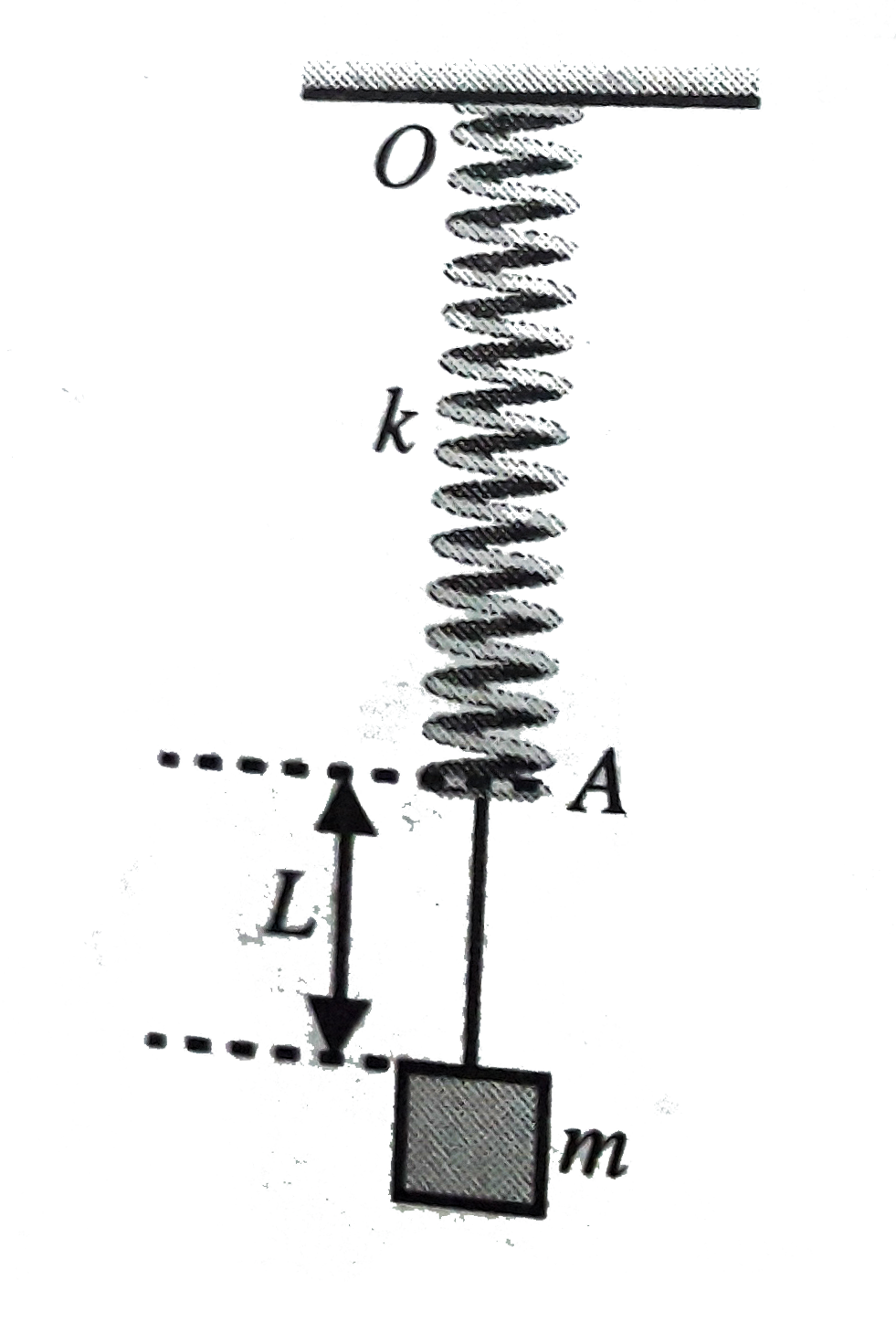 - Question 2 - Select One
A body of mass m is attached by an inelastic string to a suspended spring of spring constant k. Both the string and the spring have negligible mass and the string is inextensible and of length L. Initially, the mass m is at rest.
Q. If the mass m is now raised up to point A (the top end of the string see fig. and allowed to fall from rest, the maximum extension of the spring in the subsequent motion will beALBmgkCmgk√1+2kLmgDmgk[1+√1+2kLmg] - Question 3 - Select One
The given arrangement is released from rest when spring is in natural length. Maximum extension in spring during the motion is x0.a1,a2 and a3 are accelerations of the blocks. Make the correct options
_PHY_E01_005_Q01.png)
Aa2−a1=a1−a3Bx0=4mg3kCVelocity of 2m connected to spring when elongation is x02 is v=x02√3k14mDacceleration a1 at x04 is 3kx042m
In the system shown in figure, the block A is released from rest. Find
Tension in the string.View SolutionThe block is allwed to fall, slowly from the position wher spring is in its natural length. Find, maximum extension in the string.
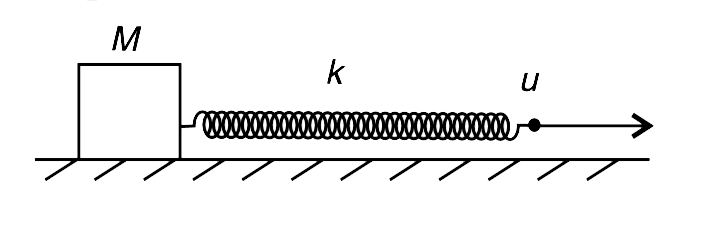
View SolutionA block of mass M is placed on a horizontal smooth table. It is attached to an ideal spring of force constant k as shown. The free end of the spring is pulled at a constant speed u. Find the maximum extension (x0) in the spring during the subsequent motion.
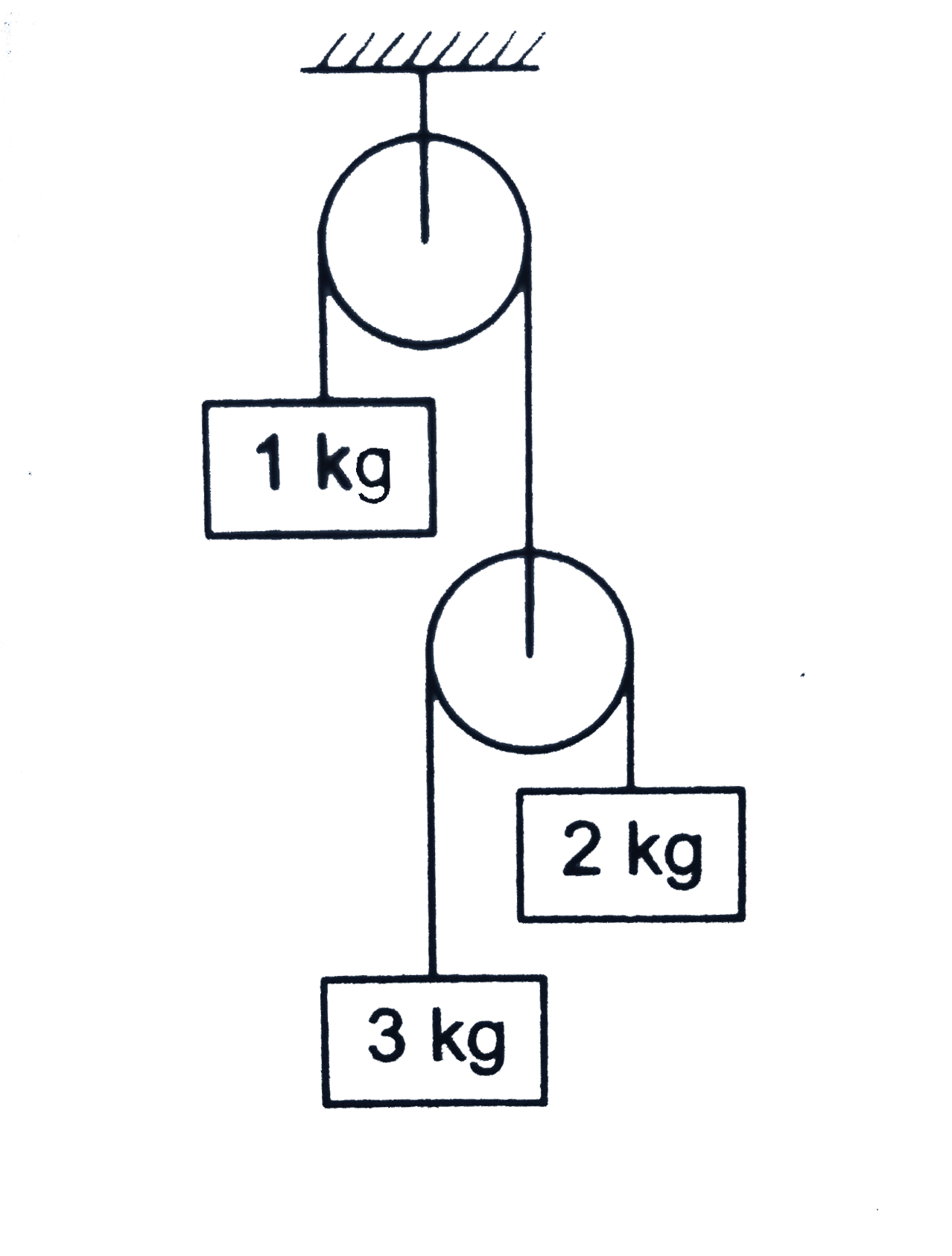
View SolutionThe system shown in figure is released is released from rest . Find acceleration of different string blocks and tenson in different strings.
View SolutionFind the maximum tension in the spring if initially spring at its natural length when block is released from rest.
View Solution