Let ABCD is a square with sides of unit length. Points E and F are taken om sides AB and AD respectively so that AE= AF. Let P be a point inside the square ABCD.The maximum possible area of quadrilateral CDFE is-
1/8
1/4
5/8
3/8
The correct Answer is:C
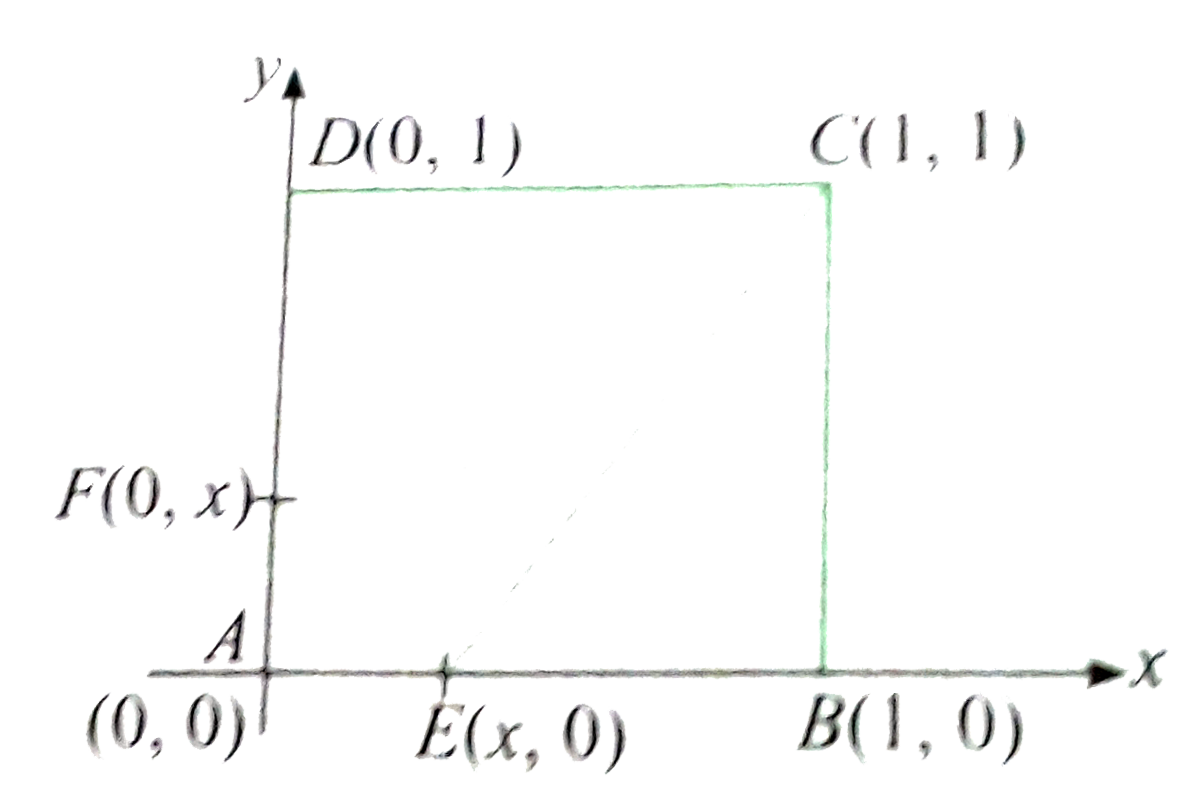
Area of CHEF
A=1−12x2−12(1−x)
=2−2x2−1+x2=1+x−x22=54−(x−12)22
Amax=58atx=12
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Matrix)
4 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Numerical)
12 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Multiple)
13 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Knowledge Check
Let ABCD be a square with sides of unit lenght. Points E and F are taken on sides AB and AD, respectively,so that AE=AF. Let P be a point inside the squre ABCD.
Let a line passing through point A divides the sqaure ABD into two parts so that the area of one portion is double the other. then the length of the protion of line inside the square isA√10/3B√13/3C√11/3D2/√3Let ABCD be a square with sides of unit lenght. Points E and F are taken on sides AB and AD, respectively,so that AE=AF. Let P be a point inside the squre ABCD.
The value of (PA)2−(PB)2+(PC)2−(PD)2 is equal toA3B2C1D0Let □PQRS be a quadrilateral. If M and N are the mid-points of the sides PQ and RS respectively, then PS+QR=
A3¯¯¯¯¯¯¯¯MNB4¯¯¯¯¯¯¯¯MNC2¯¯¯¯¯¯¯¯MND2¯¯¯¯¯¯¯¯NM
ABCD is a square, X and Y are points on sides AD and BC respectively such that AY=BX . Prove that BY=AX and ∠BAY=∠ABX
View SolutionABCD is a square. P, Q, R, S are points on the sides AB. BC, CD, DA respectively such that AP = BQ = CR = DS. What is ∠SPQ equal to ?
View SolutionABCD is a square of side length 1 unit.P and Q are points on AB and BC such that ∠PDQ=45∘ .Then the perimeter of ΔPBQ is
View SolutionLet ABCD be a parallelogram of area 124cm2 If E and F are the mid-points of the sides AB and CD respectively then find the area of the parallelogram AEFD
View SolutionABCD is a square inscribed in a circle of radius 14 cm, E,F,G and H are the midpoints of the sides DA, AB, BC and CD respectively. The area of the square EFGH will be
View Solution
