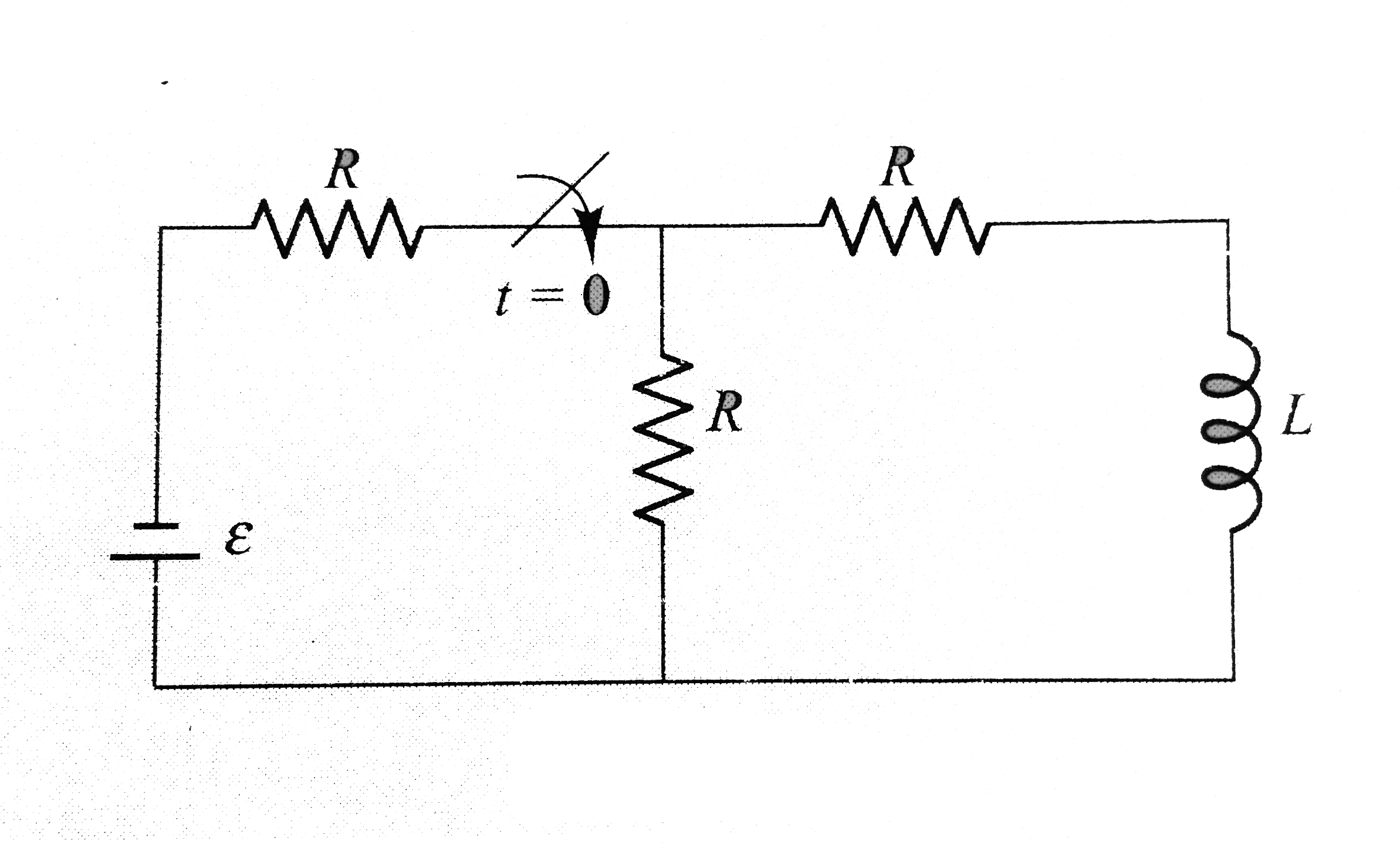In the following circuit the switch is closed at t=0.Intially there is no current in inductor.Find out current the inductor coil as a function of time.
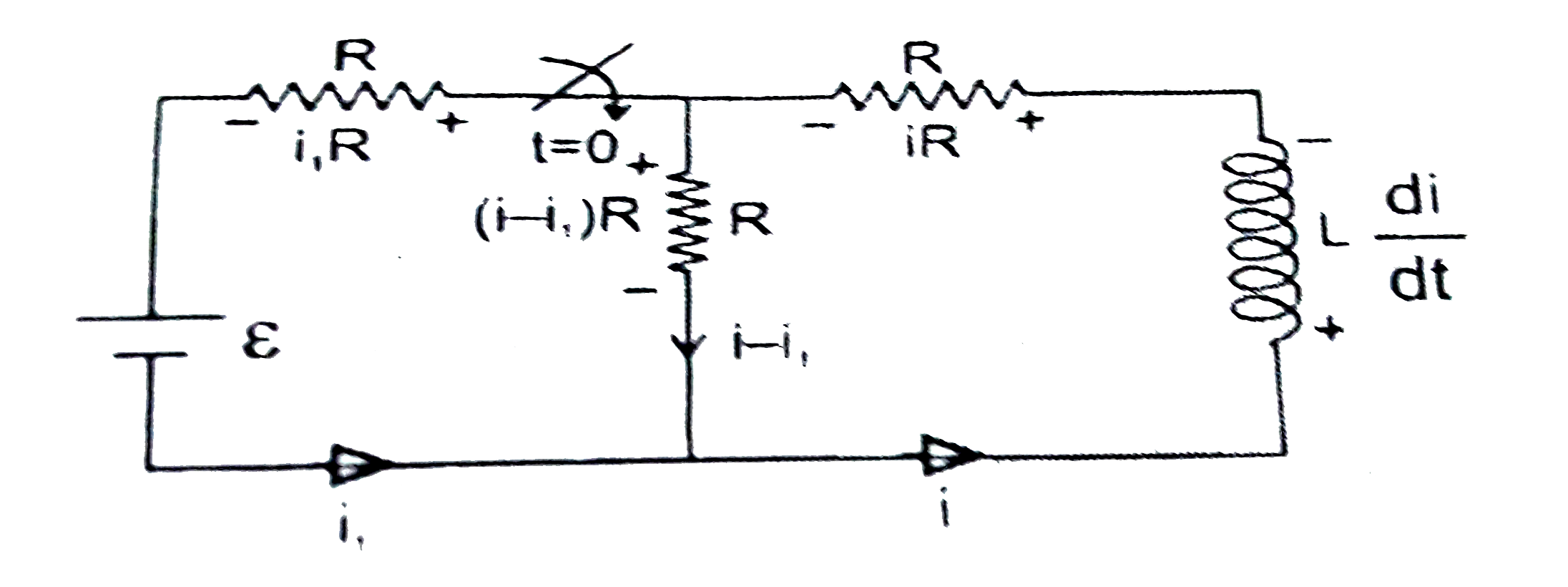
At any time t
−ε+i1R−(i−i1)R=0
−ε+2i1R−iR=0
i1=iR+ε2R Now,−ε+i1R+iR+L.didt=0
=ε+(iR+ε2)+iR+L.didt=0 ⇒=ε2+3iR2=−L.didt
(−ε+3iR2)dt=−L.di ⇒−−dt2L=di−ε+3iR
−∫t0dt2L=∫i0di−ε+3iR ⇒−t2L=13RIn(−ε+3iR−ε)
−In(−ε+3iR−ε)=3Rt2L ⇒i=+ε3R(1−e−3Rt2L)
Similar Questions
Knowledge Check
- Question 1 - Select One
Consider a L-R circuit shown in figure. There is no current in circuit switch S is closed at t = 0, time instant when current in inductor is equal to current in resistor 2R will be :
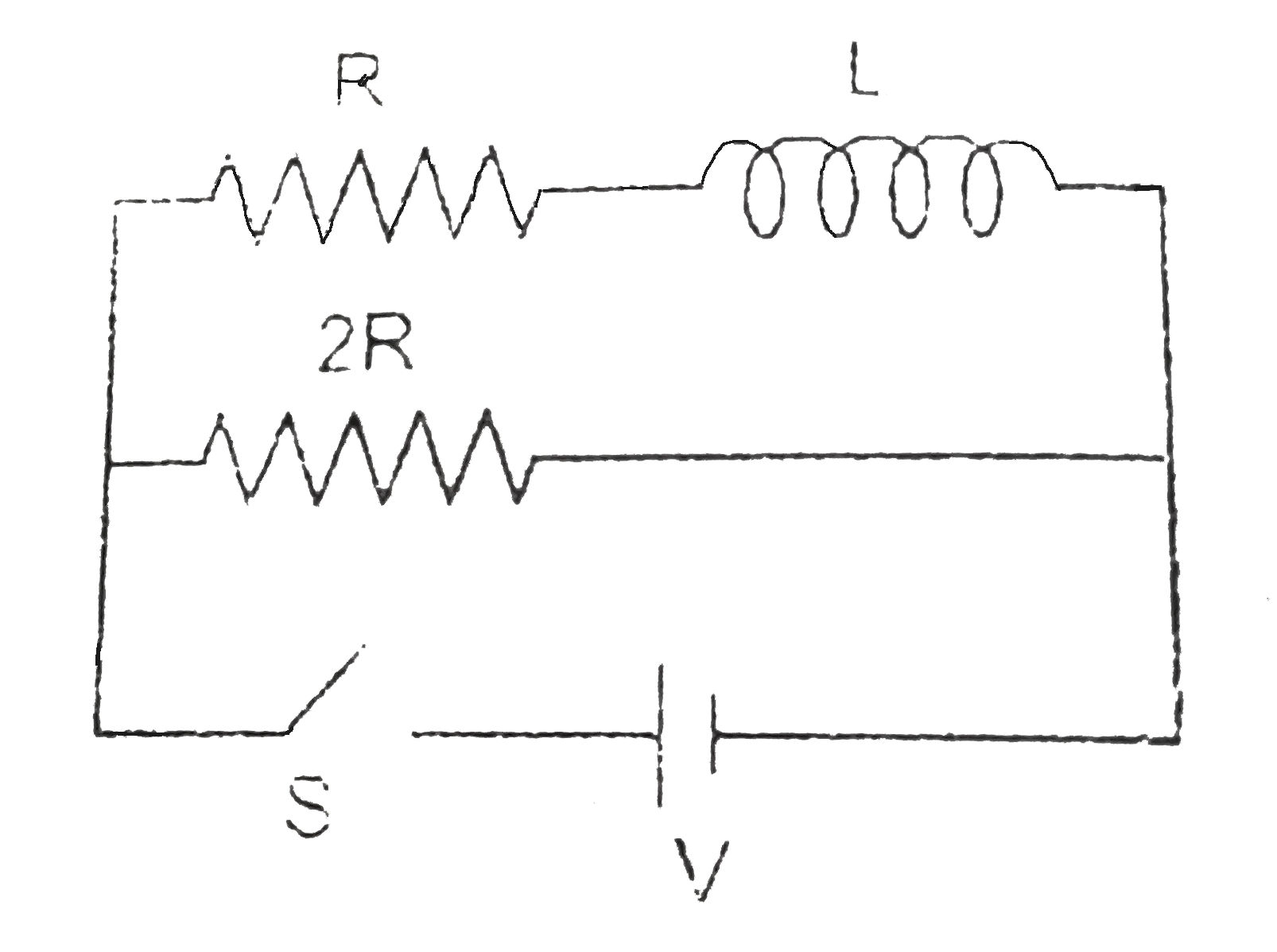 ALRln2B2LRln2CL2Rln2DL2R
ALRln2B2LRln2CL2Rln2DL2R - Question 2 - Select One
In the circuit shown in the following figure, the switch is moved to position 1 at t = 0. Which of the following most closely represents the current I as a function of time?
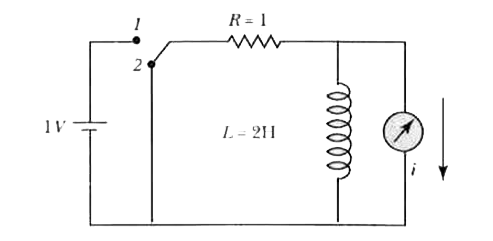 A
A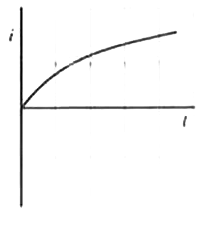 B
B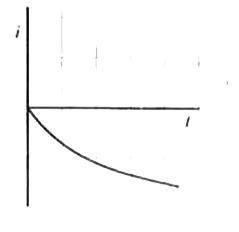 C
C D
D
Decay of current in inductor | Problems on inductor
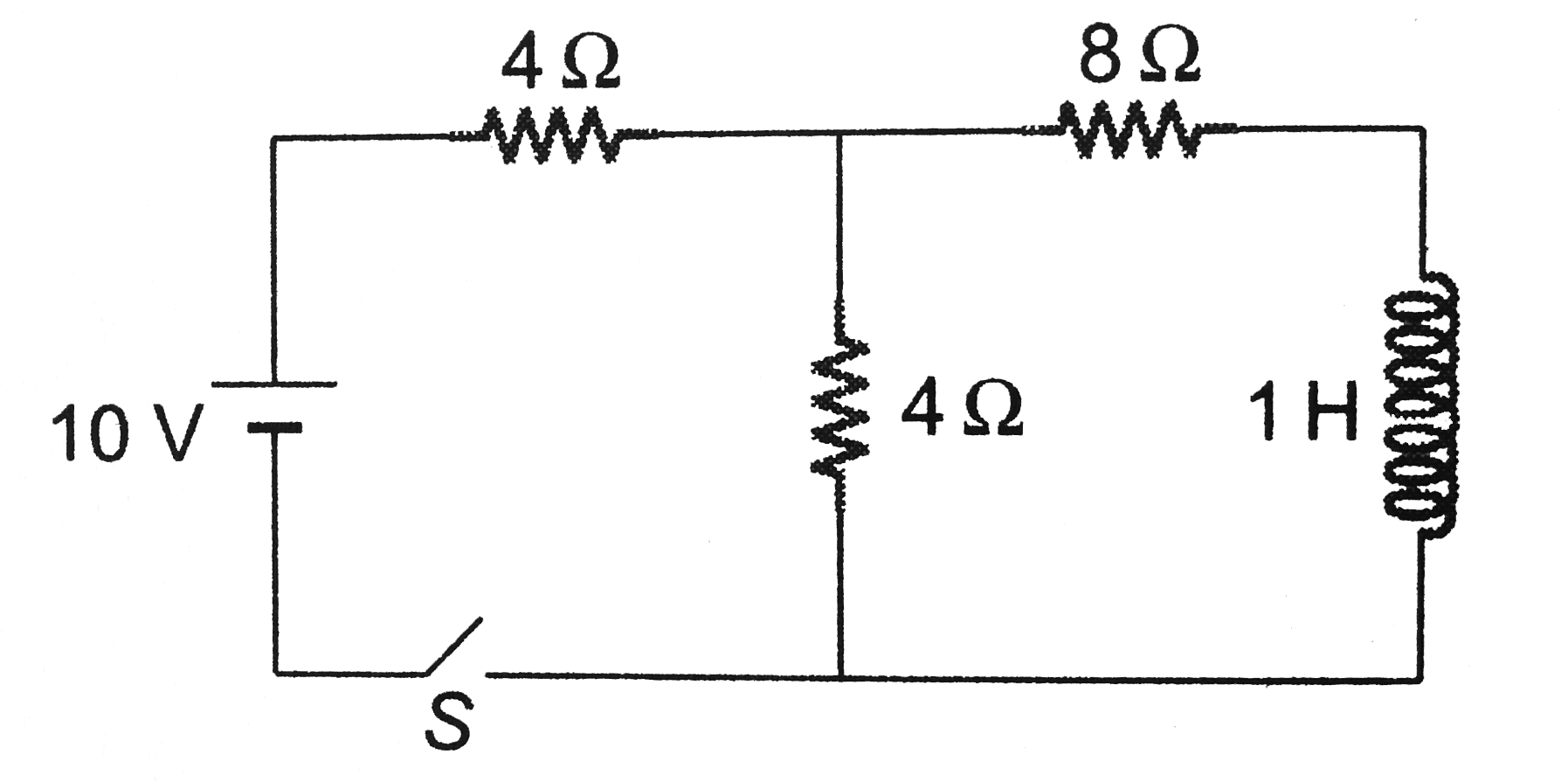
View SolutionThe switch in figure is closed at time t=0. Find the current in the inductor and the current through the switch as functions of time thereafter.
 View Solution
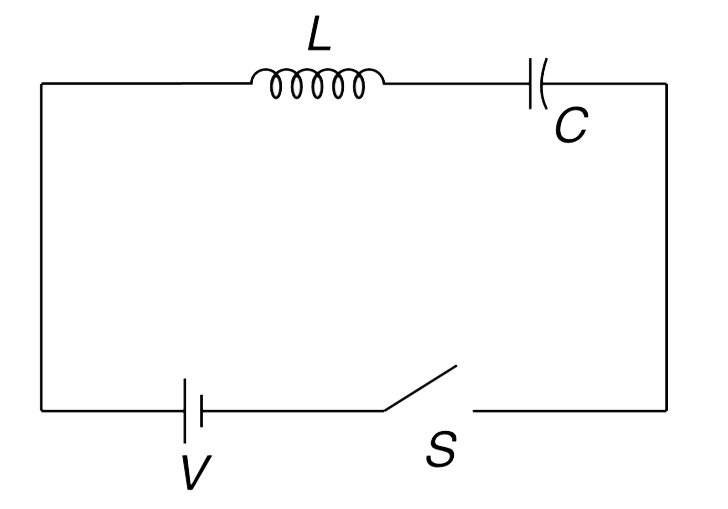
View SolutionIn the circuit shown in the figure, switch S is closed at time t=0.
(a) Write current in the circuit and charge on capacitor as a function of time. Draw the graphical plot for the same.
(b) Find maximum charge on the capacitor. What is potential difference across the inductor when charge on the capacitor is maximum?
 View Solution
View SolutionIn the circuit shown, switch S is closed at time t=0. Find the current through the inductor as a function of time t.
 View Solution
View SolutionIn Fig. 30-44, after switch S is closed at time t = 0, the emf of the source is automatically adjusted to maintain a constant current i through S. (a) Find the current through the inductor as a function of time. (b) At what time is the current through the resistor equal to the current through the inductor?
 View Solution
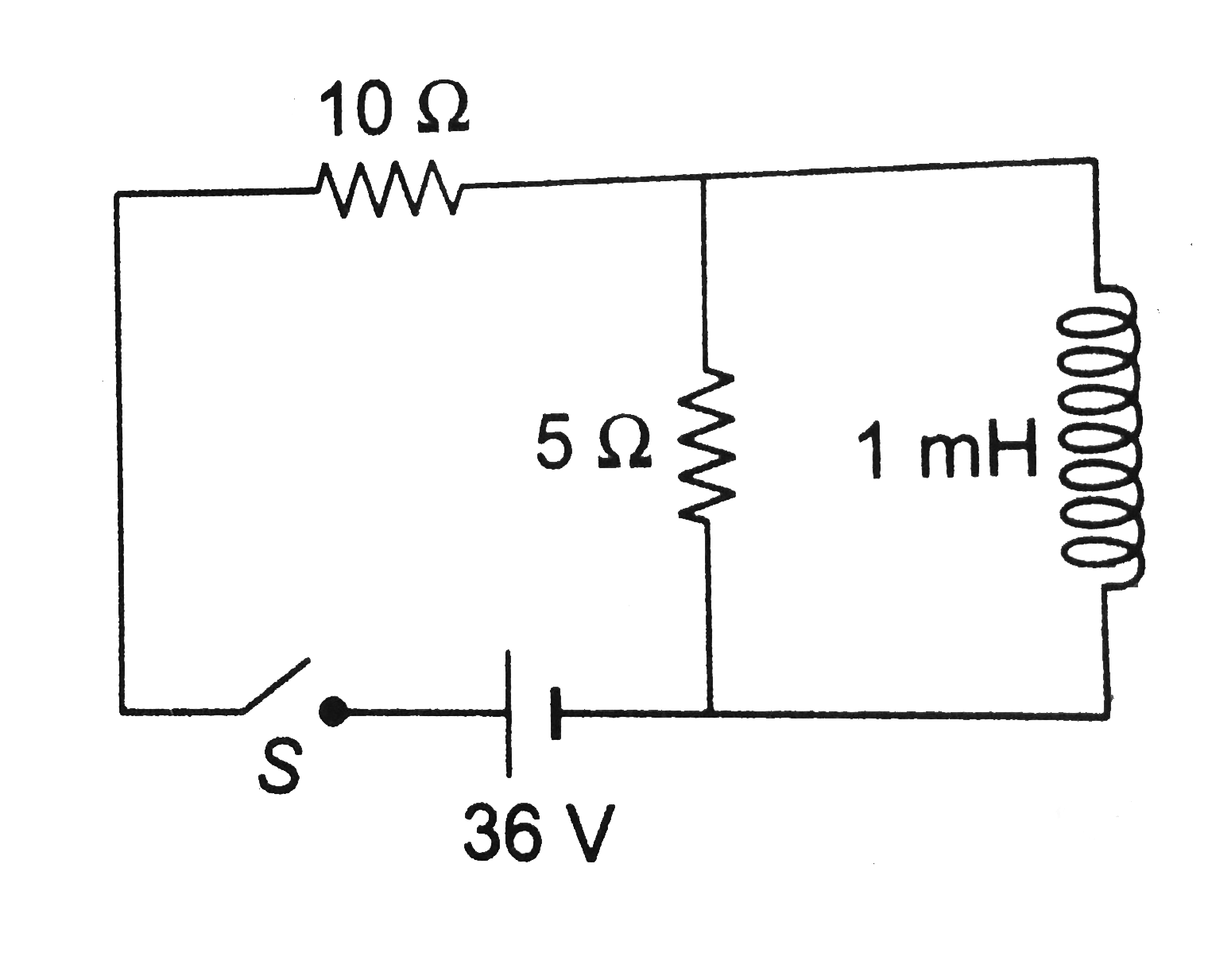
View SolutionIn the circuit arrangement shown in figure, the switch S is closed at t=0. Find the current in the inductance as a function of time? Does the current through 10Ω resistor vary with time or remains constant.
 View Solution
View Solution