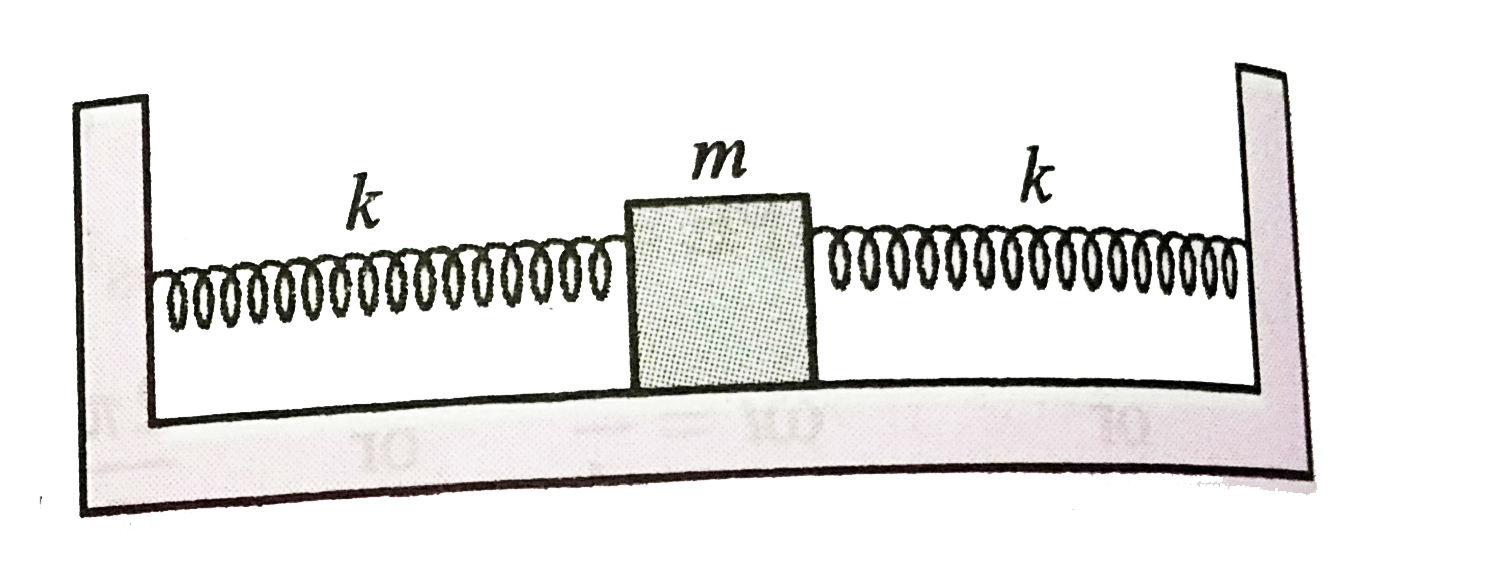
Two springs, each of spring constant k, are attached to a block of mass m as shown in the figure. The block can slide smoothly alongs a horizontal platform clamped to the oppsite walls of the trolley of mass M. If the block is displaced by xcm and released, the period of oscillaion is :

T=2π√Mm2k
T=2π√(M+m)kmM
T=2π√mM2k(M+m)
T=2π(M+m)k
The correct Answer is:C
Step by step video, text & image solution for Two springs, each of spring constant k, are attached to a block of mass m as shown in the figure. The block can slide smoothly alongs a horizontal platform clamped to the oppsite walls of the trolley of mass M. If the block is displaced by x cm and released, the period of oscillaion is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - II
1 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III
12 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II
37 Videos
- SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos
Similar Questions
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View SolutionA block of mass 1 kg is placed inside a car of mass 5 kg , as shown . The block can slide smoothly along horizontal direction. If block is displaced slightly and released , then time period of osciallation is

View Solution
Knowledge Check
Two springs, each of spring constant k = 100N/m are attached to a block of mass 2kg as shown in figure. The block can slide smoothly along horizontal platform clamped to the opposite walls of a trolley of mass 5kg. The block is slightly displace dand then released. The period of oscillation is (in seconds). (all surfaces are smooth)
 AT=2π√71000BT=2π√1140CT=2π√120DT=2π√49100
AT=2π√71000BT=2π√1140CT=2π√120DT=2π√49100Two springs are in a series combination and are attached to a block of mass 'm' which is in equilibrium. The spring constants and extension in the springs are as shown in the figure. Then the force exerted by the spring on the block is :
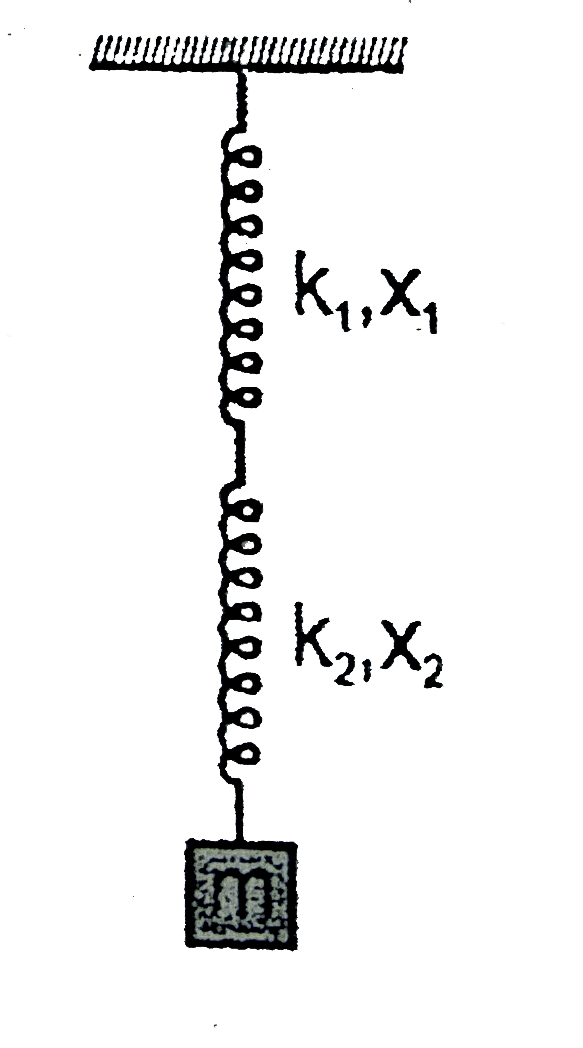 Ak1k2k1+k2(x1+x2)Bk1x1+k2x2Ck1x1DNone of these
Ak1k2k1+k2(x1+x2)Bk1x1+k2x2Ck1x1DNone of theseSpring of spring constant k is attached with a block of mass m1, as shown in figure. Another block of mass m2 is placed angainst m1, and both mass masses lie on smooth incline plane.

Find the compression in the spring when lthe system is in equilibrium.A(m1+m2)gsinθ2kB(m1+m2)gsinθkC(m1+m2)gkD2(m1+m2)gsinθk
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.

View Solution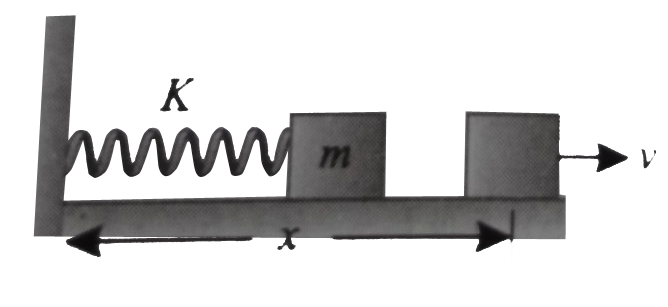
A block of mass m is pushed against a spring of spring constant k fixed at one end to a wall.The block can slide on a frictionless table as shown in the figure. The natural length of the spring is L0 and it is compressed to one fourth of natural length and the block is released.Find its velocity as a function of its distance (x) from the wall and maximum velocity of the block. The block is not attached to the spring.
View SolutionA block of mass m compresses a spring iof stifffness k through a distacne l/2 as shown in the figure .If the block is not fixed to the spring the period of motion of the block is

View SolutionA block is placed on a frictionless horizontal table. The mass of the block is m and springs are attached on either side with force constants K1andK2 . If the block is displaced a little and left to oscillate, then the angular frequency of oscillation will be
View Solution
