In an LR circuit, current at t=0 is 20A. After 2s it reduces to 18A. The time constant of the circuit is :
In(109)
2
2In(109)
2In(109)
The correct Answer is:C
Step by step video, text & image solution for In an LR circuit, current at t = 0 is 20 A. After 2 s it reduces to 18 A. The time constant of the circuit is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 1
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 2
17 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1
63 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
Knowledge Check
In an LR circuit, current at t=0 is 2A . After 2s it reduced to 18A. The time constant of the circuit is (in second)
AIn(109)B2C2In(109)D2In(109)Time constant of LR circuit will be
 ALRB2LRCL2RDNone of these
ALRB2LRCL2RDNone of theseThe current in a LR circuit builds up to 34th of its steady state value ion 4s. The time constant of this circuit is
A1in2sB2in2sC3in2sD4In2s
The current in a LR circuit builds up to 34th of its steady state value in 4s. The time constant of this circuit is
View SolutionThe current in a discharging LR circuit without the battery drops from 2.0 A to 0.10 s. (a) find the time constant of the circuit. (b) if the inductance of the circuit is 4.0 H, what is its resistance?
View SolutionThe current in a LR circuit builds up to 34th of its steady state value in 4s . The time constant of this circuit is
View SolutionConsider the circuit shown below. When switch S1 is closed, let I be the current at time t, then applying Kirchhoff's law
E−iR−Ldidt=0or∫i0diE−iR=1L∫10dt
i=ER(1−e−Rl⋅t)
LR = time constant of circuit
When current reaches its steady value (=i0, open S1 and close S2, the current does reach to zero finally but decays expnentially. The decay equation is given as i=i0e−RL⋅T).
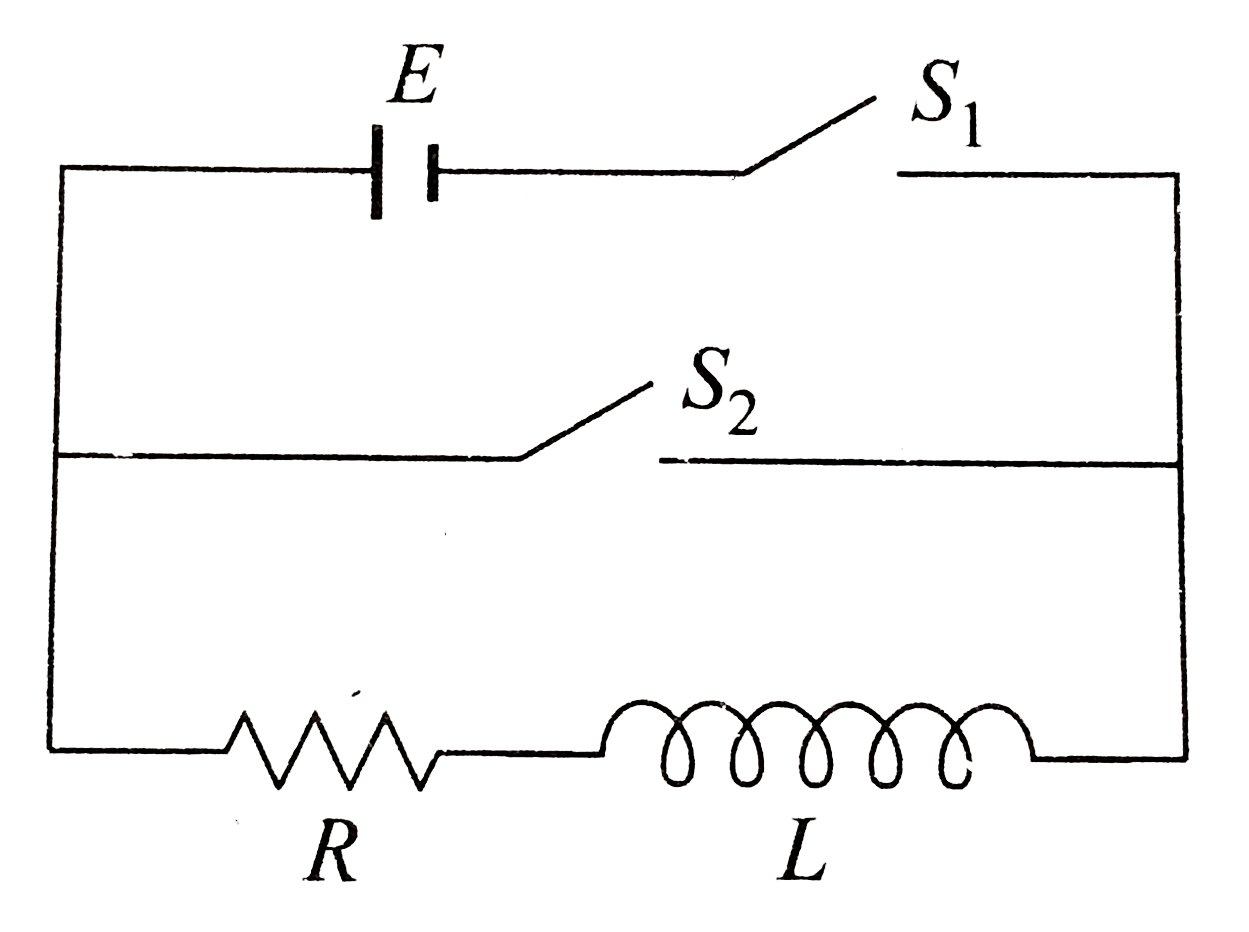
When a coil carrying a steady current is short circuited, the current decreases in it η times in time t0. The time constant of the circuit is
View SolutionA coil carrying a steady current is short-circuited. The current in it decreases α times in time t0. The time constant of the circuit is
View Solution