Two insulated wires are wound on the same hollow cylinder so as to form two solenoids sharing a common air-filled core. Let l be the length of the core, A the cross -sectional area of the core, N1 the number of times the first wire is wound around the core, and N2 the number of times the second wire is wound around the core. Find the mutual inductance of the two solenoids, neglecting the end effects.
If a current l1 flows around the first wire then a unifrom axial magnetic field of strength B1=μ0N1l1l is generated in the core.The magnetic field in the region outside the core is of negligible magnitude.The flux linking a single turn of the second wire is B1A.Thus, the flux linking all N2 turns of the second wire is ϕ2=N2B1A=μ0N1N2AI1l=MI1
∴M=μ0N1N2Al
As described prevoiusly, M is a geometric quantity depending on the dimensions of the core and the manner in which the two wires are wound around the core, but not on the actual currents flowing through the wires
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problem
12 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1
63 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
Two insulated wires are wound on the same hollow cylinder, s as to from two solenoids shering a common air-filled core. Let l be the length of the core, A the cross -sectional area of the core, N1 the number of times the first wire is wound around the core, and N2 the number of times the second wire is wound around the core. Find the mutual inductance of the two solenoids, neglecting the end effects.
View SolutionNumber of base pairs present in DNA wrapped around a core of histone proteins
View Solution
Knowledge Check
Number of base pairs present in DNA wrapped around a core of histone proteins
A146B154C200D54A current of 10 A flows, through a toroid having a core of means radius 25 cm. If 5000 turns of the conducting wire are wound on the core, then the magnetic field the core of thre toroid is
A2×10−2Wb/m2B3×1−−2Wb/m2C4×10−2Wb/m2D6×10−2Wb/m2Two wires are made of the same material and have the same volume. However wire 1 has cross-sectional area A and wire 2 has cross-sectional area 3A. If the length of wire 1 increases by Δx on applying force F, how much force is needed to stretch wire 2 by the same amount?
A4FB6FC9FDF
The length of a thread is 10-hand spans. It is used to measure the circumference of a solid cylinder. For this purpose, the thread is wound around the circumference of the cylinder.
If the thread is wound around the cylinder five times, then what is the circumference of the cylinder?
View SolutionA coil is wound on an iron core and looped back on itself so that the core has two sets of in opposite senses. How is its self inductance affected ?
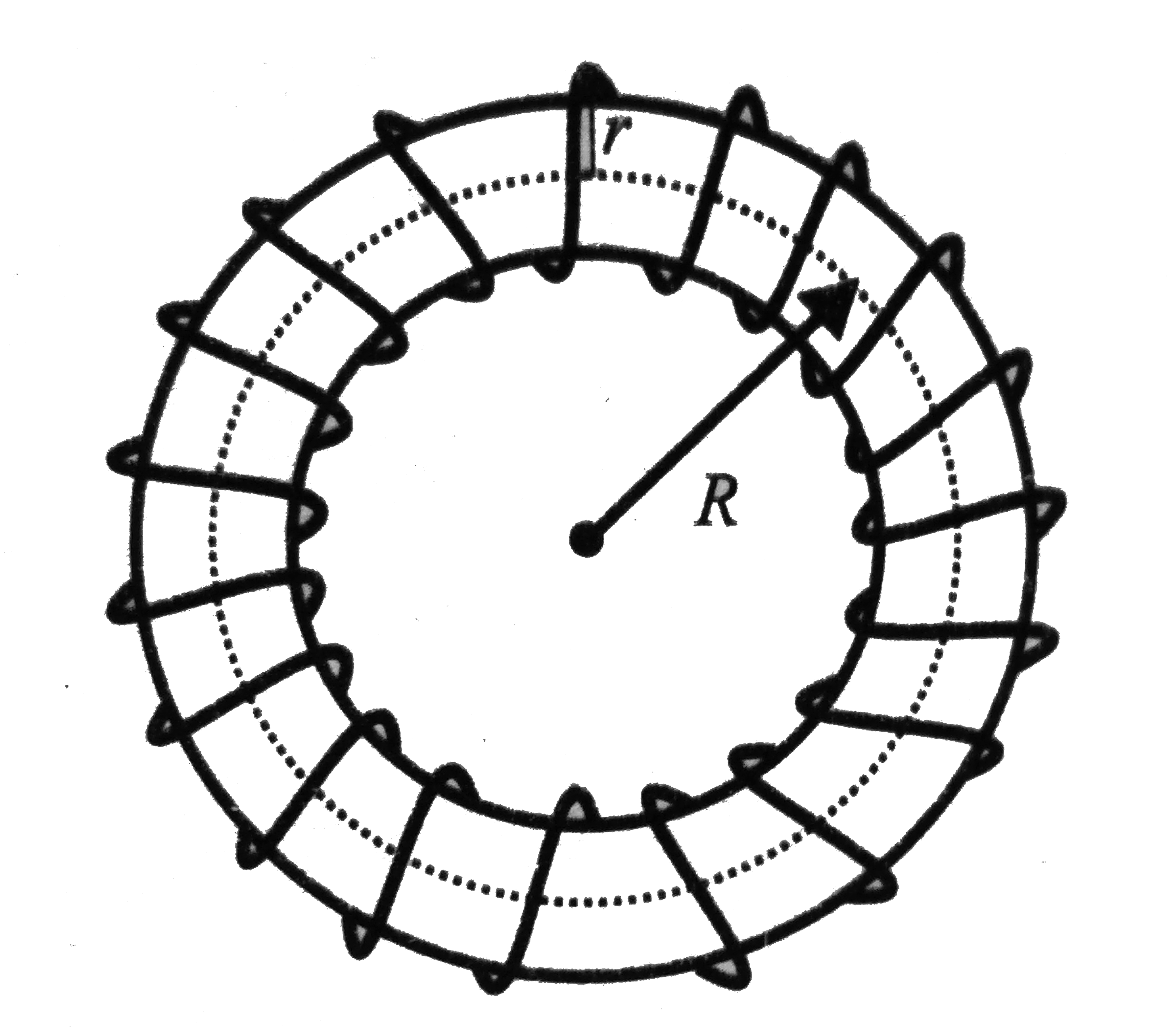
View SolutionA toroid is wound over a circular core. Radius of each turn is r and radius of toroid is R(>>r). The Coefficient of self - inductance of the toroid is given by
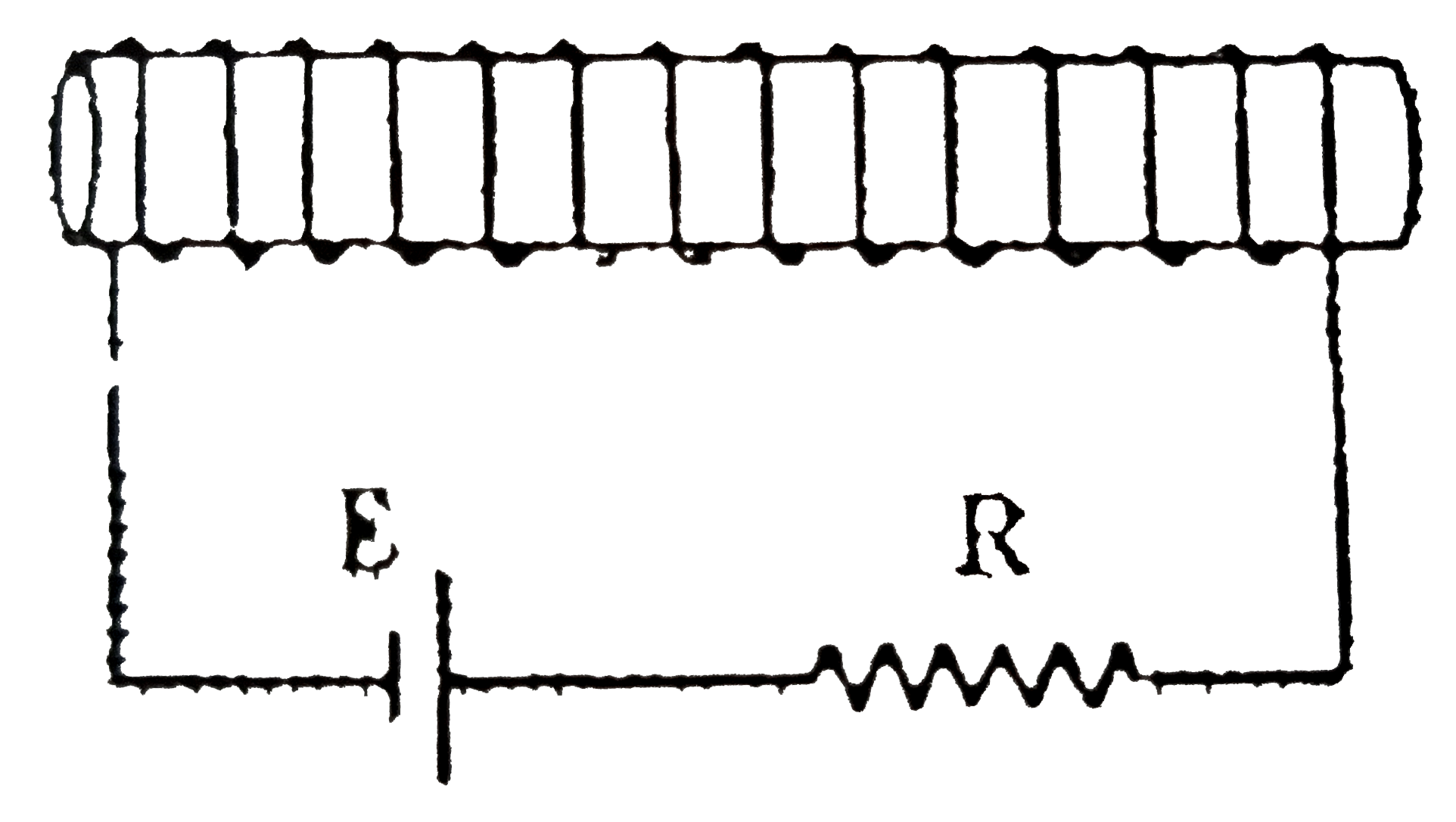
View SolutionAn iron core solenoid of length l and cross-sectional area A having N turns on it is connected to a battery through a resistance as shown in the figure. At instant t=0, the iron rod of permeability μ from the core is abruptly removed. Find the current as a function of time.
View SolutionA toroid is wound over a circular core. Radius of each turn is r and radius of toroid is R(>>r). The coefficient of self-inductance of the toroid is given by
View Solution
