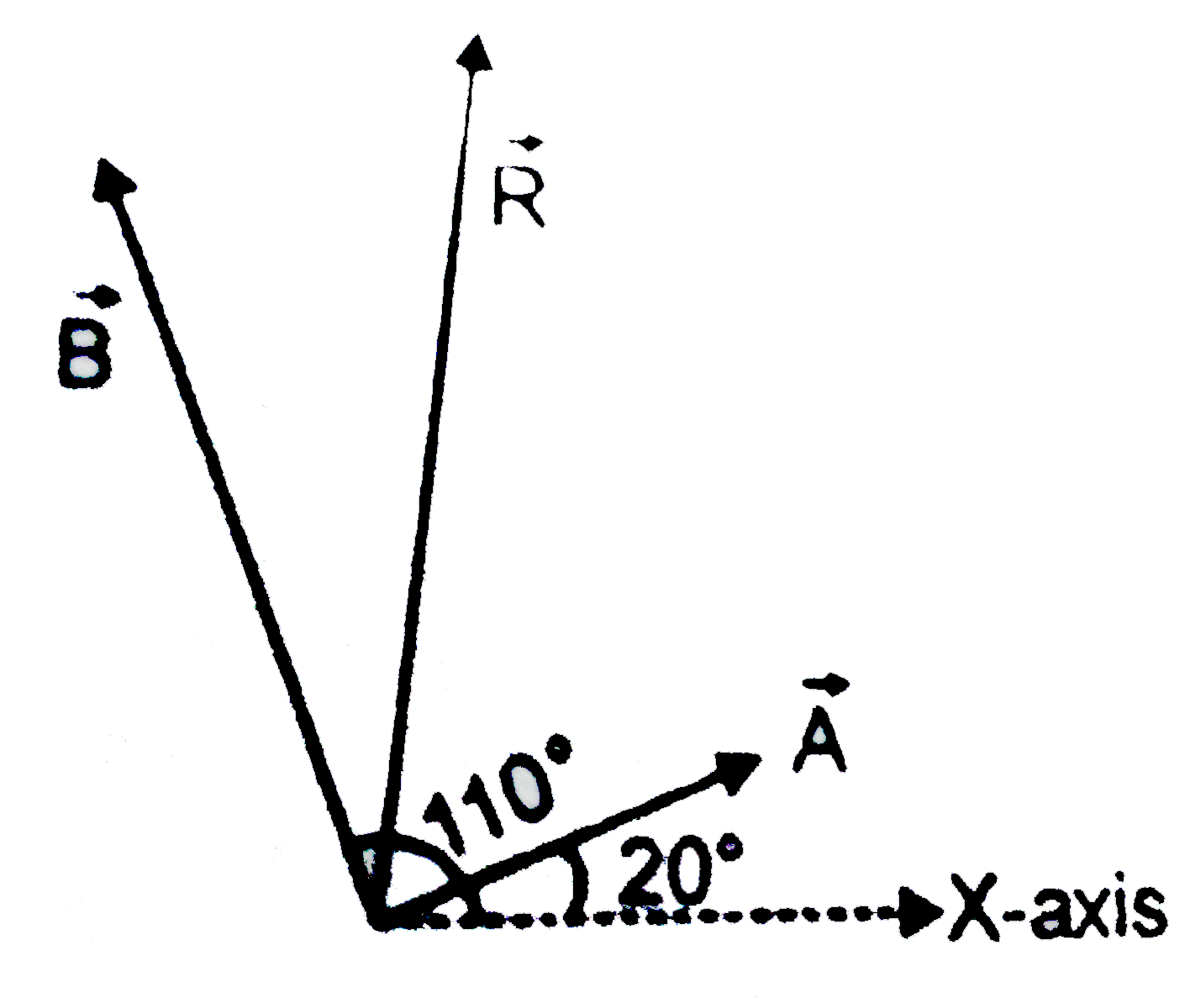
A vector $\to A$ and $\to B$ make angles of $20^{\circ}$ and $110^{\circ}$ respectively with the X-axis. The magnitudes of these vectors are $5 m$ and $12 m$ respectively. Find their resultant vector.

Video Solution

Text Solution

Verified by Experts

angle between the $\to A$ and $\to B = 110^{\circ} - 20^{\circ} = 90^{\circ}$
$R = \sqrt{A^{2} + B^{2} + 2 A B {cos 90}^{\circ}} = \sqrt{5^{2} + 12^{2}} = 13 m$
Let angle of $\to R$ from $\to A$ is $α$
$tan α = \frac{B sin θ}{A + B cos θ} = \frac{12 {sin 90}^{\circ}}{A + B cos θ} = \frac{12 {sin 90}^{\circ}}{5 + 12 {cos 90}^{\circ}} = \frac{12 \times 1}{5 + 12 \times 0} = \frac{12}{5}$
or $α = \frac{{tan}^{- 1} (12)}{5}$ with vector $\to A$ or $(α + 20^{\circ})$ with X-axis

|

Updated on:21/07/2023

Class 12 PHYSICS BASIC MATHS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A vector a makes $30^{\circ}$ and $b$ makes $120^{\circ}$ angle with the $x$ -axis. The magnitude of these vectors are 3 unit and 4 unit, respectively. The magnitude of resultant vector is
A3 unit
B4 unit
C5 unit
D1 unit
Question 2 - Select One
The resultant of $\to a$ and $\to b$ makes $α$ with $\to a$ and $β$ with $\to b$ , then (a,b represent magnitudes of respective vectors) :
A $α < β$
B $α < β if a < b$
C $α < β if a > b$
D $α < β if a = b$
Question 3 - Select One
Two vectors $\to P and \to Q$ are inclinded to each other at $60^{\circ}$ . The magnitude of $\to P and \to Q$ are 10 and 15 units respectively. What is the angle $(α)$ made by their resultant $\to R with \to P$ ?
A $α = {tan}^{- 1} (0.5)$
B $α = {tan}^{- 1} (0.6134)$
C $α = {tan}^{- 1} (0.7422)$
D $α = {tan}^{- 1} (0.92)$

ALLEN-BASIC MATHS-Question

Home
Ask Doubt
Profile