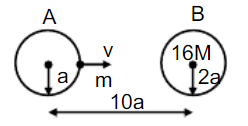
Two planets of mass $M$ and $16 M$ of radius $a$ and $2 a$ respectively, are at distance $10 a$ . Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.

$\sqrt{(\frac{5}{9}) \frac{G M}{a}}$

$\sqrt{(\frac{4}{9}) \frac{G M}{a}}$

$\sqrt{(\frac{3}{9}) \frac{G M}{a}}$

$\sqrt{(\frac{2}{9}) \frac{G M}{a}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Answer

Step by step video, text & image solution for Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - PHYSICS SECTION B
30 Videos

Knowledge Check

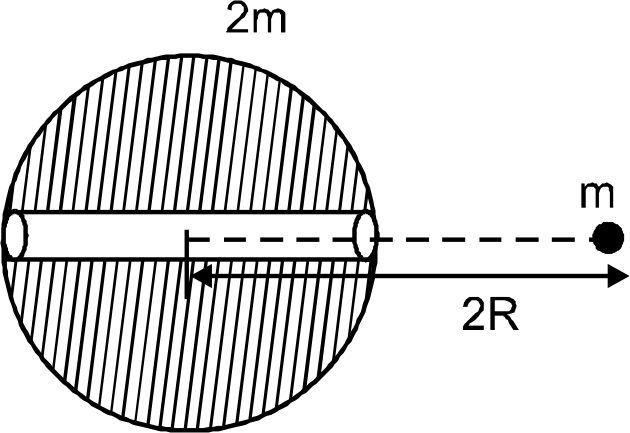
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
A $\frac{4 R}{3}$
Bvelocity of the cosmic particle at that instant is
$\sqrt{\frac{8 G m}{3 R}}$
CTotal work done by the gravitational force on both the particle is $- \frac{2 G m^{2}}{R}$
DTotal work done by the gravitational force on both the particle is $- \frac{2 G m^{2}}{R}$
What is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
A $\frac{5 G m M}{6 R}$
B $\frac{2 G m M}{3 R}$
C $\frac{G m M}{2 R}$
D $\frac{G m M}{3 R}$
What is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
A $\frac{5 G m M}{6 R}$
B $\frac{2 G m M}{3 R}$
C $\frac{G m M}{2 R}$
D $\frac{G m M}{2 R}$

JEE MAINS PREVIOUS YEAR-JEE MAIN-All Questions

Home
Ask Doubt
Profile

Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.