Consider a coaxial cable which consists of an inner wire of radius a surrounded by an outer shell of inner and outer radii b and c respectively. The inner wire carries an electric current $i_{0}$ and the outer shell carries an equal current in opposite direction. Find the magnetic field at a distance x from the axis where (a) $x < a,$ (b) $a < x < b$ , (c) $b < x < c$ and (d) $x > c$ . Assume that the current density is uniform in the inner wire and also uniform in the outer shell.

Video Solution

Text Solution

Verified by Experts

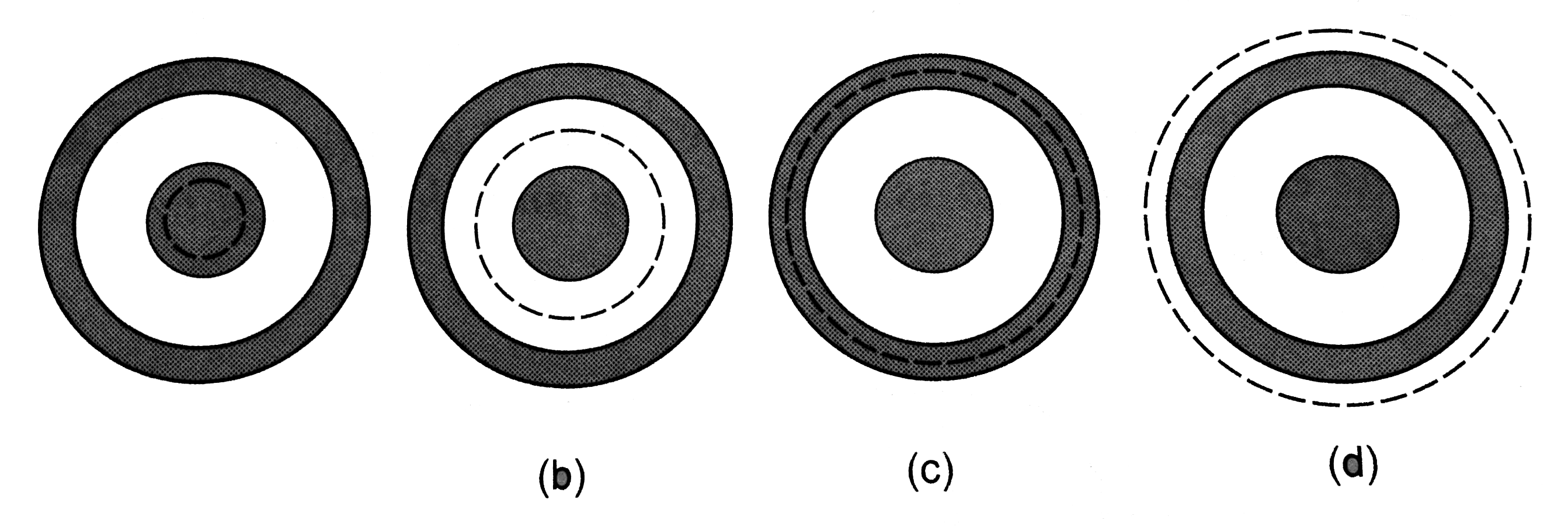
A cross-secton of the cable is shown in figure.Draw a circle of radius $x$ with the centre at the axis of the cable.The parts a,b,c, and $d$ of the figure correspond to the four parts of the problem By symmetry, the magnetic field at each point of a circle will have the same magnitude and will be tangential to it.The circulation of $B$ along the circle is , therefore,
$\oint \to B . d \to l = B 2 π x$
in each of the four parts of the figure.
(a) The current enclosed within the circle in part $b$ is $i_{0}$ so that $\frac{i_{0}}{π a^{2}} . π x^{2} = \frac{i_{0}}{a^{2}} x^{2}$
$\oint \to B . d \to l = μ_{0} i$ gives
$B .2 π x = \frac{μ_{0} l_{0} x^{2}}{a^{2}}$ or $B = \frac{μ_{0} l_{0} x^{2}}{2 π x}$
The direction will be along the tangent of the circle.
(b) The current enclosed within the circle in part $b$ is so that
(c) The area of cross-section of the outer shells is $π c^{2} - π b^{2}$ . The area of cross-section of the outer shell within the circle in part of the figure is $π x^{2} - π b^{2}$
Thus, the current through this pair is $l_{0} \frac{(x^{2} - b^{2})}{(c^{2} - b^{2})} .$ This is in the same direction to the current $i_{0}$ in the inner wire.Thus, the net current enclosed by the circle is
$l_{net} = l_{0} \frac{l_{0} ((x^{2} - b^{2}))}{(c^{2} - b^{2})} = \frac{l_{0} (c^{2} - x^{2} - 2 b^{2})}{(c^{2} - b^{2})} .$
From Ampere's law
$B 2 π x = \frac{l_{0} (c^{2} + x^{2} - 2 b^{2})}{(c^{2} - b^{2})}$ or $B = \frac{μ_{0} (l_{0} (c^{2} + x^{2} - 2 b^{2}))}{2 π x (c^{2} - b^{2})}$
(d) The net current enclosed by the circle in part $d$ of the figure is $2 i_{0}$ and hence
$B 2 π x = μ_{0} 2 i_{0}$ or $B = \frac{u_{0} l_{0}}{π x}$ .

Updated on:21/07/2023

Consider a ????cable which consists of an inner wilre of radius $a$ surrounded by an outer shell of inner and outer radii $b$ and $c$ respectively. The inner wire caries a current $I$ and outer shell carries an equal and opposite current. The magnetic field at a distance $x$ from the axis where $b < x < c$ is
A $\frac{μ_{0} I (c^{2} - b^{2})}{2 π x (c^{2} - a^{2})}$
B $\frac{μ_{0} I (c^{2} - x^{2})}{2 π x (c^{2} - a^{2})}$
C $\frac{μ_{0} I (c^{2} - x^{2})}{2 π x (c^{2} - b^{2})}$
Dzero
In a coaxial, straght cable, the central conductor and the outer conductor carry equal currents in opposite directions.The magnetic field is non-zero.

Aoutside the cable
Binside the inner conductor except axis of the conductor
Call the point inside the outer conductor and outside inner conductor
Donly on axis of conductors
Holow cylindrical wire carries current I, having inner and outer radii R and 2R respectively. The magnetic field at a point which is $\frac{5 R}{4}$ , distance away from the wire?
A $\frac{5 μ_{0} I}{18 π R}$
B $\frac{μ_{0} I}{36 π R}$
C $\frac{5 μ_{0} I}{36 π R}$
D $\frac{3}{40} \frac{μ_{0} I}{π R}$