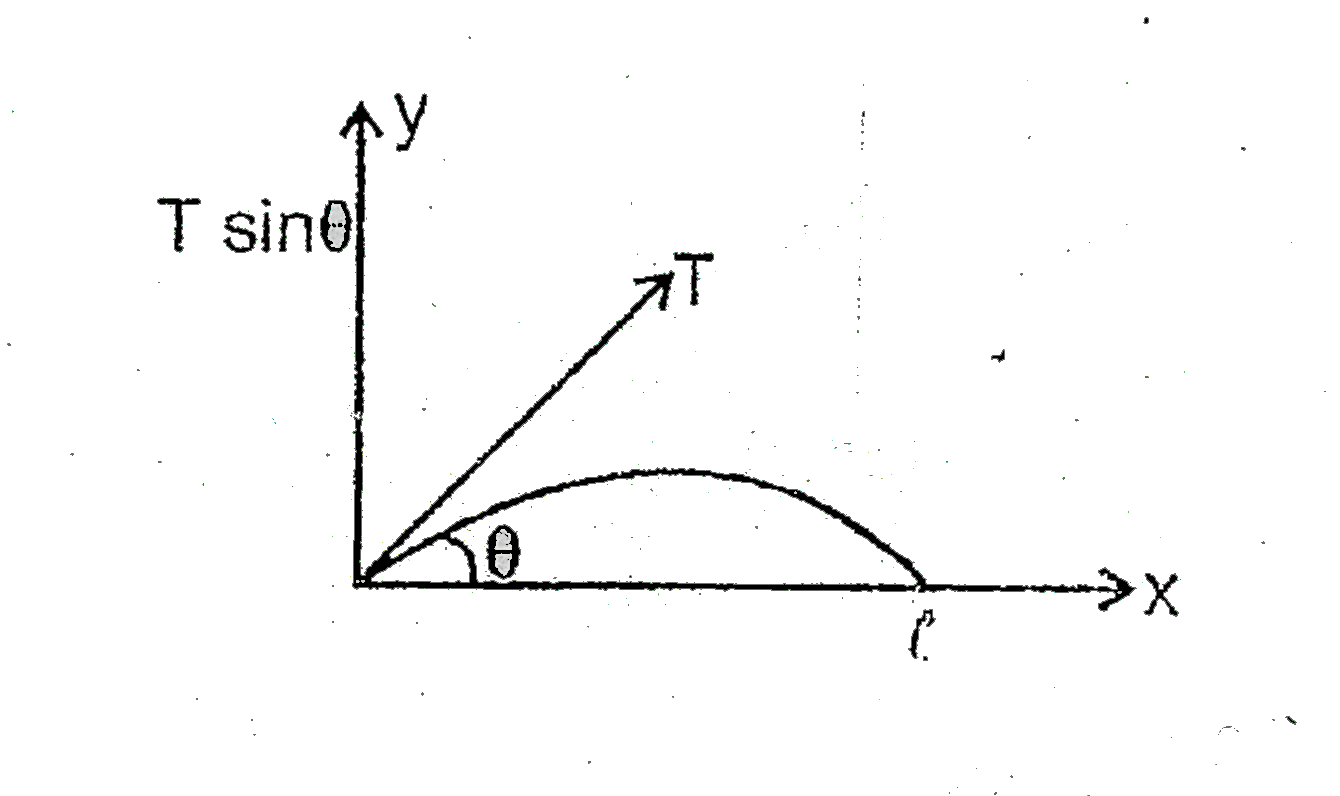
A string of length 50cm is vibrating with a fundamental frequency of 400Hz in horizontal position. In the fundamental mode the maximum displacement at the middle is 2cm from equilibrium position and the tension in the string is 10N. Determine the maximum value of the vertical component of force on the end supporting.
Fy=Tsinθ=tsinθ
=T∂y∂x
y=Asin(ωT−kx)
=0.02sin(ωt−2π0.80×2x)
∂y∂x=−0.02×π0.80cos(ωt−π0.80x)
At x=0
∂y∂x=−280πcosωt
[∂y∂x]max=π40
Fy=[T∂y∂x]=10π40=π4Ns
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A string has a length of 5 cm between fixed points and has a fundamental frequency of 20 Hz. What is the frequency of the second overtone?
A40 HzB50 HzC60 HzD30 Hz - Question 2 - Select One
A sonometer wire having a length of 50 cm is vibrating in the fundamental mode with a frequency of 100Hz. Which of the following is the type of propagating wave and its speed?
ALongitudinal, 50 m/sBTranserse, 50 m/sCLongitudinal, 100 m/sDTransverse, 100 m/s - Question 3 - Select One
An open pipe of length 120 cm vibrates in its fundamental mode. At what distance from one end of the pipe, the pressure variation is maximum ?
AAt 30 cmBAt 60 cmCAt 90 cmDAt 120 cm
A string of length 105 cm produces fundamental frequency of 142 Hz. How much should its length be reduced to produce a fundamental frequency of 213 Hz.
View SolutionA string vibrating with a fundamental frequency of 8 Hz has tension T1. This string vibrates with a fundamental frequency of 15 Hz when the tension is T2 .
Find the fundamental frequency of the string when its tension is T1+T2 .View SolutionThe string length of an instrument is 100 cm and fundamental frequency is 120 Hz. To get a fundamental frquency of 150 Hz, where it should be pressed ?
View SolutionA certain violin wire of length 60cm and mass 4.0gm fixed at both ends vibrates with fundamental frequency of 440Hz. Position of the finger from end of the string when violin plays a note of frequency 550Hz is
View SolutionA string of length l and mass m is fixed at both ends with tension t and excited in its fundamental mode.The maximum displacement at the mid point is A Find (a) the maximum kinetic energy of the string ,9b0 the mean kinetic energy of the srting averaged over one oscillation period
hint Take sine distribution of amplitudesView Solution


