If $cos θ = \frac{4}{5}$ then find the value of $(sin θ cos θ + {tan}^{2} θ)$ .

Video Solution

Text Solution

Verified by Experts

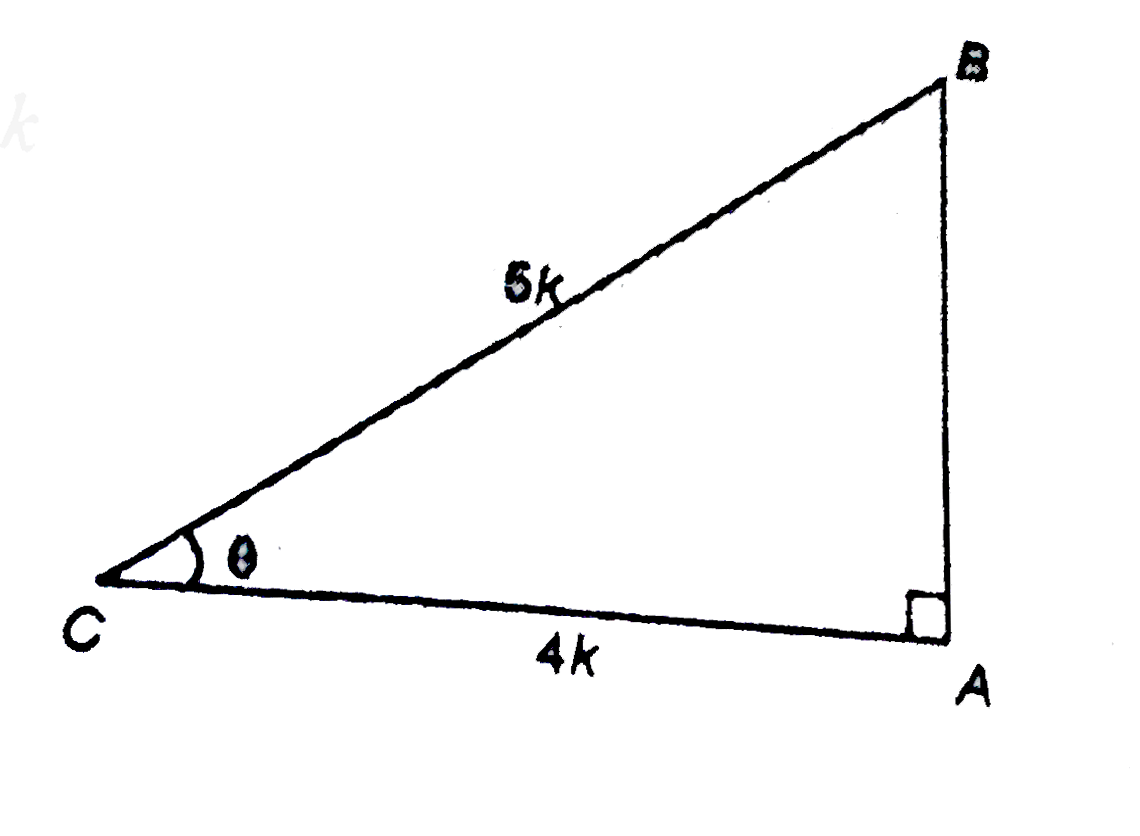
Construct a right - angled $Δ A B C$ in which $< B A C = 90^{\circ}, A C = 4 k$
and BC=5k.
In $Δ A B C$ ,
From Pythagoras theorem
$A B^{2} + A C^{2} = B C^{2}$
$\Rightarrow A B^{2} = B C^{2} - A C^{2} = {(5 k)}^{2} - {(4 k)}^{2}$
$= 25 k^{2} - 16 k^{2} = 9 k^{2}$
$\Rightarrow A B = 3 k$
$∴ sin θ = \frac{A B}{B C} = \frac{3 k}{5 k} = \frac{3}{5}$
$and tan θ = \frac{A B}{A C} = \frac{3 k}{4 k} = \frac{3}{4}$
Now , $sin θ cos θ + {tan}^{2} θ = \frac{3}{5} \cdot \frac{4}{5} + {(\frac{3}{4})}^{2} = \frac{12}{25} + \frac{9}{16}$
$\frac{192 + 225}{400} = \frac{417}{400}$

Updated on:21/07/2023

|

Class 10 MATHS TRIGONOMETRIC RATIOS AND IDENTITIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

If $cos θ = \frac{5}{13}$ , then find the value of ${tan}^{2} θ + {sec}^{2} θ$ .
$0^{\circ} < θ < 90^{\circ} & tan θ = \sqrt{6 + \sqrt{6 + \sqrt{6 + ... . \infty}}}$ , then find the value of ${sec}^{2} θ$ .
A(a) $\frac{39}{23}$
B(b) $\frac{39}{25}$
C(c) $\frac{313}{25}$
D(d) $\frac{329}{21}$

NAGEEN PRAKASHAN-INTRODUCTION TO TRIGONOMETRY-Revision Exercise Long Answer Questions

Home
Ask Doubt
Profile