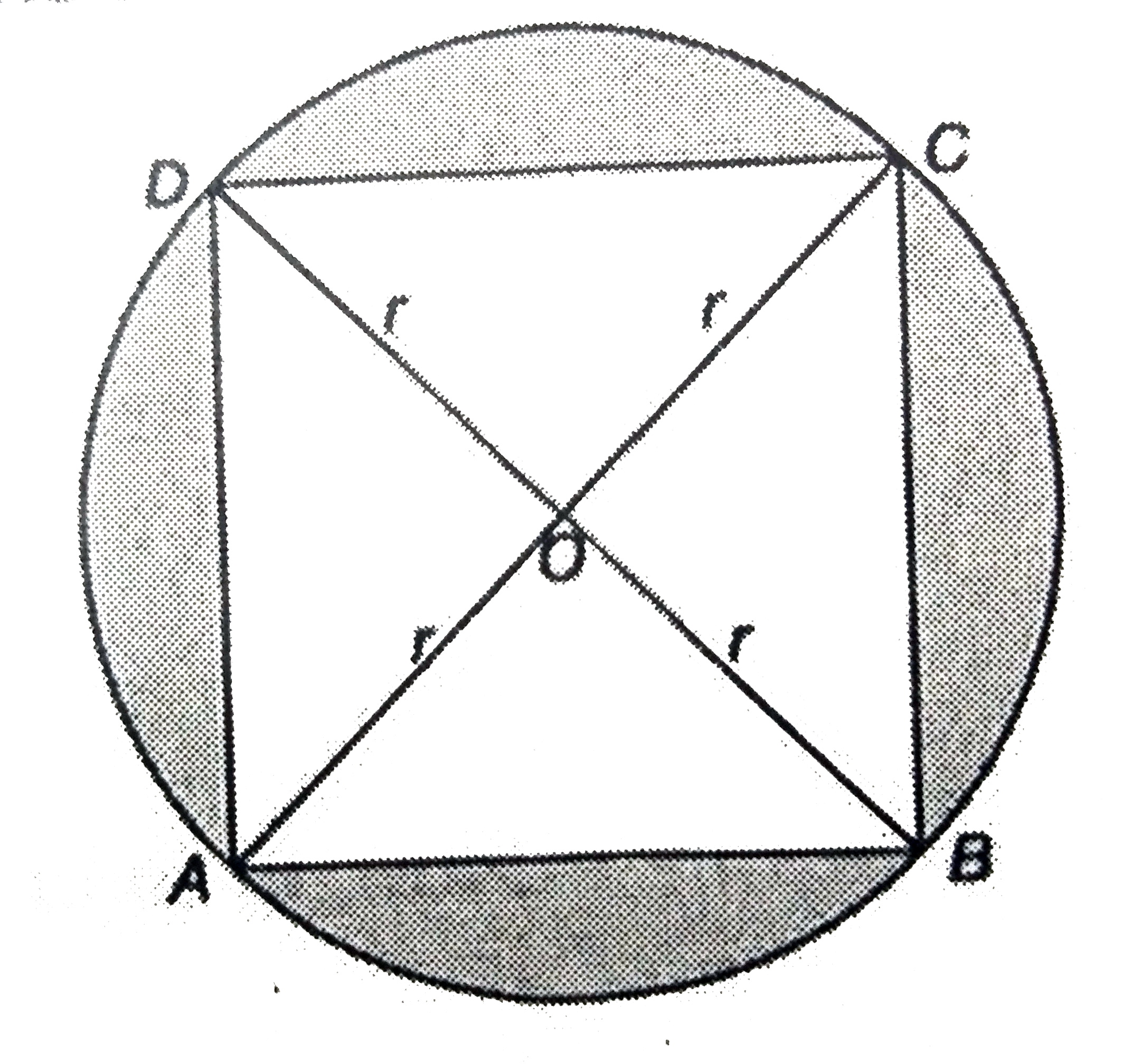
A square of the largest area is cut out of a circle. What % of the are

A square paper is cut into smaller pieces such that each piece is exactly a square, not necessarily of the equal size. From each such square a circle of the largest possible area is cut out. Find the ratio of the area of all the possible circles to the area of the original square.
AA) 1:1
BB) $π : 2$
CC) $π : 4$
DD) data insufficient
The area of a square is twice the area of a circle. The area of the circle is $392 c m^{2}$ . What is the length of the side of the square?
A28 cm
B26 cm
C24 cm
D22 cm
A square of side length 2 cm is circumscribed by a circle, then area of circle is
A $\sqrt{2} π c m^{2}$
B $π c m^{2}$
C $2 π c m^{2}$
D $8 π c m^{2}$

A square of the largest area is cut out of a circle. What % of the area of the circle is lost as trimmings ?