If the equation of mirror is given by y=2/πsinπx(y>0,0≤x≤1) then find the point on which horizontal ray should be incident so that the reflected ray become perpendicular to the incident ray
_E01_073_Q01.png)
(13,√3π)
(√3π,13)
(23,√3π)
(1,0)
The correct Answer is:A,C
_E01_073_S01.png)
y=2πsinπx,dydx=2πcosπ×π⇒dydx=±1
tan45∘=1=2cosπx
cosπx=12⇒πx=nπ3∴x=13and23
∴y=2π×√32(∵sinπ×13=√32)=√3π
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-3
12 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-04[A]
23 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE- 01
45 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
427 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
Unpolarized light is incident on a plane glass surface. What should be the angle of incidence so that the reflected and refracted rays are perpendicular to eachother ?
View SolutionA converging beam of light rays in incident on a concave spherical mirror whose radius of curvature is 0.8m. Determine the position of the point on the optical axis of the mirror where the reflected rays intersect, if the extensions of the incident rays intersect the optical axis 40cm form the mirror's pole.
View Solution
Knowledge Check
A reflecting surface is represented by the equation
y=2Lπsin(πxL), where 0≤x≤L. A ray of light travelling horizontally becomes vertical after reflection with the surface. The co-ordinates of the point where this ray is incident is.
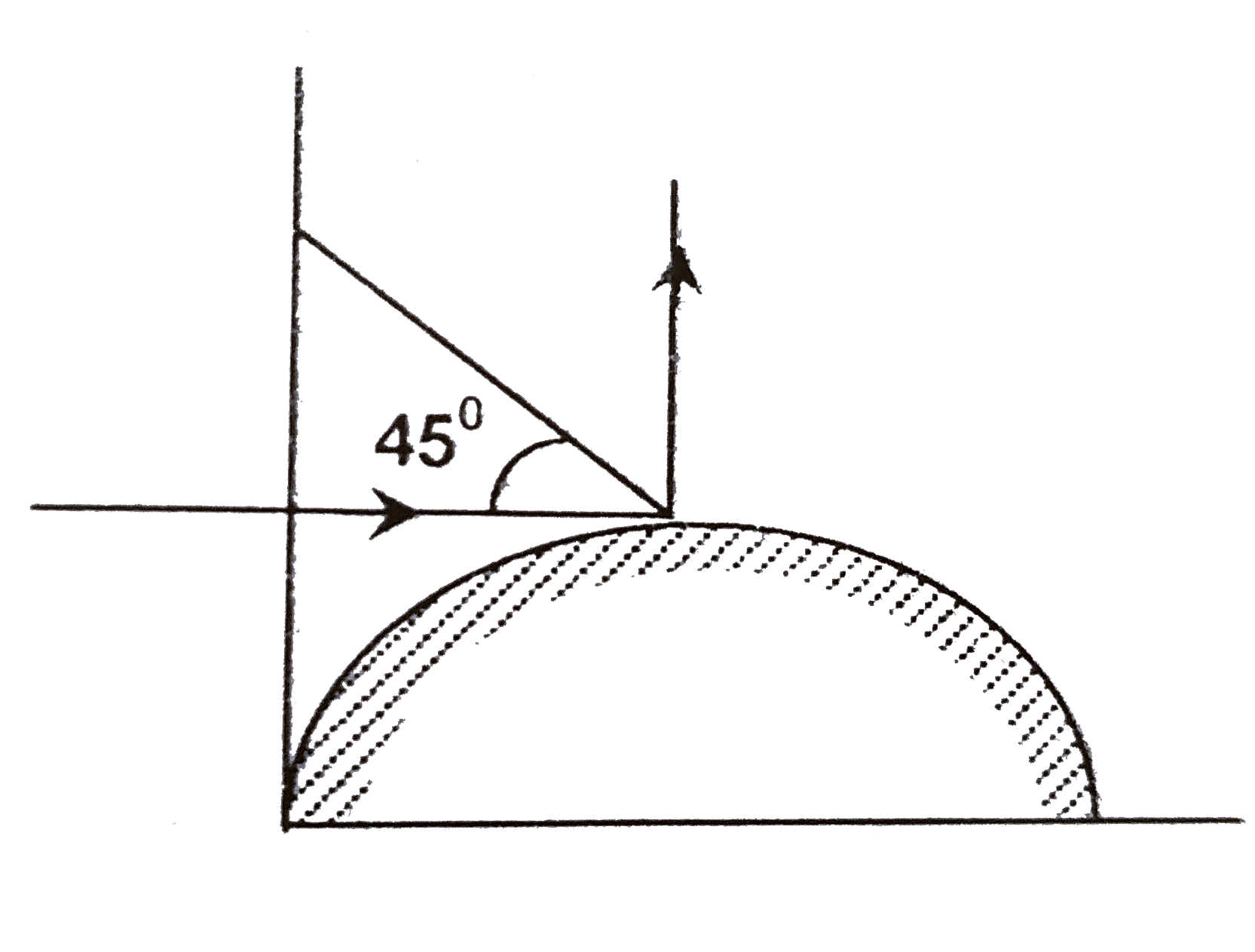 A(L4,√2Lπ)B(L3,√3Lπ)C(3L4,√2Lπ)D(2L3,√3Lπ)
A(L4,√2Lπ)B(L3,√3Lπ)C(3L4,√2Lπ)D(2L3,√3Lπ)A plane mirror, reflecting a ray of incident light, is rotated through an angle θ about an axis through the point of incidence in the plane of the mirror perpendicular to the plane of incidence, then
AThe reflected ray does not rotateBThe reflected ray rotates through an angle θCThe reflected ray rotates through an angle 2θDThe incident ray is fixedA plane mirror, reflecting a ray of incident light, is rotated through an angle θ about an axis through the point of incidence in the plane of the mirror perpendicular to the plane of incidence, then
AThe reflected ray does not rotate.BThe reflected ray rotates through an angle θ.CThe reflected ray rotates through an angle 2θ.DNone of these
A glass plate has a thickness t and refravtive index μ A light ray is allowed to incident in the plate from air. Find at what angle of incidence will the rays refracted and reflected by the plate be perpendicular to each other ? For this angle of incidence, calculate the lateral displacement of the ray
t(μ2−1)μ√1+μ2
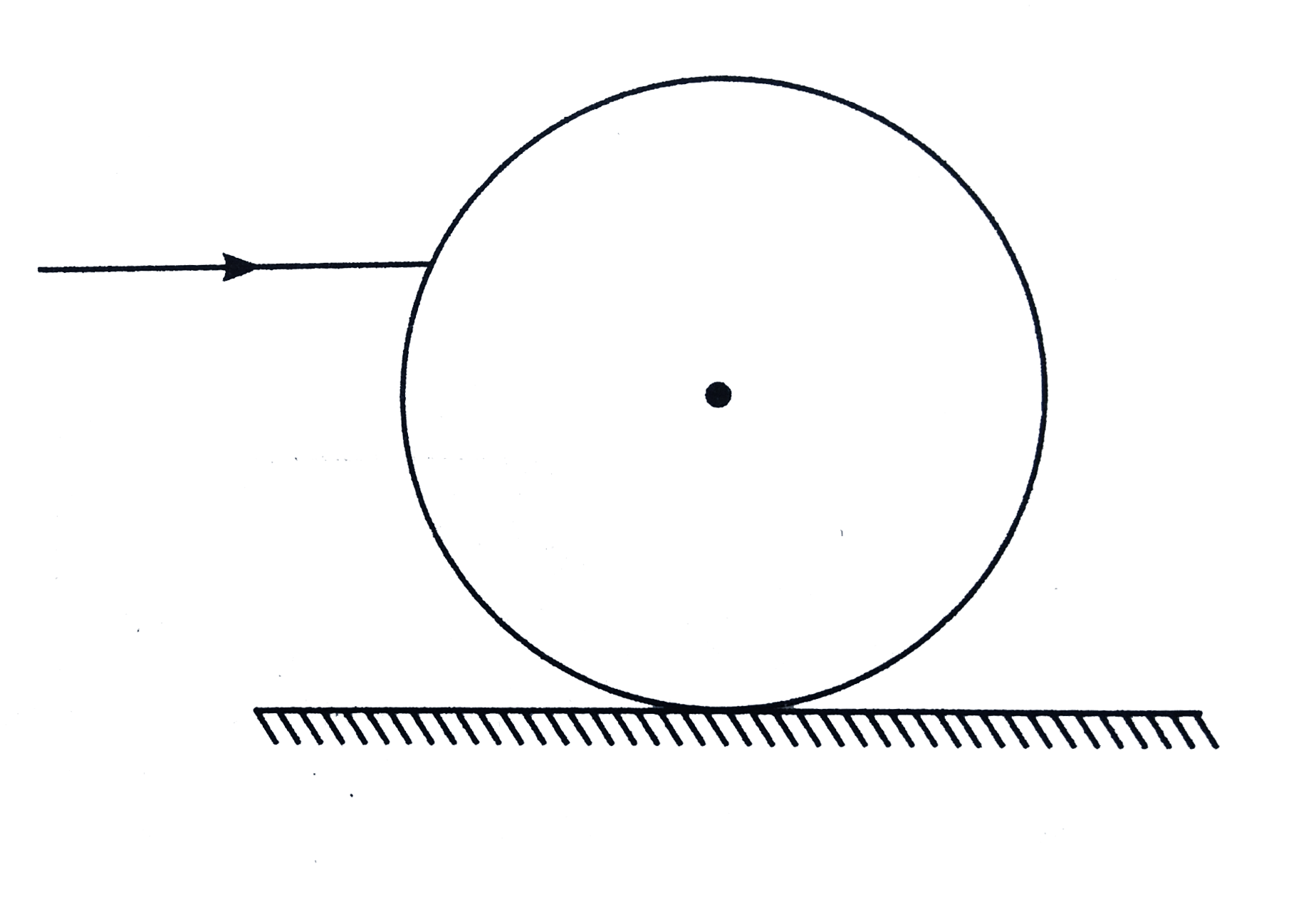
View SolutionA cylindrical glass rod of radius 0.1m and refractive index √3 lies on horizontal plane mirror. A horizontal ray of light moving perpendicular to the axis of the rod is incident on it. At what height from the mirror should the ray be incident so that it leaves the rod at a height of 0.1m above the plane mirror ? At what distance a second similar rod, parallel to the first, be placed on the mirror, such that the emergent ray from the second rod is in line with the incident ray on the first rod ?
View SolutionA cylinderical glass rod of radius 0.1m and refractive index √3 lies on a horizontal plane mirror. A horizontal ray of light moving perpendicular to the axis of the rod is incident on it. At what height from the mirror should the ray be incident so that it leaves the rod at a height of 0.1m above the plane mirror? At what centre to centre distance a second similar rod, parallel to the first, be placed on the mirror, such that the emergent ray from the second rod is in line with the incident ray on the first rod?
View SolutionThe equation sinx+siny+sinz=−3 for 0≤x≤2π,0≤y≤2π,0≤z≤2π has
View SolutionUnpolarized light is incident on a plane glass surface having refractive index . The angle of incidence at which reflected and refracted rays would become perpendicular to each other is :
View Solution
