A light spring of spring constant k is kept compressed between two blocks of masses m and M on a smooth horizontal surface. When released, the blocks acquire velocities in opposite directions. The spring loses contact with the blocks when it acquires natural length. The spring was initially compressed through a distance d find the final speeds of the two blocks.
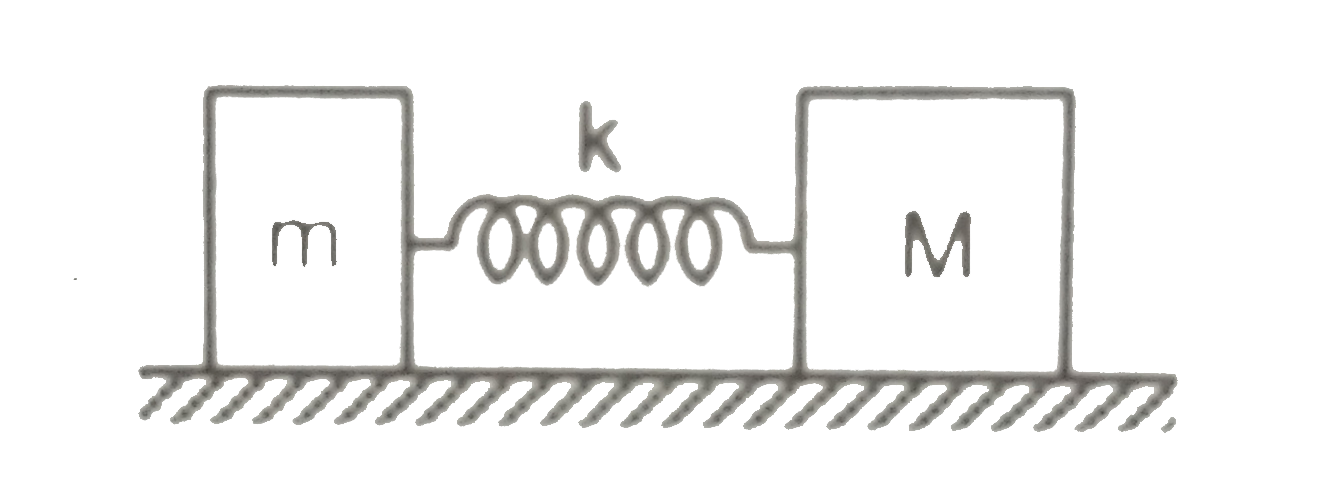
Consider the two blocks plus the spring to be the system. No external force acts on this system in horizontal direction. Hence, the linear momentum will remain constant. Suppose, the block of mass M moves with a speed v1 and the other block with a speedv after losing contact with the spring. From conservation of linear momentum in horizontal direction we have Mv1−Mv2=0 or v1=mMv2 Intially, the energy of the system = 12kx2 Finally, the energy of the system 12mv22+12Mv21 As there is no friction, mechanical energy will remain conserved. Therefore, 12mv22+12Mv21=12kx2 Solving Eqs. (i) and (ii), we get or, v2=[kMm(M+m)]12 x and v1=[kmM(M+m)]12x
Similar Questions
A light spring of spring constant k is kept compressed between two blocks of masses m and M on a smooth horizontal surface. When released, the blocks acquire velocities in opposite directions. The spring loses contact with the blocks when it acquires natural length. I fhte spring was initially compressed through a distance d find the final speeds of the two blocks.

View SolutionA light spring of constant k is kept compressed between two blocks of masses m and M on a smooth horizontal surface. When released, the block acquire velocities in opposite directions. The spring loses contact with the blocks when it acquires natural length. if the spring was initially compressed through a distance x, find the final speeds of the two blocks.
View Solution
Knowledge Check
A light spring of force constant 'K' is held between two blocks of masses 'm' and '2m'. The two blocks and the spring system rests on a smooth horizontal floor. Now th blocks are moved towards each other compressing the springs by 'x' and suddenly released. The relative velocity between the blocks when the spring attains its natural length will be
A(√3K2m)xB(√2K3m)xC(√K3m)xD(√K2m)xTwo blocks of masses m1 and m2 are kept on a smooth horizontal table as shown in figure. Block of mass m1 (but not m2 is fastened to the spring. If now both the blocks are pushed to the left so that the spring is compressed a distance d. The amplitude of oscillatin of block of mass m1 after the system is released is
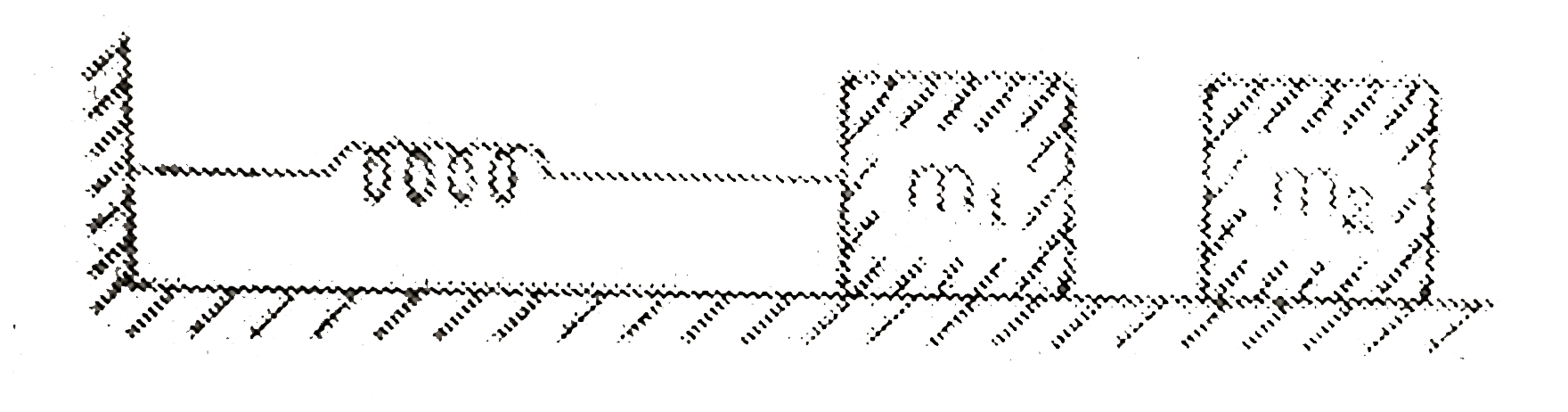 Ad√m1m1+m2Bd√m2m1+m2Cd√2m2m1+m2Dd√(2m1m1+m2
Ad√m1m1+m2Bd√m2m1+m2Cd√2m2m1+m2Dd√(2m1m1+m2An ideal spring is permanently connected between two blocks of masses M and m. The blocks-spring system can move over a smooth horizontal table along a straight line along the length of the spring as shown in Fig.The blocks are brought nearer to compress the spring and then released. In the subsequent motion,
 Ainitially they move in opposite directions with velocities inversely proportional to their massesBthe ratio of their velocities remains constantClinear momentum and energy of the system remain conservedDthe two blocks will Oscillate about their centre of mass which remains stationary
Ainitially they move in opposite directions with velocities inversely proportional to their massesBthe ratio of their velocities remains constantClinear momentum and energy of the system remain conservedDthe two blocks will Oscillate about their centre of mass which remains stationary
In the fig 4.24 a block of mass m moves with velocity v0 toward a stationaly block of a mass M on a smooth horizontal surface. Find the maximum compression in the spring of stiffnesss constant K.

View SolutionThe two blocks A and B of same mass connected to a spring and placed on a smooth surface. They are given velocities (as shown in the figure) when the spring is in its natural length :
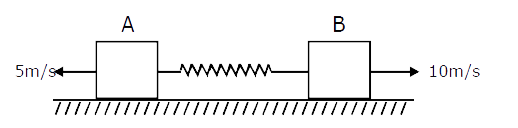
View SolutionTwo blocks A and B of masses m & 2m placed on smooth horizontal surface are connected with a light spring. The two blocks are given velocities as shown when spring is at natural length.
(i) Find velocity of centre of mass (b) maximum extension in the spring
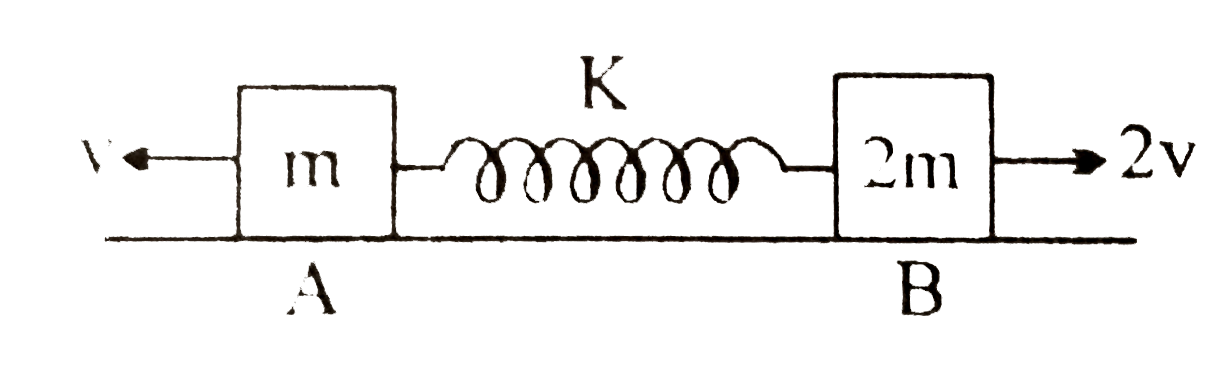
View SolutionA block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. Two maximum compression in spring is

View SolutionA spring, placed horizontally on a rough surface is compressed by a block of mass m, placed on the same surface so as to store maximum energy in the spring. If the coefficient of friction between the block and the surface is μ, the potential energy stored in the spring is
View Solution
