An Lc circuit contains a 40 mH inductor and a $25 μ F$ capacitor. The resistance of the circuit is negligible.The time is measured from the instant the circuit is closed. The energy stored in the circuit is completely magnetic at time (in milliseconds)

$0, 3.14, 6.28$

$0, 1.57, 4.71$

$1.57, 4.71, 7.85$

$1.57, 3.14, 4.71$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

An $L C$ circuit contains a $20 m H$ inductor asn a $50 μ F$ capacitor with initial change of $10 m C$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t = 0$ .

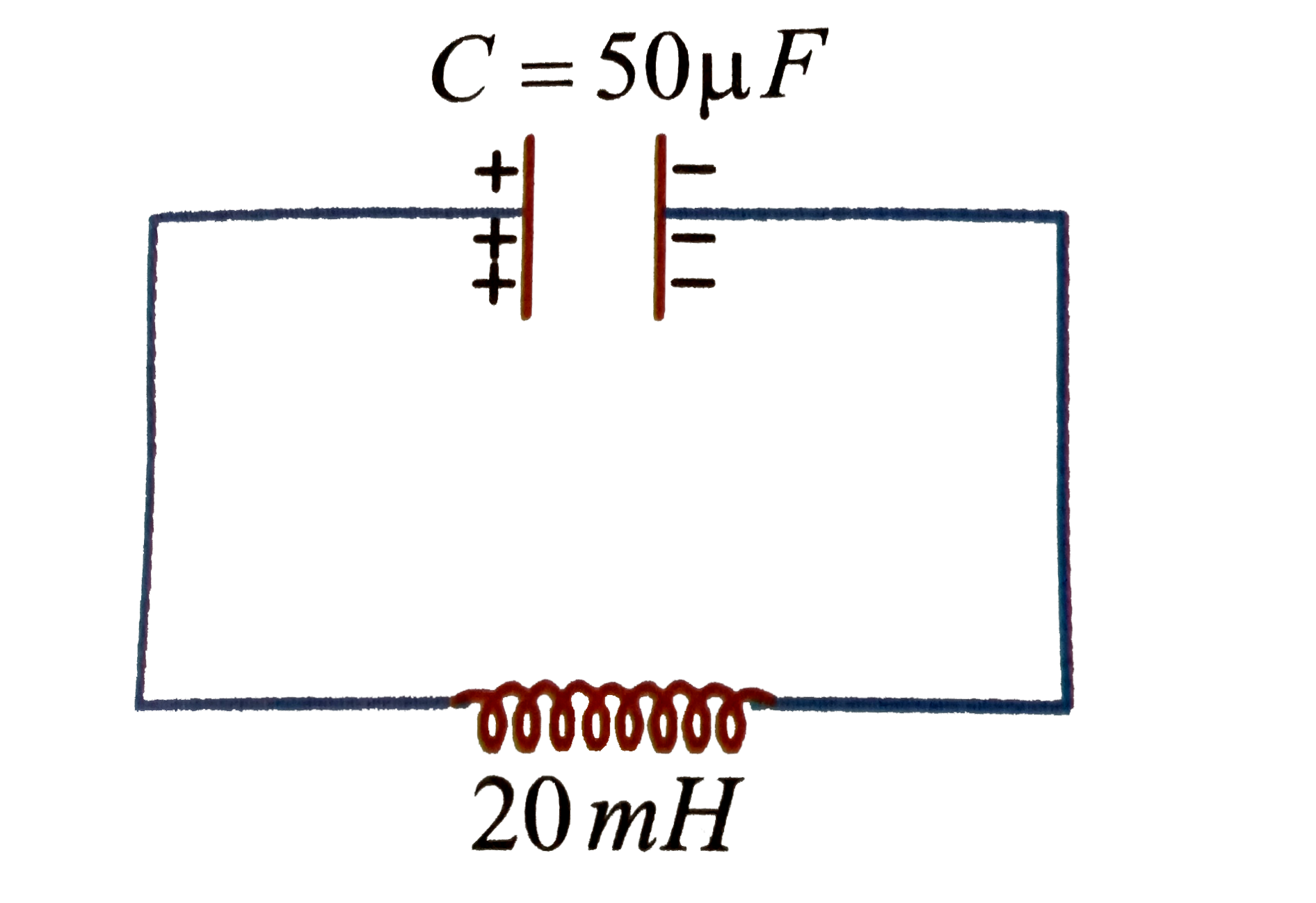AEnergy stored in the circuit in completely electrical at $t = \frac{n π}{2000}$
BEnergy stored in the circuitin completely magnetic at $t = \frac{(2 n + 1) π}{2000}$
CEnergy stored in the circuit in shared equally between the inductor and capacitor at $t = \frac{(2 n + 1) π}{4000}$
DEnergy stored in the circuit is shared euqally between the inductor and capacitor at $t = \frac{n π}{2000}$
An LC circuit contains a 20 mH inductor and a $50 μ F$ capacitor with an initial charge of 10 mC. The resistance of the circuit is negligible. Let the instant at which the circuit which is closed be t=0. At what time the energy stored is completely magnetic ?
At = 0
B $t = 1.54 m s$
C $t = 3.14 m s$
D $t = 6.28 m s$
In the circuit shown, the energy stored in $1 μ F$ capacitor is
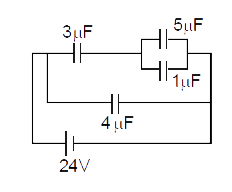A $40 μ J$
B $64 μ J$
C $32 μ J$
Dnone of these