Two bodies at different temperatures T1andT2 , if brought in thermal contact do not necessarily settle to the mean temperature (T1+T2)/2 Explain.
To explain why two bodies at different temperatures T1 and T2 do not necessarily settle to the mean temperature T1+T22 when brought into thermal contact, we can follow these steps:
1. Understanding Heat Transfer:
When two bodies at different temperatures are brought into thermal contact, heat will flow from the hotter body (at temperature T1) to the colder body (at temperature T2). This process continues until thermal equilibrium is reached, meaning both bodies will eventually reach a common final temperature Tf.
2. Heat Exchange Equation:
The heat lost by the hot body is equal to the heat gained by the cold body. Mathematically, this can be expressed as:
Heat lost by hot body=Heat gained by cold body
This can be written as:
m1s1(T1−Tf)=m2s2(Tf−T2)
where m1 and m2 are the masses of the hot and cold bodies, respectively, and s1 and s2 are their specific heats.
3. Rearranging the Equation:
Rearranging the above equation gives:
m1s1T1+m2s2T2=(m1s1+m2s2)Tf
4. Final Temperature Calculation:
From the rearranged equation, we can solve for the final temperature Tf:
Tf=m1s1T1+m2s2T2m1s1+m2s2
5. Conditions for Mean Temperature:
The mean temperature T1+T22 will only be reached if:
- The masses of both bodies are equal (m1=m2).
- The specific heats of both bodies are equal (s1=s2).
6. Conclusion:
If the masses and specific heats of the two bodies are not equal, the final temperature Tf will not be the arithmetic mean T1+T22. Instead, it will be weighted by the masses and specific heats of the two bodies, leading to a final temperature that is dependent on these factors.
When two bodies at different temperatures are brought into thermal contact, heat will flow from the hotter body (at temperature T1) to the colder body (at temperature T2). This process continues until thermal equilibrium is reached, meaning both bodies will eventually reach a common final temperature Tf.
The heat lost by the hot body is equal to the heat gained by the cold body. Mathematically, this can be expressed as:
Heat lost by hot body=Heat gained by cold body
This can be written as:
m1s1(T1−Tf)=m2s2(Tf−T2)
where m1 and m2 are the masses of the hot and cold bodies, respectively, and s1 and s2 are their specific heats.
Rearranging the above equation gives:
m1s1T1+m2s2T2=(m1s1+m2s2)Tf
From the rearranged equation, we can solve for the final temperature Tf:
Tf=m1s1T1+m2s2T2m1s1+m2s2
The mean temperature T1+T22 will only be reached if:
- The masses of both bodies are equal (m1=m2).
- The specific heats of both bodies are equal (s1=s2).
If the masses and specific heats of the two bodies are not equal, the final temperature Tf will not be the arithmetic mean T1+T22. Instead, it will be weighted by the masses and specific heats of the two bodies, leading to a final temperature that is dependent on these factors.
Topper's Solved these Questions
- THERMODYNAMICS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - THERMODYNAMICS EXERCISE - SHORT ANSWER TYPE QUESTIONS (3 MARKS)
10 Videos
- THERMODYNAMICS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - THERMODYNAMICS EXERCISE - LONG ANSWER TYPE QUESTIONS (5 MARKS)
1 Videos
- THERMODYNAMICS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - THERMODYNAMICS EXERCISE - MULTIPLE CHOICE QUESTIONS (MCQs)
20 Videos
- ROTATIONAL MOTION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ROTATIONAL MOTION EXERCISE - MULTIPLE CHOICE QUESTIONS
20 Videos
- WORK, ENERGY AND POWERBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - WORK, ENERGY AND POWEREXERCISE - MCQ
20 Videos
Similar Questions
Two bodies at different temperature T1 and T2. If brought in thermal contact do not mecesserily settle to the mean temperature (T1+T2)/2.
View SolutionExplain why
(a) Two bodies at different temperature T1andT2 if brought in thermal contact do not necessarily settle to the mean temperature (T1+T2)/2 ?
(b) The coolant in a chemical or nuclear plant (i.e., the liquid used to prevent different parts of a plant from getting too hot)should have high specific heat. Comment.
(c) Air pressure in a car tyre increases during driving . Why?
(d) The climate of a harbour town is more temperature (i.e., without extremes of heat and cold) than that of a town in a desert at the same latitude. Why?
View Solution
Knowledge Check
Assertion : Two bodies at different temperatures if brought in thermal contact do not necessary settle to the mean temperature
Reason : The two bodies may have different thermal capacities.AIf both the assertion and reason are true and reason is the correct explanation of the assertion.BIf both the assertion and reason are true but the reason is not correct explanation of the assertion.CIf the assertion is true but the reason is falseDIf the both the assertion and reason are false.This section contains Assertion and Reason type questions. Each question has 4 choices (1), (2), (3) and (4), out of which only one is correct.
Assertion (A) Two bodies at different temperature if brought in thermal contact do not necessarily settle to a mean temperature.
Reason (R) The two bodies may have different thermal capacities.ABoth Assertion and Reason are correct and Reason Is the correct explanation of the AssertionBBoth Assertion and Reason are correct but Reason Is not the correct explanation of AssertionCAssertion Is correct but Reason Is IncorrectDAssertion Is incorrect but Reason Is correctIf two bodies at different temperatures T1 and T2 are brought in thermal contact, the mean temperature T1+T22. When
Amass of two bodies are equalBpressure on two bodies are equalCtherma capacities of two bodies are equalDvolume of two bodies are equal
Two curves are given at temperatures T1andT2 in an isothermal process, then-
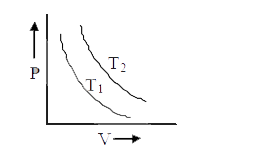
View SolutionThe graph of (xm) vs P at two different temperature T1 and T2 is [where T1>T2]
View SolutionThe most probable speed of the molecules in a gas at temperature T2 is equal to the average speed of the molecules at temperature T1 Find T2/T1
View SolutionA rod of length l with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as k=a/T, where a is a constant. The ends of the rod are kept at temperatures T1andT2. Find the function T(x), where x is the distance from the end whose temperature is T1.
View SolutionValue of Equilibrium constant at two different temperature is given as:
then the value of △H of reaction, △G1 and △G2 at temperature T1&T2 respectively are :
View Solution
