The water acidity in a pool is considered normal when the average pH reading of three daily measurements is between 8.2and8.5 If the first two pH readings are 8.48and8.35, then find the range of pH value for the third reading that will result in the acidity level being normal.
To solve the problem step by step, we need to find the range of the third pH reading (let's call it x) that will keep the average pH of the three readings between 8.2 and 8.5.
Step 1: Set Up the Average
The average pH of the three readings can be expressed as:
Average=8.48+8.35+x3
Step 2: Establish the Inequality
We know that the average must be between 8.2 and 8.5. Therefore, we can write the inequality:
8.2<8.48+8.35+x3<8.5
Step 3: Multiply by 3
To eliminate the fraction, multiply all parts of the inequality by 3:
3×8.2<8.48+8.35+x<3×8.5
This simplifies to:
24.6<8.48+8.35+x<25.5
Step 4: Combine the Known Values
Now, combine the known pH readings:
8.48+8.35=16.83
So, we can rewrite the inequality as:
24.6<16.83+x<25.5
Step 5: Isolate x
Now, we will isolate x in both parts of the inequality.
1. For the left side:
24.6−16.83<x
Calculating this gives:
7.77<x
2. For the right side:
16.83+x<25.5
Isolating x gives:
x<25.5−16.83
Calculating this gives:
x<8.67
Step 6: Combine the Results
Now we can combine both parts of the inequality:
7.77<x<8.67
Conclusion
Thus, the range of the third pH reading x that will keep the average pH level normal is:
(7.77,8.67)
The average pH of the three readings can be expressed as:
Average=8.48+8.35+x3
We know that the average must be between 8.2 and 8.5. Therefore, we can write the inequality:
8.2<8.48+8.35+x3<8.5
To eliminate the fraction, multiply all parts of the inequality by 3:
3×8.2<8.48+8.35+x<3×8.5
This simplifies to:
24.6<8.48+8.35+x<25.5
Now, combine the known pH readings:
8.48+8.35=16.83
So, we can rewrite the inequality as:
24.6<16.83+x<25.5
Now, we will isolate x in both parts of the inequality.
24.6−16.83<x
Calculating this gives:
7.77<x
16.83+x<25.5
Isolating x gives:
x<25.5−16.83
Calculating this gives:
x<8.67
Now we can combine both parts of the inequality:
7.77<x<8.67
Thus, the range of the third pH reading x that will keep the average pH level normal is:
(7.77,8.67)
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - NCERT EXEMPLARCHAPTER - LINEAR INEQUALITIESEXERCISE - Long Answer Type Question
6 Videos
- LINEAR INEQUALITIESBOOK - NCERT EXEMPLARCHAPTER - LINEAR INEQUALITIESEXERCISE - Objective Type Questions
14 Videos
- LIMITS AND DERIVATIVES BOOK - NCERT EXEMPLARCHAPTER - LIMITS AND DERIVATIVES EXERCISE - FILLERS
4 Videos
- MATHEMATICAL REASONING BOOK - NCERT EXEMPLARCHAPTER - MATHEMATICAL REASONING EXERCISE - OBJECTIVE TYPE QUESTIONS
21 Videos
Similar Questions
The water acidity in a pool is considered normal when the average pH reading of three daily measurements is between 7.2 and 7.8. If the first two pH reading are 7.48 and 7.85, find the range of pH value for the third reading that will result in the acidity level being normal.
View Solutionhe water acidity in a pool is considered normal when the average ph reading of three daily measurement is between 7.4 and 8.2. If the first two ph reading are 7.48 and 8.42. Find the range of ph value for the third reading that will result in the acidity level being normal
View SolutionThe water aciditiy in a pond is considered normal when the average pH reading of three daily measurement is between 7.1 and 7.8. If the first two pH readings are 7.25 and 7.8,find the range of pH of the water of the pond for the 3rd reading that will result in the acidity level being normal.
View SolutionThe average of 8 readings is 24.3. Out of which the average of first two is 18.5 and that of next three is 21.2. If the sixth reading is 3 less than seventh and 4 more than eighth, what is the sixth reading?
View SolutionWhy does the rain water normally have a pH of about 5.6 ? When does it become acid rain?
View SolutionWhy does the rain water normally have a pH of about 5.6 ? When does it become acid rain ?
View SolutionWhy does the rain water normally have a pH of about 5.6 ? When does it become acid rain ?
View SolutionTwo solutions A and B have pH values of 5 and 8 respectively . Which solution will be basic in nature ?
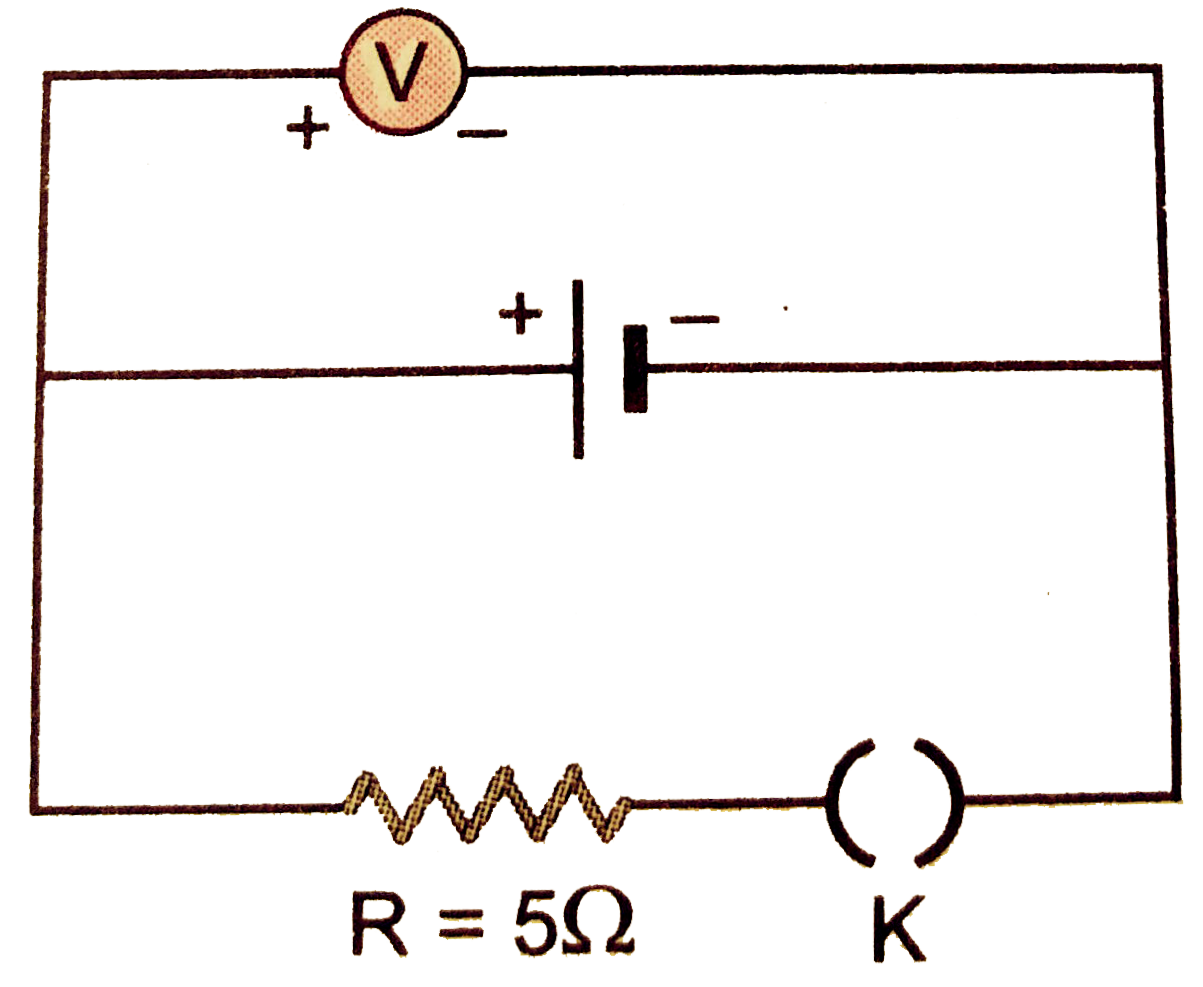
View SolutionThe reading on a high resistance voltmeter. When a cell is connected across it is 2.2V. When the terminals of the cell are connected to a resistance of 5Ω the voltmeter reading drop to 1.8V. Find the internal resistance of the cell.
View SolutionThe pH of 10−8 M acid solution lies between .........and .............. .
View Solution
