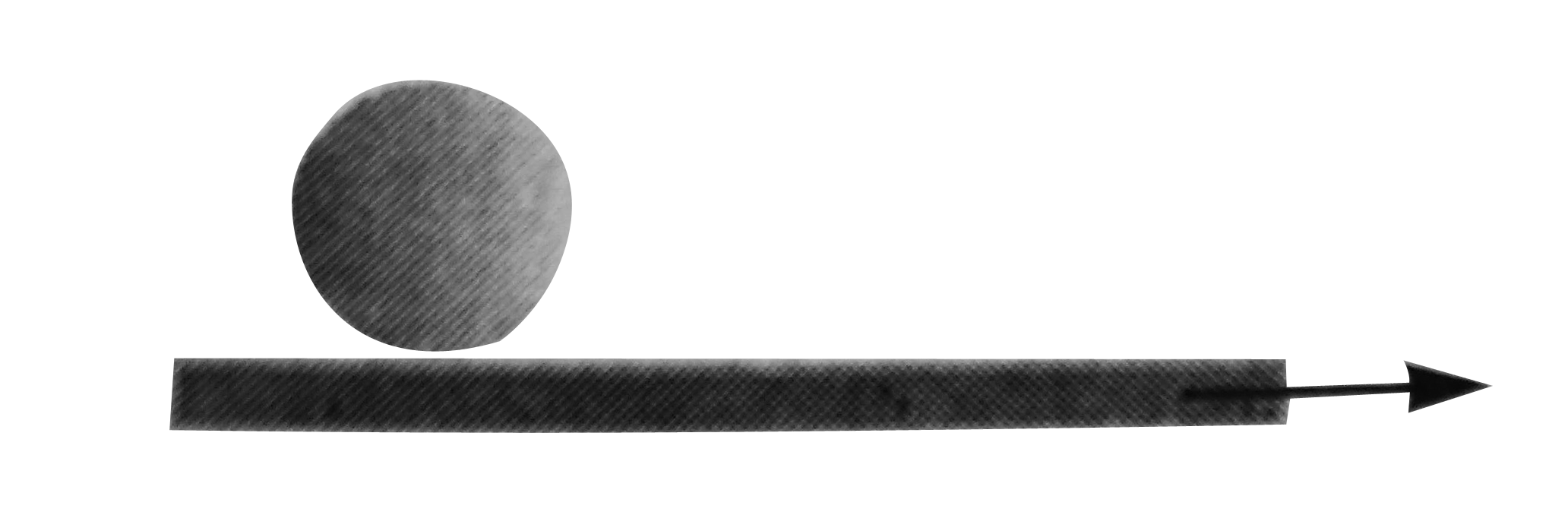
A solid sphere of mass m and radius R is gently placed on a conveyer belt moving with constant velocity v0. If coefficient of friction between belt and sphere is 2/7 the distance traveled by the centre of the sphere before it starts pure rolling is
v207g
2v2049g
2v205g
2v207g
The correct Answer is:A
Nμ=ma⇒a=gμ⇒NμR=23mR2α⇒5gμ2Rv=gμt,ω=5gμ2Rt
Pure rolling starts when v+Rω=v0
⇒gμt+52gμt=v0⇒t=2v07gμ

s=12at2=12gμ4v2049g2μ2=2v2049gμ⇒s=2v2049g(2/7)v207g
Similar Questions
A suitcase is gently dropped on a conveyor belt moving at 3ms−1 If the coefficient of friction between the belt and suitcase is 0.5 how far will the suitcase move on the belt before coming to rest ?
View Solution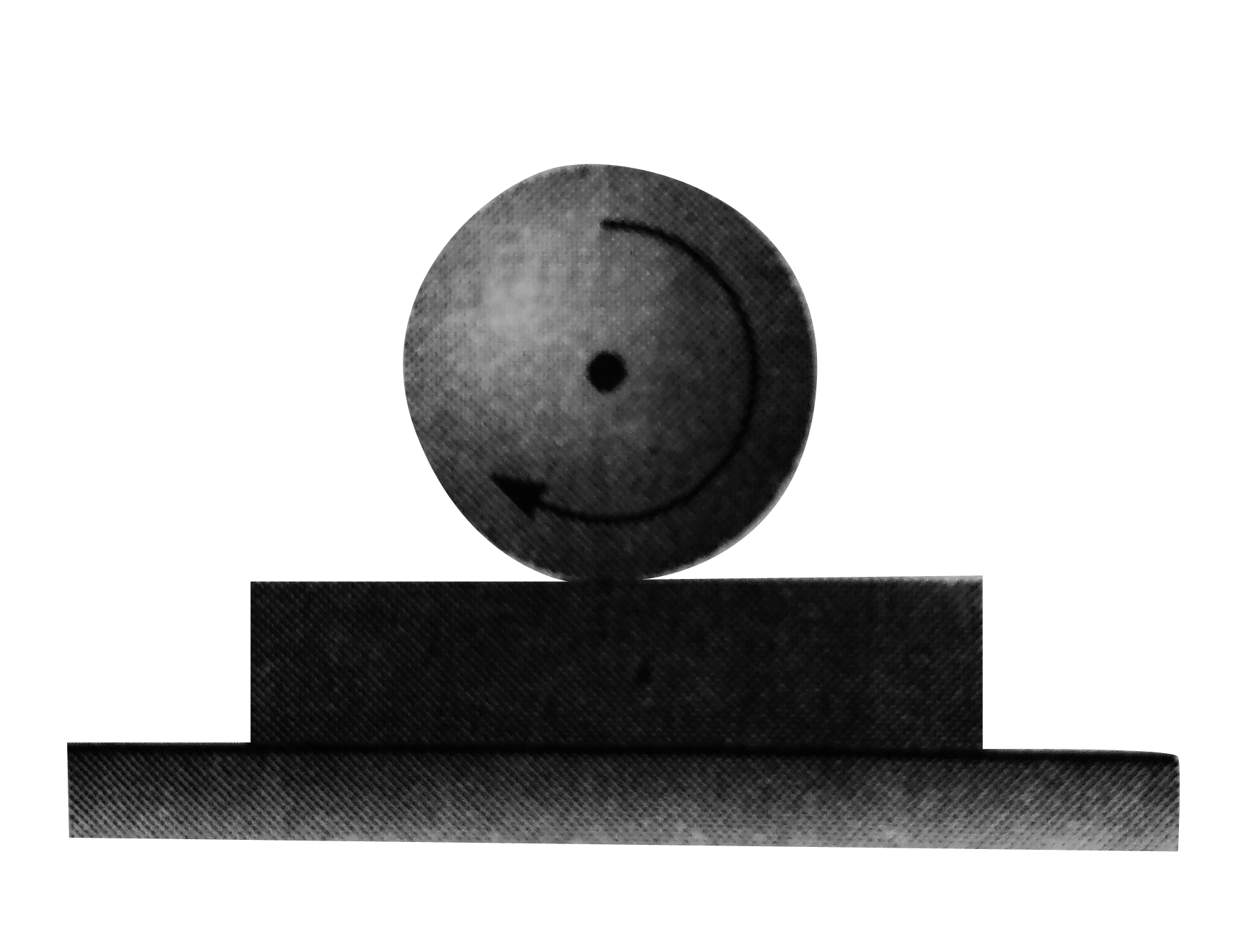
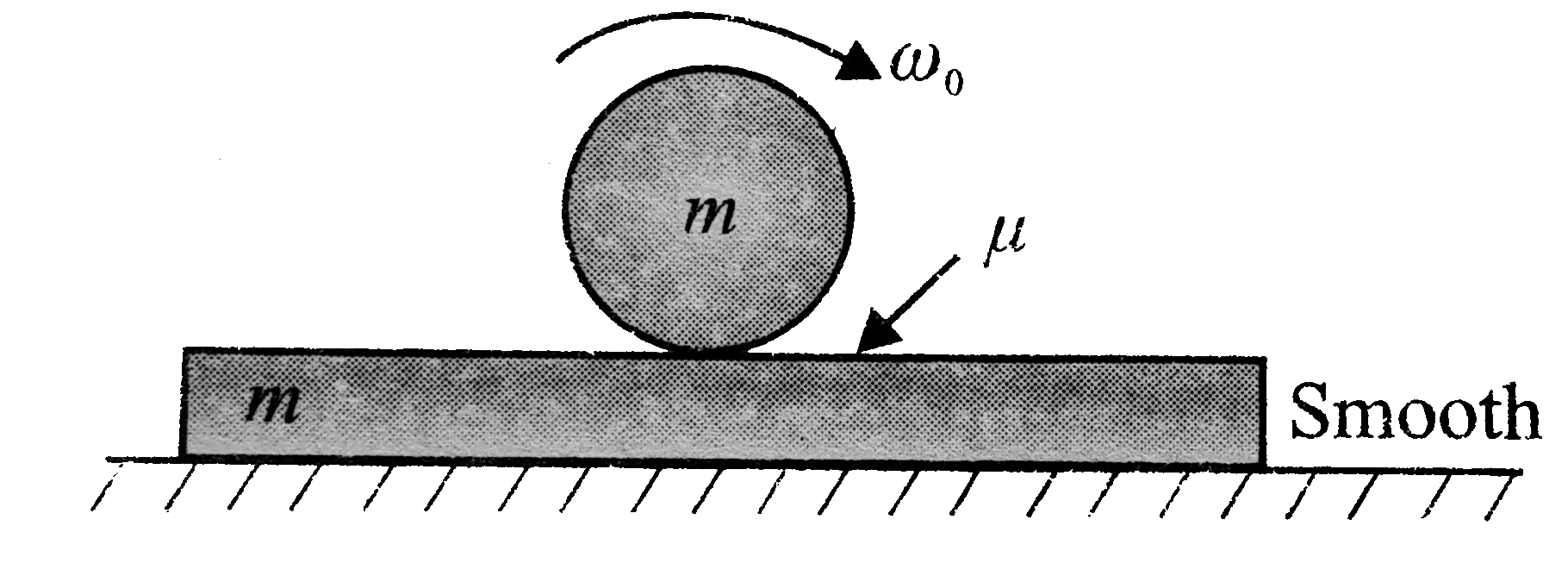
A horizontal plank having mass m lies on a smooth horizontal surface. A sphere of same mass and radius r is spined to angular frequency ω0 and gently placed on the plank as shown in the figure. If coefficient of friction between the plank and the sphere is μ. Find the distance moved by the plank till sphere starts pure rolling on the plank. the plank is long enough.
View Solution
Knowledge Check
An object is gently placed on a long converges belt moving with 11ms−1. If the coefficient of friction is 0.4, then the block will side in the belt upto a distance of
A10.21 mB15.125 mC20.3 mD25.6 mA hollow sphere of mass m and radius R is rolling downdard on a rough inclined plane of inclination θ. If the coefficient of friction between the hollow sphere and incline is μ, then
AFriction opposes its translationBFriction supports rotation motionCOn decreasing θ, frictional force decreasesDAll of theseA sphere of mass m and radius r is projected in a gravity free space with speed v. If coefficient of viscosity of the medium in which it moves is 16π , the distance travelled by the body before it stops is
Amv2rB2mvrCmvrDmv4r
A long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.
Find the velocity of the sphere after the pure rolling starts
View SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.

Find the displacement of the plank till the sphere starts pure rolling.
View SolutionAn object is gently placed on a long conveyer belt moving with a speed of 5 ms−1 If the coefficient of friction between the object and the belt is 0.5, the block will slide on the belt up to a distance (Take g = 10 ms−2)
View SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.

Find the velocity of the sphere after the pure rolling starts
View SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.

Find the displacement of the plank till the sphere starts pure rolling.
View Solution
