Question

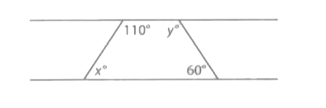
$98<x<102$
$103<y<107$
$\begin{array}{cc}\text{Quantity A}& \text{Quantity B}\\ y-x& |y-x|\end{array}$

Options

A. Quantity A is greater.
B. Quantity B is greater.
C. The two quantities are equal.
D. The relationship cannot be determined from the infromation given.

Answer

C

Explanation

To solve the problem, we need to analyze the given inequalities and the expressions for Quantity A and Quantity B.

1. Identify the ranges for x and y:
- From the problem, we know that:
\( 98 < x < 102 \)
\( 103 < y < 107 \)

2. Determine the maximum and minimum values:
- The maximum value of \( x \) is just under 102, and the minimum value of \( y \) is just over 103. Thus, we can conclude:
\( x < 102 \quad \text{and} \quad y > 103 \)

3. Compare y and x:
- Since \( y \) is always greater than \( x \) (because the smallest \( y \) can be is just over 103 and the largest \( x \) can be is just under 102), we can write:
\( y - x > 0 \)

4. Define Quantity A and Quantity B:
- Quantity A is defined as:
\( \text{Quantity A} = y - x \)
- Quantity B is defined as:
\( \text{Quantity B} = |y - x| \)

5. Evaluate the relationship between Quantity A and Quantity B:
- Since we established that \( y - x > 0 \), it follows that:
\( |y - x| = y - x \)
- Therefore, we can conclude:
\( \text{Quantity A} = \text{Quantity B} \)

6. Final conclusion:
- Since both quantities are equal, we can state:
\( \text{Quantity A} = \text{Quantity B} \)

Final Answer:
Both quantities are equal.