Consider a horizontally oriented syringe containing water locate at a height of 1.25m above the ground. The diameter of the plunger is 8mm and the diameter of the nozzle is 2mm. The plunger is pushed with a constant speed of 0.25m/s. Find the horizontal range of water stream on the grond. Take g=10m/s2
The correct Answer is:2
From equation of continuity (Av = constant )
π4(8)2(0.25)=π4(2)2(v) …(i)
Here, v is the velocity of water with which water comes out of the syringe (Horizontally) Solving equation (i), we get v=4m/s
The path of water after leaving the syringe will be a parabola.
Range , R=ut=u√2hg⇒R=4√2×1.2510⇒R=410×5=2m
Similar Questions
Consider a horizontally oriented syringe containing water located of a height of 1.25m above the ground. The diameter of plunger is 8mm and the diameter if the nozzle is 2mm. The plunger is pushed with a constant speed of 0.25m/s. Find the horizontal range of water stream on the ground. Take g=10m/s2.
.
View Solution_E01_216_Q01.png)
A syringe containing water is held horizontally with its nozzle at a height h above the ground as shown in fig the cross sectional areas of the piston and the nozzle are A and a respectively the piston is pushed with a constant speed v. Find the horizontal range R of the stream of water on the ground.
View Solution
Knowledge Check
A particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1mA particle is projected at an angle 60∘ with the horizontal with a speed 10m/s. Then, latus rectum is (Take g=10m/s2)
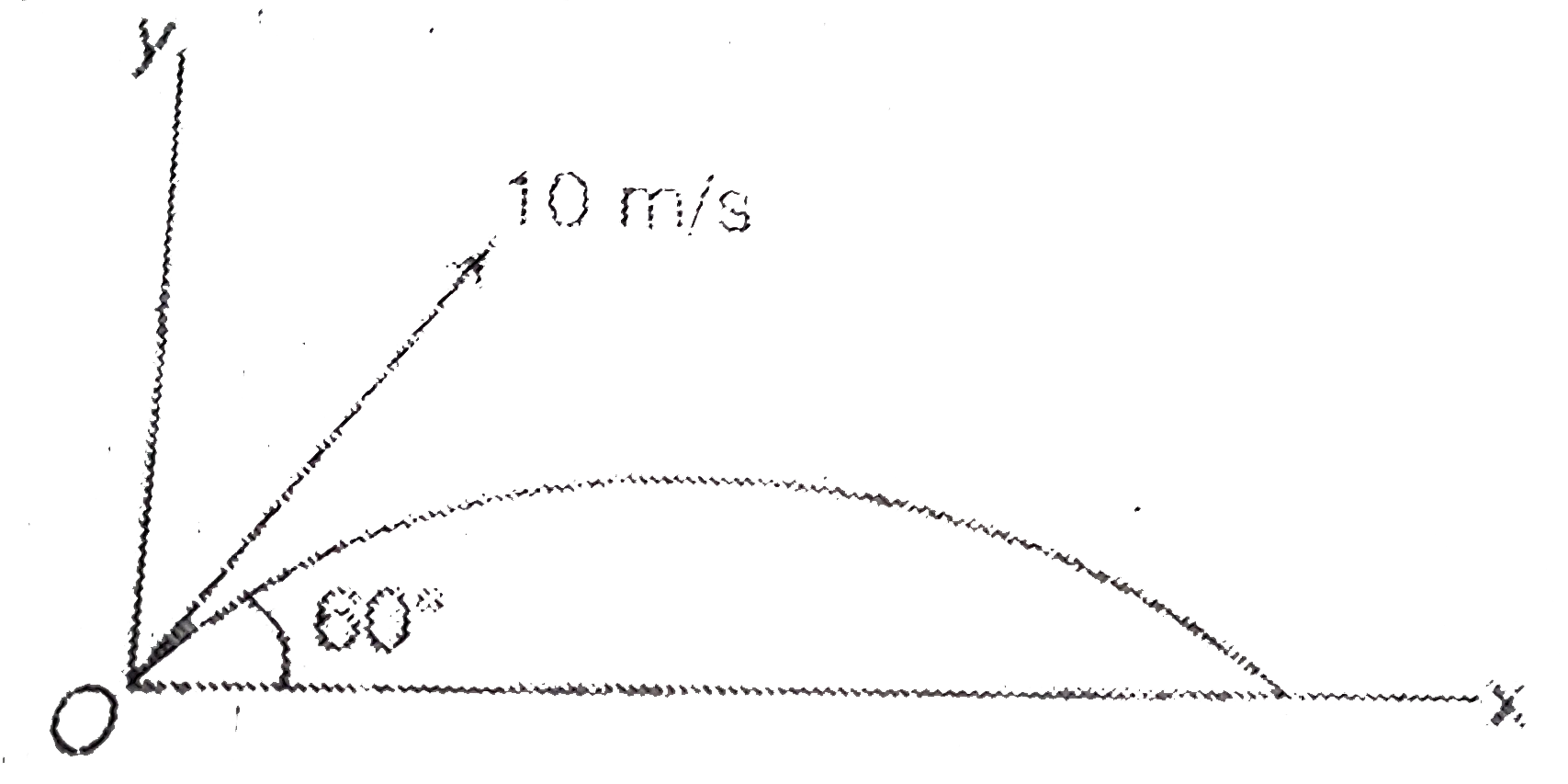A5mB15mC10mD0
A ball is thrown up at a speed of 4.0 m/s. Find the maximum height reached by the ball. Take g=10m/s2.
View SolutionWater is flowing at a speed of 0.5 m/s through a horizontal pipe of internal diameter 3 cm. Determine the diameter of the nozzle if the water is to emerge at a speed of 3 m/s.
View SolutionOil flows in a pipeline at a speed of 0.8 m/s. The oil flow rate is 2×103 tons/h. Find the diameter of the pipeline. 19.2. The internal diameter of a nozzle is 2 cm. It emits
View SolutionWater flow througyh a horizontal pipe whose internal diameter is 2.0cm at a speed of 1.0ms−1 What should be the diameter of the nozzle, If the water is to emerge at a speed of 4.0ms−1?
View SolutionFind the energy possessed by an object of mass 10 kg when it is at a height of 6 m above the ground.Given, g=9.8m/s2
View SolutionA syringe of diameter D = 8 mm and having a nozzie of diameter d = 2 mm is placed horizontally at a height of 1.25 m as shown in the figure . An incompressible and non - viscous liquid is filled in syringe and the piston is moved at speed of 0.25 m/s .Find the range of liquid jet on the ground.
View Solution