A ball A moving with momentum 2ˆi+4ˆj collides with identical ball B moving with momentum 6ˆj . After collision momentum of ball B is 10ˆj . Which of the following statement is correct?
After collision momentum of A is 4ˆi
After collision momentum of A is parallel to momentum of B
After collision momentum of A is 2ˆi
If →PAand→PB are momenta of balls A and B, then Δ(∣∣→PA∣∣+∣∣→PB∣∣)=0
The correct Answer is:C
To solve the problem, we need to analyze the momentum of the two balls before and after the collision.
1. Identify the initial momentum of both balls:
- Ball A has an initial momentum of →pA=2^i+4^j.
- Ball B has an initial momentum of →pB=0^i+6^j.
2. Calculate the total initial momentum:
- The total initial momentum →pinitial is the vector sum of the momenta of both balls:
→pinitial=→pA+→pB=(2^i+4^j)+(0^i+6^j)=2^i+10^j
3. Identify the final momentum of ball B:
- After the collision, the momentum of ball B is given as →p′B=0^i+10^j.
4. Let’s denote the final momentum of ball A as →p′A.
- We know that momentum is conserved, so the total final momentum →pfinal must equal the total initial momentum:
→pfinal=→p′A+→p′B=→p′A+(0^i+10^j)
5. Set up the equation for momentum conservation:
→pinitial=→pfinal⟹2^i+10^j=→p′A+(0^i+10^j)
6. Solve for →p′A:
- By equating the components, we find:
- In the ^i direction:
2 = p_{A_x}' \quad \text{(where p_{A_x}' isthe \hat{i} componentof \vec{p_A'} )}
- In the ^j direction:
10=p′Ay+10⟹p′Ay=0
7. Thus, the final momentum of ball A is:
→p′A=2^i+0^j=2^i
8. Conclusion:
- The correct statement is that after the collision, the momentum of ball A is 2^i.
- Ball A has an initial momentum of →pA=2^i+4^j.
- Ball B has an initial momentum of →pB=0^i+6^j.
- The total initial momentum →pinitial is the vector sum of the momenta of both balls:
→pinitial=→pA+→pB=(2^i+4^j)+(0^i+6^j)=2^i+10^j
- After the collision, the momentum of ball B is given as →p′B=0^i+10^j.
- We know that momentum is conserved, so the total final momentum →pfinal must equal the total initial momentum:
→pfinal=→p′A+→p′B=→p′A+(0^i+10^j)
→pinitial=→pfinal⟹2^i+10^j=→p′A+(0^i+10^j)
- By equating the components, we find:
- In the ^i direction:
2 = p_{A_x}' \quad \text{(where p_{A_x}' isthe \hat{i} componentof \vec{p_A'} )}
- In the ^j direction:
10=p′Ay+10⟹p′Ay=0
→p′A=2^i+0^j=2^i
- The correct statement is that after the collision, the momentum of ball A is 2^i.
Similar Questions
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA ball of mass m moving with a speed u1 collides elasticity with another identical ball moving with velocity u2.
(a) Find the velocities of the balls after collision if the impact is direct.
(b) Find the angle between velocities after collision if they collide obliquely and u2=0
View Solution
Knowledge Check
Given A=3ˆi+4ˆjandB=6ˆi+8ˆj which of the following statement is correct ?
AA×B=0B|A||B|=12C|A|=15DA.|B|=48A ball of mass 1 kg moving with velocity →v=3ˆi+4ˆj collides with a wall and after collision velocity of the ball is −2ˆi+6ˆj . Which of the following unit vectors is perpendicular to wall?
A15(−3ˆi−4ˆj)B1√29(5ˆi+2ˆj)C1√40−(2ˆi+6ˆj)D1√40(5ˆi−2ˆj)A ball with initial momentum →P collides with rigid wall elastically. If −→P1 be its momentum after collision then
A−→P1=→PB−→P1=−→PC−→P1=2→PD−→P1=−2→P
Ball of mass 5.0 kilograms moving at 20m/s collides with ball B of unknown mass moving at 10m/s in the same direction . After collision ball A moves at 10m/s an ball B at 15m/s , both still in the same direction. What is the mass of ball B?
View SolutionA billiard ball moving with a speed of 5m/s collides with an identical ball, originally at rest. If the first ball stop dead after collision, then the second ball will move forward with a speed of:
View SolutionA billiard ball moving with a speed of 5m/s collides with an identical ball, originally at rest. If the first ball stop dead after collision, then the second ball will move forward with a speed of:
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The speed of each ball after the collision is
View SolutionA ball of mass m moving with velocity v, collide with the wall elastically as shown in the figure. After impact the change in angular momentum about P is :
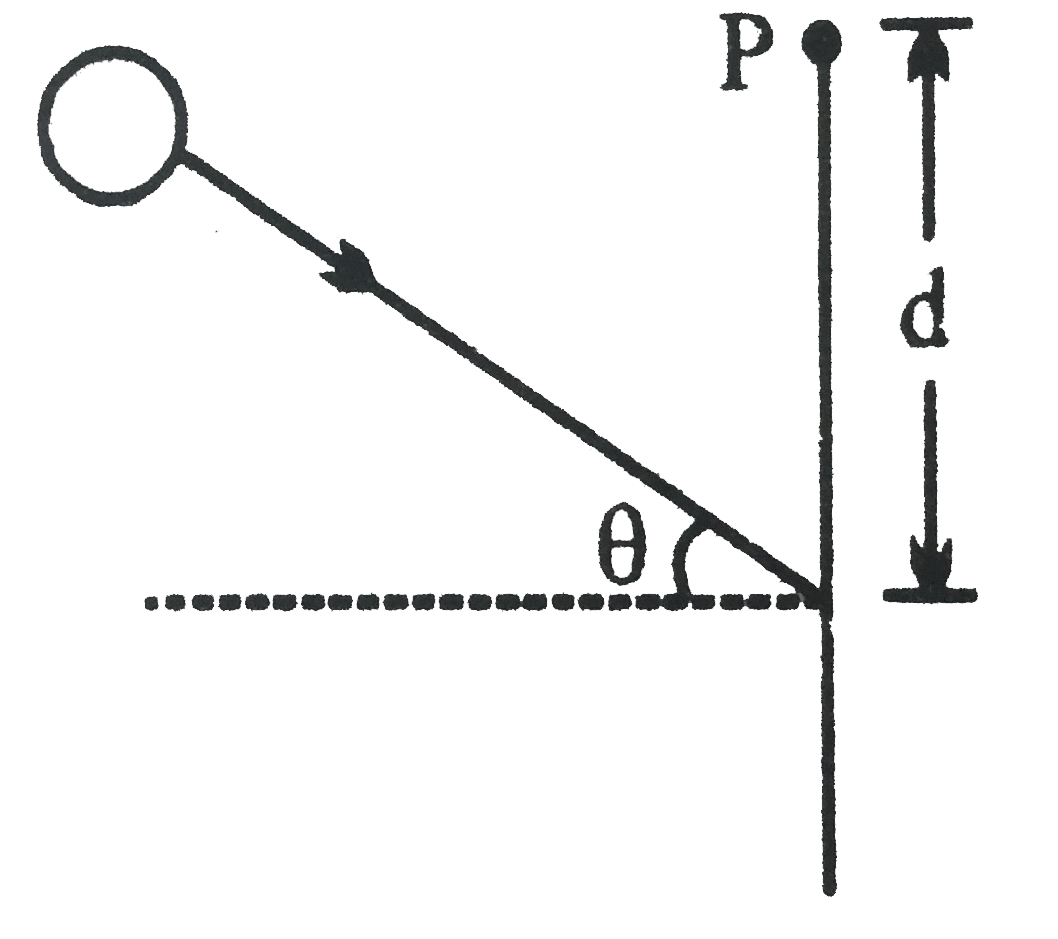 .
.
View Solution
