
A uniform dics of mass m and radius R=8023π2m is pivoted smoothly at P. If a uniform ring of mass m and radius R is welded at the lowest point of the disc, find the period of SHM of the system (disc + ring). (in seconds)
The correct Answer is:2
The time period of a physical pendulum is T=2π√IpMgr
Here we have three quantities Ip,m and r.
Let us calculate the quantities one by one as follows:
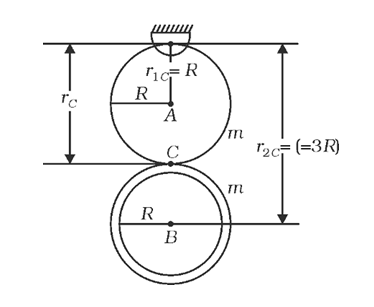
Finding Ip:Ip=(Ip)ring
where(Ip)r∈g=IA+m(PA)2=mR22+mR2=3mR22 and (Ip)r∈g=IB+m(PB)2=mR2+m(3R2)=10mR2 Then, we have Ip=32mR2=10mR2=232mR2
Finding r, r=2
Similar Questions

A uniform disc of mass m and radius R=8023π2m is pivoted smoothly at P. If a uniform disc of mass m and radius R is welded at the lowest point of the disc, find the period of SHM of the system (disc + ring). (in seconds)
View SolutionFind the moment of inertia of uniform ring of mass M and radius R about a diameter.
View Solution
Knowledge Check
For a uniform ring of mass M and radius R at its centre
Afield and potential both are zeroBfield is zero but potential is GMRCfield is zero but potential is −GM/RDmagnitude of field is GMR2 and potential −GMRA uniform solid sphere of mass m and radius r is suspended symmetrically by a uniform thin spherical shell of radius 2r and mass m.
AThe gravitational field at a distance of 1.5r from the centre is 29Gmr2BThe gravitational field at a distance of 2.5r from centre is 825Gmr2CThe gravitational field at a distance of 1.5r from centre is zeroDThe gravitational field between the sphere and spherical shell is uniform.The M.I. of a uniform semicircular disc of mass M and radius R about a line perpendicular to the plane of the disc and passing through the centre is
A12MR2B14MR2CMR2D34MR2
Moment of inertia of a uniform circular ring of mass M and radius R about a diameter of ring is………………. .
View SolutionFind the moment of inertia of a half uniform ring (mass m, radius r) about its center.
View SolutionA uiform ring of mass M and radius R is placed directly above a uniform sphere of mass 8M and same radius R. The centre of the sphere. The gravitational atraction between the sphere and the ring is
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionThe moment of inertia of a uniform circular disc of mass M and of radius R about one of its diameter is
View Solution
