An ant is sitting at the edge of a rotating disc. If the ant reaches the other end, after moving along the diameter, the angular velocity of the disc will:-
remain constant
first decreases and then increases
first increases, then decrease
Iincrease continuously
The correct Answer is:C
To solve the problem, we need to analyze the motion of the ant on the rotating disc and the implications for angular momentum and angular velocity.
1. Understanding the System:
- We have a rotating disc with an ant sitting at its edge. The ant moves along the diameter of the disc towards the center and then to the other edge.
2. Conservation of Angular Momentum:
- Since there are no external forces acting on the system (disc + ant), the angular momentum of the system is conserved. This means that the initial angular momentum must equal the final angular momentum.
3. Angular Momentum Expression:
- The angular momentum L of the system can be expressed as:
L=I⋅ω
where I is the moment of inertia and ω is the angular velocity.
4. Moment of Inertia:
- The moment of inertia I of the system consists of two parts: the moment of inertia of the disc and the moment of inertia of the ant.
- For the disc (assuming it has mass M and radius R):
Idisc=12MR2
- For the ant (assuming it has mass m and is at a distance r from the center):
Iant=mr2
- Thus, the total moment of inertia when the ant is at the edge is:
Itotal=12MR2+mR2
5. Effect of Ant Moving Towards the Center:
- As the ant moves towards the center, its distance r decreases, leading to a decrease in the moment of inertia I.
- Since angular momentum L is conserved, if I decreases, ω must increase to keep L constant.
6. Effect of Ant Moving from Center to Edge:
- When the ant reaches the center and then starts moving towards the other edge, r increases again, which increases the moment of inertia I.
- To conserve angular momentum, as I increases, ω must decrease.
7. Conclusion:
- Therefore, the angular velocity of the disc first increases as the ant moves towards the center and then decreases as the ant moves from the center to the other edge.
Final Answer:
The angular velocity of the disc will first increase and then decrease.
- We have a rotating disc with an ant sitting at its edge. The ant moves along the diameter of the disc towards the center and then to the other edge.
- Since there are no external forces acting on the system (disc + ant), the angular momentum of the system is conserved. This means that the initial angular momentum must equal the final angular momentum.
- The angular momentum L of the system can be expressed as:
L=I⋅ω
where I is the moment of inertia and ω is the angular velocity.
- The moment of inertia I of the system consists of two parts: the moment of inertia of the disc and the moment of inertia of the ant.
- For the disc (assuming it has mass M and radius R):
Idisc=12MR2
- For the ant (assuming it has mass m and is at a distance r from the center):
Iant=mr2
- Thus, the total moment of inertia when the ant is at the edge is:
Itotal=12MR2+mR2
- As the ant moves towards the center, its distance r decreases, leading to a decrease in the moment of inertia I.
- Since angular momentum L is conserved, if I decreases, ω must increase to keep L constant.
- When the ant reaches the center and then starts moving towards the other edge, r increases again, which increases the moment of inertia I.
- To conserve angular momentum, as I increases, ω must decrease.
- Therefore, the angular velocity of the disc first increases as the ant moves towards the center and then decreases as the ant moves from the center to the other edge.
The angular velocity of the disc will first increase and then decrease.
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 3 ( Section-A )
46 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 3 ( Section-B )
24 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 1
90 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
A horizontal disc of radius R and mas 20 M is pivoted to rotate freely about a vertical axis through its centre. A small insect A of mass M and another small insect B of mass mM4 are initially at diametrically opposite points on the periphery of the disc. The whole system is imparted an angular speed ω0 Insect A walks along the diameter with constant velocity v relative to the disc unit it reaches B which remains at rest on the disc. A then eats B and returns to its starting point along the original path with same speed v relative to the disc.
(a) Find the angular speed of the disc when A reaches the centre after eating B.
(b) Plot approximately, the variation of angular speed of the disc with time for the entire journey of the insect A.
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis through its centre about which it can freely rotate. The man starts to move around the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
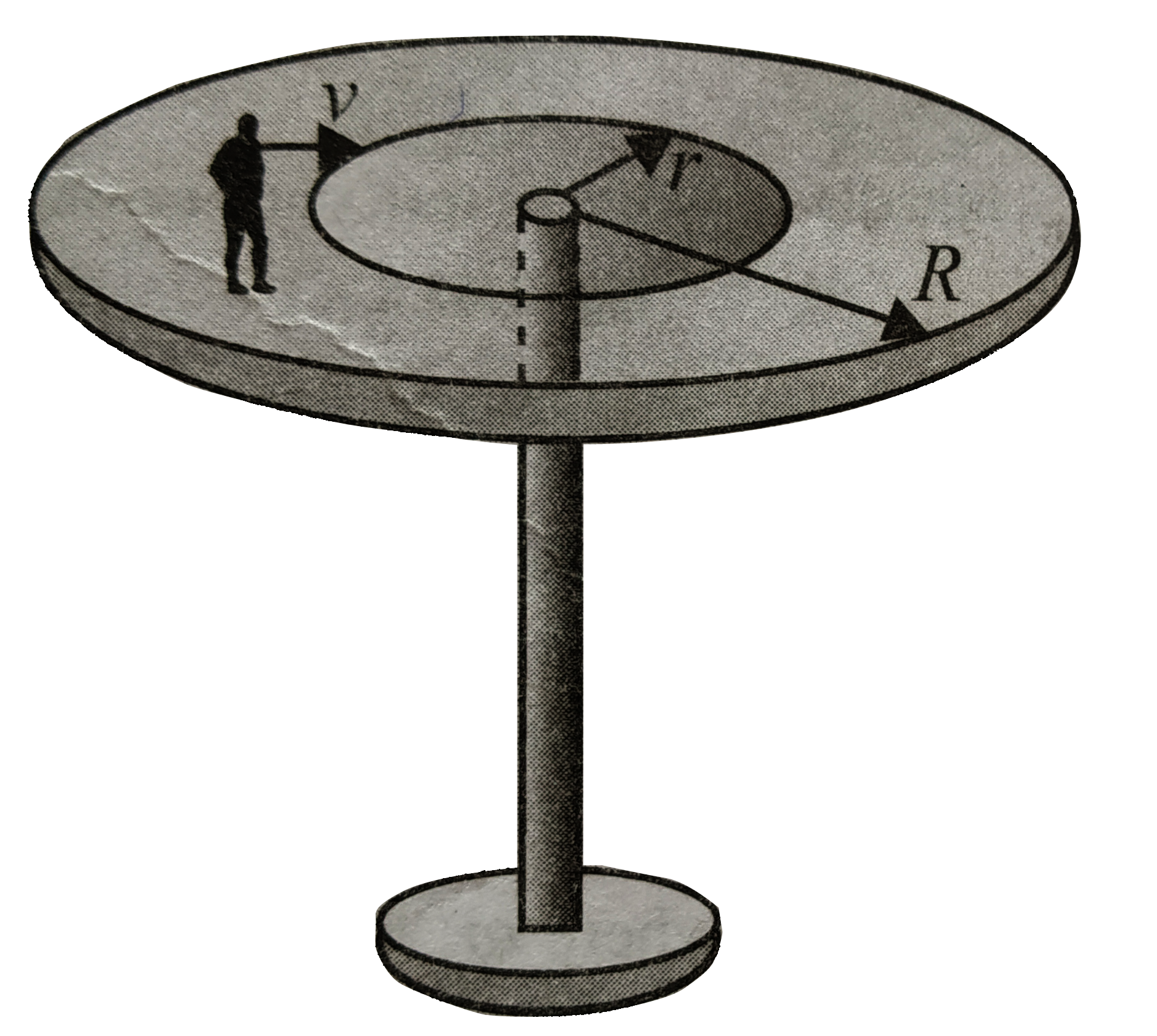
View Solution
Knowledge Check
A disc of radius 10 cm can rotate about an axis passing through its centre and perpendicular to its plane. A force of 10 N is applied along the tangent in the plane of the disc. If the moment of inertia of the disc about its centre is 5kgm2, then the increase in the angular velocity of the disc in 10 s will be
A2 rad/sB4 rad/sC1 rad/sD50 rad/sThree ants are sitting on the vertices of an equilateral triangle of side of a... At t= 0, ant 1 starts approaching ant 2 with a speed v, ant 2 starts approaching ant 3. with a speed v and ant 3 start approaching ant 1 with a speed v. The three ant meet at the centroid of the triangle at t = 2a/3v.
Q The angular velocity of ant 2 with respect to ant 1 at t=0 is A3v2aBv√32aCv2aD2v2a
A3v2aBv√32aCv2aD2v2aA cockroach is moving with velocity v in anticlockwise direction on the rim of a disc of radius R of mass m. The moment of inertia of the disc about the axis is I and it is rotating in clockwise direction with an angular velocity ω. If the cockroach stops, the angular velocity of the disc will be
AIωI+mR2BIω+mvRI+mR2CIω−mvRI+mR2DIω−mvRI
A disc with moment of inertiaI1, is rotating with an angular velocity ω1 about a fixed axis. Another coaxial disc with moment of inertia I2, which is at rest is gently placed on the first disc along the axis. Due to friction discs slips against each other and after some time both disc start rotating with a common angular velocity. Find this common angular velocity.
View SolutionA thin horizontal circular disc is rotating about a vertical axis passing through its centre. An insect is at rest at a point near the rim of the disc. The insect now moves along a diameter of the disc to reach other end. During the journey of the insect, the angular speed of the disc
View SolutionA thin horizontal circular disc is roating about a vertical axis passing through its centre. An insect is at rest at a point near the rim of the disc. The insect now moves along a diameter of the disc to reach its other end. During the journey of the insect, the angular speed of the disc.
View SolutionA uniform disk of mass 300kg is rotating freely about a vertical axis through its centre with constant angular velocity ω. A boy of mass 30kg starts from the centre and moves along a radius to the edge of the disk. The angular velocity of the disk now is
View SolutionThe angular velocity of a rotating disc decreases linearly with angular displacement from 60 rev/min to zero during 10 rev . Determine the angular velocity of the disc 3 sec after it begins to slow down
View Solution
