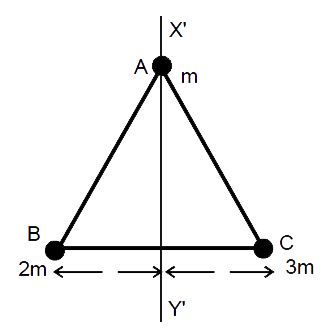
Three light rods, each of length 2e, are joined together to form a triangle. Three particles A, B, C of masses m, 2m, 3m are fixed to the vertices of the triangle. Find the moment of inertia of the resulting body about
an axis passing through A and the midpoint of BC.
IA about X' Y' =m(0)2
IB about X' Y' = 2m(l)2
IC about X' Y' =3m(l)2
Therefore the moment of inertia of the body about X' Y' is
m(0)2+2m(l)2+3m(l)2=5ml2
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 1
90 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 2
40 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
Three light rods, each of length 2l, are joined together to form a triangle. Three particles A, B, C of masses m, 2m, 3m are fixed to the vertices of the triangle. Find the moment of inertia of the resulting body about
an axis through A perpendicular to the plane ABC,
View SolutionThree particles each of mass 12 g are located at the vertices of an equilateral triangle of side 10 cm. Calculate the moment of inertia of the system about an axis passing through the centroid and perpendicular to the plane of the triangle?
View Solution
Knowledge Check
Three particles each of 'm' are kept at the three vertices of an equilateral triangle of side 'a' . Moment of inertia of the system about an axis passing through the centroid and perpendicular to its plane is
A3ma2Bma2Cma2/3D2/3ma2Three point masses each of mass m are placed at the corners of an equilateral triangle of side 'a'. Then the moment of inertia of this system about an axis passing along one side of the triangle is
Ama2B3ma2C34ma2D23ma2Three point masses each of mass m are placed at the corners of an equilateral triangle of side 'a'. Then the moment of inertia of this system about an axis passing along one side of the triangle is
Ama2B3ma2C34ma2D23ma2
Three particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side L figure. Find thee moment of inertia of the system about the line AX perpendicular to AB in the plane of ABC

View Solution
Find the moment of inertia of the rod AB about an axis yy as shown in figure. Mass of the rod is m and length is l.
View SolutionThree particles A,B and C of masses m,2m and 3m are placed on a straight line of length 2L as shown. Find the M.I. about an axis passing through B and perpendicular to the line AC.
View SolutionA uniform rod of mass m is bent into the form of a semicircle of radius R. The moment of inertia of the rod about an axis passing through A and perpendicular to the plane of the paper is

View SolutionThree point masses, each of mass m, are placed at the corners of an equilateral triangle of side L. The moment of inertia of this system about an axis along one side of the triangle is
View Solution
