f:(0,∞)→(−π2,π2) be defined as, f(x)=tan−1(logex).
The graph of y = f (x) is best represented by
A
B
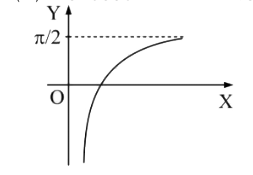
C
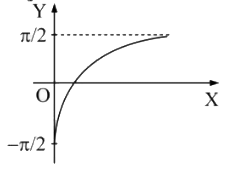
D
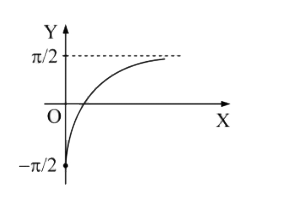
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for f: (0,oo) to (-pi/2,pi/2)" be defined as, "f(x)=tan^(-1) (log_(e)x). The graph of y = f (x) is best represented by by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
f:(0,∞)→(−π2,π2) be defined as, f(x)=tan−1(logex).
The above function can be classified as :Ainjective but not surjectiveBsurjective but not injectiveCneither injective nor surjectiveDboth injective as well as surjectivef:(0,∞)→(−π2,π2) be defined as, f(x)=tan−1(logex).
If x1,x2andx3 are the points at which g(x) =[f (x)] is discontinuous (where, [.] denotes the greatest integer function), then (x1+x2+x3) is :A2B3Cgreater than 3Dbelong toLet f:I−{−1,0,1}→[−π,π] be defined as f(x)=2tan−1x−tan−1(2x1−x2), then which of the following statements (s) is (are) correct ?
Af(x) is bijectiveBf(x) is injective but not surjectiveCf(x) is neither injective nor surjectiveDf(x) is an odd function
Let f(0,∞)→(−∞,∞) be defined as f(x)=ex+lnx and g=f−1, then find the g'(e).
View SolutionLet f:R→[0,π2) be defined by f(x)=tan−1(x2+x+a). Then the set of values of a for which f is onto is (a)(0,∞) (b) [2,1] (c) [14,∞] (d) none of these
View SolutionLet f:(−1,∞)→R be defined by f(0)=1 and f(x)=1xloge(1+x),x≠0. Then the function f:
View SolutionIf f:[1,∞)→[2,∞) is defined by f(x)=x+1x, find f−1(x)
View SolutionLet f:R→[0,π2) be a function defined by f(x)=tan−1(x2+x+a). If f is onto, then a is equal to
View Solution
