If x+3 is positive, then which one of the following must be positive?
x−3
(x−3)(x−4)
(x−3)(x+3)
(x+3)(x+6)
The correct Answer is:D
To solve the problem, we need to determine which of the given options must be positive if x+3 is positive.
1. Understanding the Condition:
We start with the condition given in the problem:
x+3>0
This implies:
x>−3
Hint: Remember that when you isolate x, you can subtract or add the same number to both sides of the inequality.
2. Evaluating the Options:
We have four options to evaluate. We will check each one to see if it must be positive given that x>−3.
Option A: x−3
We check if x−3>0:
x−3>0⟹x>3
Since x can be any value greater than −3 (e.g., x=0), x−3 can be negative. Thus, Option A is not necessarily positive.
Hint: Test values of x that satisfy x>−3 to see if the expression is positive.
Option B: (x−3)(x−4)
We check if (x−3)(x−4)>0:
- If x=0:
(0−3)(0−4)=(−3)(−4)=12(positive)
- If x=5:
(5−3)(5−4)=(2)(1)=2(positive)
- If x=3.5:
(3.5−3)(3.5−4)=(0.5)(−0.5)=−0.25(negative)
Since we found a case where it is negative, Option B is not necessarily positive.
Hint: Consider values of x around the roots of the expression to determine the sign.
Option C: (x−3)(x+3)
We check if (x−3)(x+3)>0:
- If x=0:
(0−3)(0+3)=(−3)(3)=−9(negative)
Since we found a case where it is negative, Option C is not necessarily positive.
Hint: Test extreme values of x to see if the product can yield a negative result.
Option D: (x+3)(x+6)
We check if (x+3)(x+6)>0:
- Since x+3>0 (given), we know x+6 will also be positive for any x>−3 because:
x+6>−3+6=3>0
Therefore, both factors are positive, and their product is positive:
(x+3)(x+6)>0
Thus, Option D must be positive.
3. Conclusion:
The correct answer is:
Option D: (x+3)(x+6)
We start with the condition given in the problem:
x+3>0
This implies:
x>−3
We have four options to evaluate. We will check each one to see if it must be positive given that x>−3.
We check if x−3>0:
x−3>0⟹x>3
Since x can be any value greater than −3 (e.g., x=0), x−3 can be negative. Thus, Option A is not necessarily positive.
We check if (x−3)(x−4)>0:
- If x=0:
(0−3)(0−4)=(−3)(−4)=12(positive)
- If x=5:
(5−3)(5−4)=(2)(1)=2(positive)
- If x=3.5:
(3.5−3)(3.5−4)=(0.5)(−0.5)=−0.25(negative)
Since we found a case where it is negative, Option B is not necessarily positive.
We check if (x−3)(x+3)>0:
- If x=0:
(0−3)(0+3)=(−3)(3)=−9(negative)
Since we found a case where it is negative, Option C is not necessarily positive.
We check if (x+3)(x+6)>0:
- Since x+3>0 (given), we know x+6 will also be positive for any x>−3 because:
x+6>−3+6=3>0
Therefore, both factors are positive, and their product is positive:
(x+3)(x+6)>0
Thus, Option D must be positive.
The correct answer is:
Option D: (x+3)(x+6)
Similar Questions
Knowledge Check
If x5+x2<0, then which one of the following must be true?
Ax<−1Bx<0Cx>0Dx>1If ab>0, then which one of the following must be true?
Aa/b>0Ba−b>0Ca+b>0Db−a>0If x<y<−1, then which one of the following expression is positive?
A−x2ByCx2yDx2y2
Which one of the following substance gives a positively charged sol?
View SolutionWhich one of the following compounds gives a positive iodoform test?
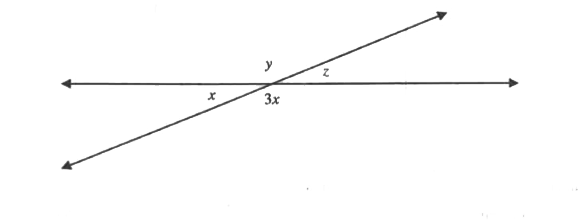
View SolutionFrom the figure, which one of the following must be true?
View SolutionIf n is a positive integer, which one of the following must have a remainder of 3 when divided by any of the number 4,5 and 6 ?
View SolutionIf −3x+6≥18, which of the following must be true?
View Solution
