In ΔCAT,∠A is a right angle. Which of the following is equal to tanT?
CACT
CAAT
CTCA
CTAT
The correct Answer is:B
To solve the problem, we need to find the value of tanT in triangle ΔCAT, where ∠A is a right angle.
1. Identify the Triangle:
We have triangle CAT with ∠A being the right angle. This means that C and T are the other two vertices.
2. Understand the Definition of Tangent:
The tangent of an angle in a right triangle is defined as the ratio of the length of the opposite side to the length of the adjacent side.
3. Identify the Sides:
- The side opposite to angle T is CA (height).
- The side adjacent to angle T is AT (base).
4. Write the Tangent Ratio:
Therefore, we can express tanT as:
tanT=OppositeAdjacent=CAAT
5. Final Expression:
Thus, the value of tanT in triangle CAT is:
tanT=CAAT
Conclusion:
The correct answer is tanT=CAAT.
We have triangle CAT with ∠A being the right angle. This means that C and T are the other two vertices.
The tangent of an angle in a right triangle is defined as the ratio of the length of the opposite side to the length of the adjacent side.
- The side opposite to angle T is CA (height).
- The side adjacent to angle T is AT (base).
Therefore, we can express tanT as:
tanT=OppositeAdjacent=CAAT
Thus, the value of tanT in triangle CAT is:
tanT=CAAT
The correct answer is tanT=CAAT.
Similar Questions
Knowledge Check
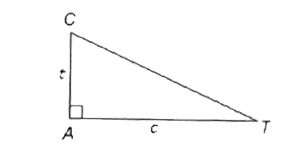
Given ΔCAT above, which of the following is equal to tc?AcosCBcosTCtanCDtanTAn acute angle of a right triangle measures x radians. Which of the following is equal to cosx when x=π9?
A−cos(11π18)B−sin(π9)Csin(8π9)Dsin(7π18)In △ABC,∠A=30∘,a=6andc=8. Which of the following must be true?
A0∘<∠C<90∘B90∘<∠C<135∘C45∘<∠C<135∘D0∘<∠C<45∘ or 135∘<∠C<180∘
A quadrilateral having each angle is a right angle and whose all sides are equal is a (a) square (b) rectangle (c) rhombus (d) parallelogram
View SolutionIf two acute angles of a right triangle are equal, then each acute is equal to (a)300 (b) 450 (c) 600 (d) 900
View Solution(Pythagoras's Theorem) Prove by vector method that in a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
View Solution(Pythagoras's Theorem) Prove by vector method that in a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
View SolutionIF G is in the interior of right angle ∠DEF, then which of the following could be the measure of ∠GEF ?
View Solution
