In a right triangle ABC, right angled at A, on the leg AC as diameter, a semicircle is described. The chord joining A with the point of intersection D of the hypotenuse and the semicircle, then the length AC equals to
AB.AD√AB2+AD2
AB.ADAB+AD
√AB.AD
AB.AD√AB2.AD2
The correct Answer is:D
Step by step video, text & image solution for In a right triangle ABC, right angled at A, on the leg AC as diameter, a semicircle is described. The chord joining A with the point of intersection D of the hypotenuse and the semicircle, then the length AC equals to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - MOTIONCHAPTER - CIRCLEEXERCISE - Exercise -2 (Level - II) Multiple correct | JEE Advanced
14 Videos
- CIRCLEBOOK - MOTIONCHAPTER - CIRCLEEXERCISE - Exercise - 3 (Subjective | JEE Advanced)
49 Videos
- CIRCLEBOOK - MOTIONCHAPTER - CIRCLEEXERCISE - Exercise - 1 (Objective Problems | JEE Main)
48 Videos
- BINOMIAL THEOREM BOOK - MOTIONCHAPTER - BINOMIAL THEOREM EXERCISE - Exercise -4 (Level - II) ( Previous Year )
7 Videos
- COMPLEX NUMBER BOOK - MOTIONCHAPTER - COMPLEX NUMBER EXERCISE - EXERCISE - 4 (LEVEL -II) PREVIOUS YEAR - JEE ADVANCED
33 Videos
Similar Questions
In a triangle ABC, right angled at A, on the leg AC as diameter, a semicircle is described. If a chord joi A with the point of intersection D of the hypotenuse and the semicircle, then the length of AC equals to
View SolutionIn a triangle ABC, right angled at A, on the leg AC as diameter, a semicircle is described. If a chord joins A with the point of intersection D of the hypotenuse and the semicircle, then the length of AC is equal to AB.AD√AB2+AD2 (b) AB.ADAB+AD √AB.AD (d) AB.AD√AB2−AD2
View Solution1. In a right angled triangle ABC, AB = AC. Thena:b:c is
View SolutionABC is right triangle right angled at B.If BD is the length of the perpendicular drawn from B to AC then
View Solution1. In a right angled triangle ABC, AB = AC. Thena:b:c is
View SolutionA(za),B(zb),C(zc) are vertices of right angled triangle, zc being the orthocentre. A circle is described on AC as di- ameter. Find the point of intersection of the circle with hypotenuse.
View SolutionABC is a right triangle right angled at C and AC = √3BC . Prove that ∠ABC=60∘ .
View SolutionIn triangle ABC, angle B is right angled, AC=2 and A(2,2),B(1,3) then the length of the median AD is
View SolutionIf a circle is inscribed in right angled triangle ABC with right angle at B, show that the diameter of the circle is equal to AB+BC−AC.
View SolutionA right angle triangle whose hypotenuse is c and its perpendicular sides are a and b. Three semicircles are drawn along the three sides of this triangle in such a way that the diameter of each semicircle is equal to the length of the respective side of the triangle. The semicircle drawn wiht the help of hypotenuse intersects the other two semicircles as shown in the concerned diagram. Find the area of the shaded region.
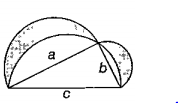
View Solution
