If $\to a = ˆ i + ˆ j + ˆ k$ & $\to B = ˆ i - ˆ j + ˆ k$ are the sides of AB & AC of $△ A B C$ if AD is the angle bisector of $∠ B A C$ where D lies on BC, then length of AD is

A

1

B

$\sqrt{2}$

C

$\sqrt{3}$

D

2

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

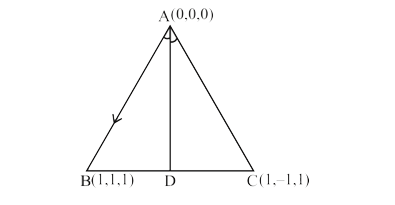
Let A is at origin then B(1,1,1) and C(1,-1,1) also $A B = \sqrt{3} A C = \sqrt{3}$
Hence bisector theorem
$\frac{B D}{D C} = \frac{A B}{A C} = 1$
$∴ D (1, 0, 1) ∴ A D = \sqrt{1 + 0 + 1} = \sqrt{2}$ .

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
If $\to A = ˆ i + ˆ j + ˆ k and \to B = 2 ˆ i + ˆ j$ find
$\to A . \to B$
A6
B3
C9
D12
Question 2 - Select One
If $\to A = 3 ˆ i + ˆ j + 2 ˆ k$ and $\to B = 2 ˆ i - 2 ˆ j + 4 ˆ k$ , then value of $∣ ∣ \to A + \to B ∣ ∣$ will be
A $8 \sqrt{2}$
B $8 \sqrt{3}$
C $8 \sqrt{5}$
D $5 \sqrt{8}$
Question 3 - Select One
If $\to A = 2 ˆ i + 3 ˆ j - ˆ k$ and $\to B = - ˆ i + 3 ˆ j + 4 ˆ k$ then projection of $\to A$ on $\to B$ will be
A $\frac{3}{\sqrt{13}}$
B $\frac{3}{\sqrt{26}}$
C $\sqrt{\frac{3}{26}}$
D $\sqrt{\frac{3}{13}}$

Recommended Questions

Home
Ask Doubt
Profile