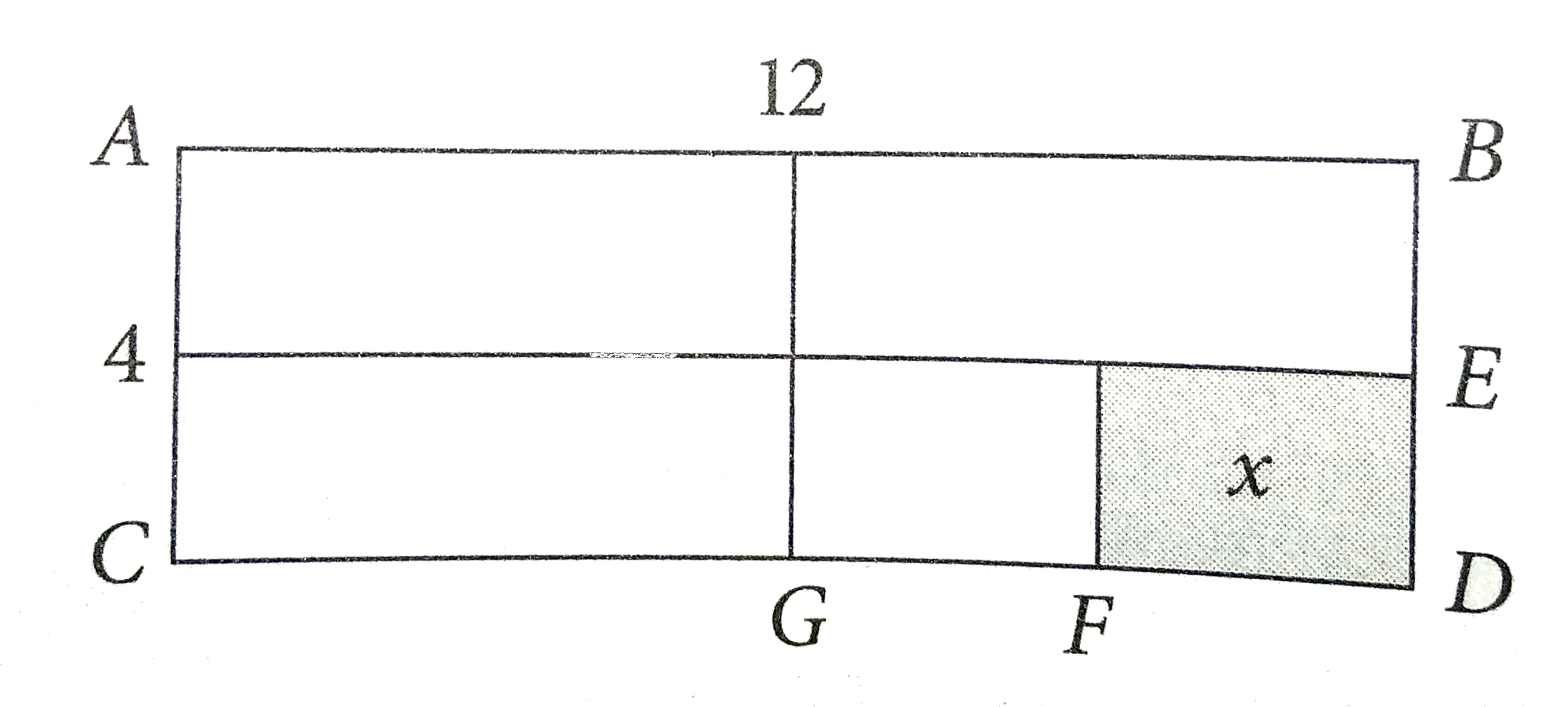
$If F is equidistant from G and D, and E in equidistant from B and D, what fractional part of rectangle ABCD is area x?$
If F is equidistant from G and D, and E in equidistant from B and D, what fractional part of rectangle ABCD is area x?

$\frac{1}{16}$

$\frac{1}{8}$

$\frac{1}{4}$

$\frac{1}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

Answer

Step by step video, text & image solution for If F is equidistant from G and D, and E in equidistant from B and D, what fractional part of rectangle ABCD is area x? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS PROBLEM SETS

Topper's Solved these Questions

PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 6: FRACTIONS, DECIMALS, AND PERCENTS
11 Videos
PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 7: AVERAGES, RATIOS, PROPORTIONS, AND PROBABILITIES
11 Videos
PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 4: MORE PLUGGING IN THE ANSWER CHOICES
11 Videos
PRACTICE TEST 4BOOK - PRINCETONCHAPTER - PRACTICE TEST 4EXERCISE - Math Test-Calculator
38 Videos
SAT MATH: THE BIG PICTUREBOOK - PRINCETONCHAPTER - SAT MATH: THE BIG PICTUREEXERCISE - Example
4 Videos

$If F is equidistant from G and D, and E in equidistant from B and D, what fractional part of rectangle ABCD is area x?$
If F is equidistant from G and D, and E in equidistant from B and D, what fractional part of rectangle ABCD is area x?

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

PRINCETON-PROBLEM SETS-PROBLEM SET 5: ESTIMATING