Derive an expression for torque on a current loop placed in a magnetic field . Solution in Tamil

Video Solution

Text Solution

Verified by Experts

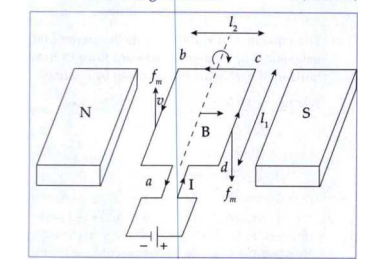
Consider a single rectangular loop PQRS kept in a uniform magnetic field $\vec{B}$ . Let a and b be the length and breadth of the rectangular loop respectively . Let $\hat{n}$ be the unit vector normal to the plane of the current loop . This unit vector $\hat{n}$ completely describes the orientation of the loop.
Let $\vec{B}$ directed from north pole to south pole of the magnet as shown in Figure.

When an electric current is sent through the loop , the net force acting is zero but there will be net torque acting on it. For the sake of understanding, we shall consider two configurations of the loop ,
(i) unit vector $\hat{n}$ points perpendicular to the field (ii) unit vector points at an angle $θ$ with the field.
when unit vector $\hat{n}$ is perpendicular to the field
In the simple configuration , the unit vector $\hat{n}$ is perpendicular to the field and plane of the loop is lying on xy plane as shown in Figure. Let the loop be divided into four sections PQ, QR , RS and SP. The Lorentz force on each loop can be calculated as follows :
(a) Force on section PQ ,
$\vec{i} = - a \hat{j} and \vec{B} = B \hat{i}$
${\vec{F}}_{PQ} = \vec{I l} \times \vec{B} (\hat{j} \times \hat{i}) = I a B \hat{k}$
Since the unit vector normal to the plane $\hat{n}$ is along the direction of $\hat{k}$
(b) The force on section QR
$\vec{l} = \vec{b i} and \vec{B} = B \hat{i}$
${\vec{F}}_{QR} = \vec{I l} \times \vec{B} = - I b B (\hat{i} \times \hat{i}) = \vec{0}$
( c ) The force on section RS
$\vec{l} = a \hat{j} and \vec{B} = B \hat{i}$
${\vec{F}}_{RS} = \vec{I l} \times \vec{B} = I a B (\hat{j} \times \hat{i}) = - I a B \hat{k}$
Since, the unit vector normal to the plane is along the direction of $- \hat{k}$ .
(d) The force on section SP
$\vec{l} = - b \hat{j} and \vec{B} = B \hat{i}$
${\vec{F}}_{SP} = \vec{I l} \times \vec{B} = - I b B (\hat{i} \times \hat{i}) = \vec{0}$
The net force on the rectangular loop is
${\vec{F}}_{net} = {\vec{F}}_{PQ} + {\vec{F}}_{FS} + {\vec{F}}_{SP}$
${\vec{F}}_{net} = I a B \hat{k} + \vec{0} - I a B \hat{k} + {\vec{F}}_{net} = \vec{0}$
Hence, the net force on the rectangular loop in this configuration is zero . Now let us calculate the net torque due to these dorces about an axis passing through the center
${\vec{τ}}_{net} = \sum_{i = 1}^{4} {\vec{τ}}_{i} = \sum_{i = 1}^{4} {\vec{r}}_{i} \times {\vec{F}}_{i}$
$= (\frac{b}{2} I a B + 0 + \frac{b}{2} I a B + 0) \hat{j}$
${\vec{τ}}_{net} = a b I B \hat{j}$
Since, A = ab is the area of the rectangular loop PQRS, therefore, the net torque for this configuration is
${\vec{τ}}_{net} = A B I \hat{j}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile