One mole of a perfect gas, initally at a pressure and temperature of 105N/m2 and 300 K, respectively, expands isothermally unitl its volume is doubled and then adiabatically until its volume is again doubled. Find final pressure and temperature of the gas Find the total work done during the isothermal and adiabatic processes. Given γ=1.4. Also draw the P-V diagram for the process.

Step by step video & image solution for One mole of a perfect gas, initally at a pressure and temperature of 10^(5) N//m^(2) and 300 K, respectively, expands isothermally unitl its volume is doubled and then adiabatically until its volume is again doubled. Find final pressure and temperature of the gas Find the total work done during the isothermal and adiabatic processes. Given gamma = 1.4. Also draw the P-V diagram for the process. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Problems from competitive examinations
22 Videos
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Problems for self practice
97 Videos
- Thermal Properties of MatterBOOK - SL ARORACHAPTER - Thermal Properties of MatterEXERCISE - Exercise
449 Videos
- Units and MeasurementsBOOK - SL ARORACHAPTER - Units and MeasurementsEXERCISE - Exercise
499 Videos
Similar Questions
One mole of perfect gas, initially at a pressure and temperture of 105Nm−2 and 300 K, respectively, expands isothermally until its volume is doubled and then adiabatically until its volume is again doubled. Find the final pressure of the gas. Given γ=1.4.
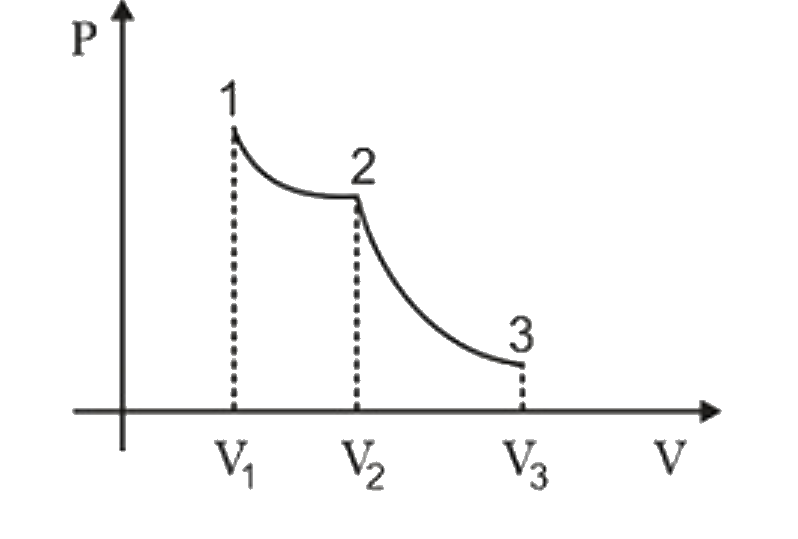
View SolutionA gass of given mass at a pressure of 105Nm−2 expands isothermally until its volume is doubled and then adiabatically until volume is again double. Find the final pressure of the gas. (γ=1.4)
View Solution
Knowledge Check
Initial pressure and volume of a gas are P and V respectively. First it is expanded isothermally to volume 4 V and then compressed adiabatically to volume V. The final pressure of gas will be (given γ=32)
A1PB2PC4PD8PA monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take γ=53)
A64 PB32 PCP64D16 PA monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take γ=53)
A64 PB32 PCP/64D16 P
A litre of hydrogen at 127∘C and 106dy≠cm−2 pressure expands isothermally until its volume is doubled and then adiabatically until its volume is redoubled. Find the final pressure of the gas. Given γ=1.4.
View SolutionA certain volume of a gas (diatomic) expands isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given γ= 1.4 and that there is 0.1 mole of the gas. Also calculate the work done in the two cases. R=8.3Jmole−1K−1
View SolutionA certain volume of a gas (diatomic) expands isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given g=1.4 and that there is 0.1mo≤ of the gas. Also calculate the work done in the two coses R=8.3Jmo≤−1K−1
View SolutionA certain volume of a gas (diatomic) expandds isothermally at 20∘C until its volume is doubled and then adiabatically until its volume is again doubled. Find the final temperature of the gas, given γ=1.4 and that there is 0.1 mole of the gas. Also calculate the work done in the two cases. R=8.3Jmole−1K−1
View SolutionA monoatomic gas at a pressure P, having a volume V expands isothermally to a volume 2V and then adiabatically to a volume 16 V. The final pressure of the gas is (Take γ=5/3)
View Solution
