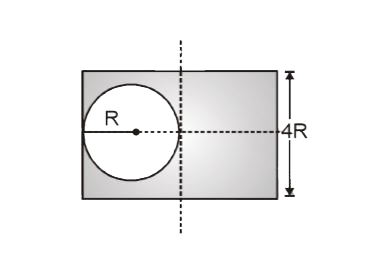
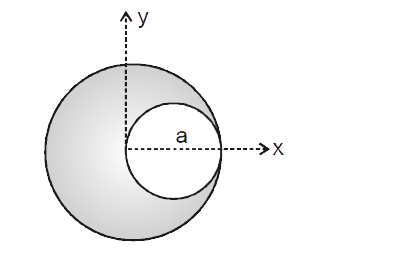
Find the position of center of mass of the uniform lamina shown in figure, if small disc of radius a2 is cut from disc of radius a.
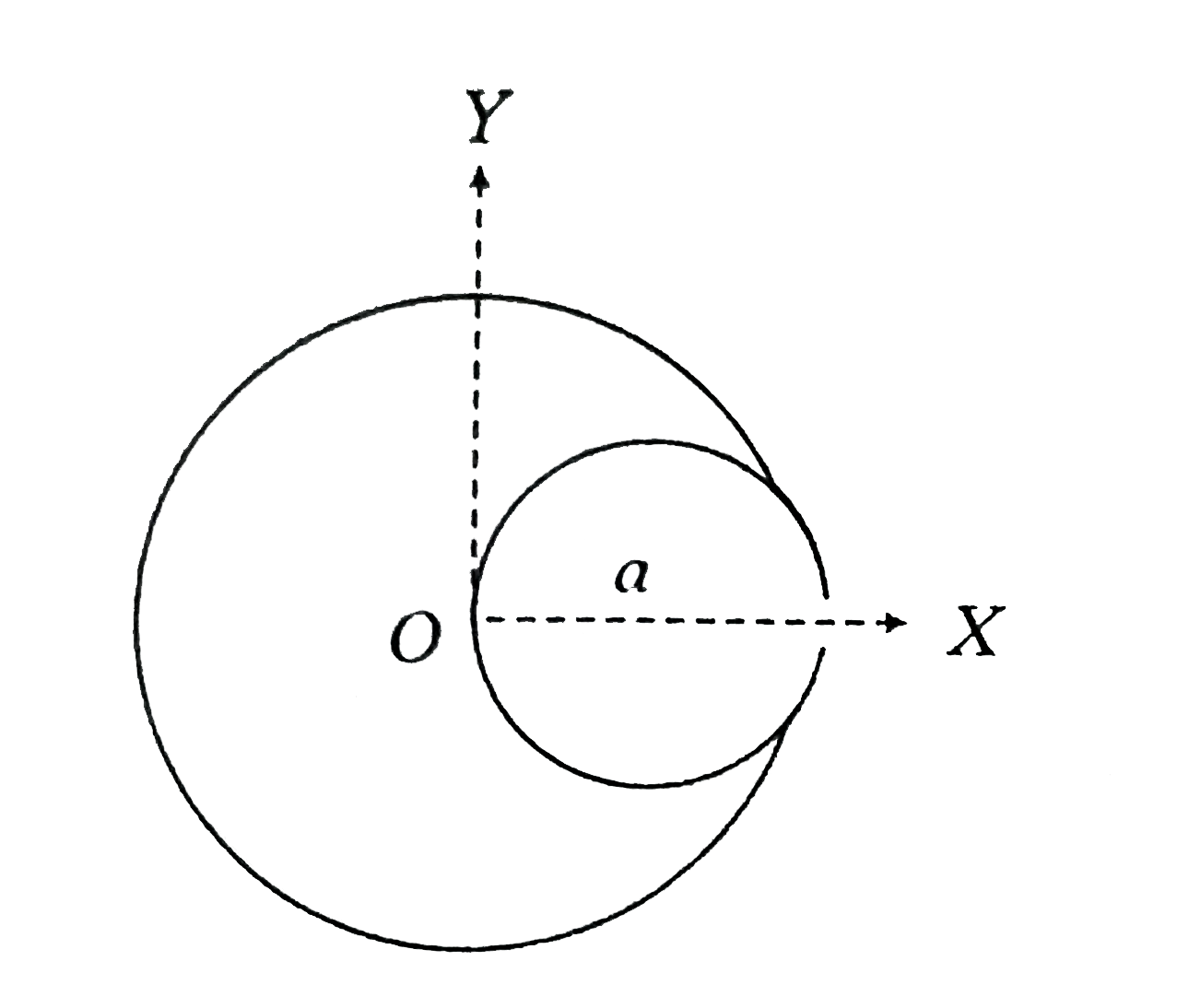
(−a6,a)
(−a2,0)
(−a6,0)
(−a3,0)
The correct Answer is:C
Here, A1 = area of complete circle =πa2
A2=area of small circle.
=π(a2)2 = πa24
(x1,y1) = coordinates of center of mass of large circle = (0,0)
(x2,y2) = coordinates of center of mass of small circle =(α2,0)
Using, O xCM = A1x1−A2x2A1−A2
we get, xCM = −πa24(a2)πa2−πa24=-(1/8)/(3/4)a=-(a)/6
andy_(cm)=0 as y1andy_(2)⊥harezer⊙Therefore,c∞rd∈atesofCMofthelaminashown∈figureare(-a/6,0).
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CENTRE OF MASSBOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Taking it together
73 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEYCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Knowledge Check
Find the position of center of mass of the uniform lamina shown in figure, if small disc of radius a2 is cut from disc of radius a.
 A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)
A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)Find the position of centre of mass of a uniform disc of radius R from which a hole of radius is cut out. The centre of the hole is at a distance R/2 from the centre of the disc.
 ARr22(R2−r2) towards right of OBRr22(R2−r2) towards left of OC2Rr2(R2+r2) towards right of OD2Rr2(R2+r2) towards left of O
ARr22(R2−r2) towards right of OBRr22(R2−r2) towards left of OC2Rr2(R2+r2) towards right of OD2Rr2(R2+r2) towards left of OFind moment of inertia of half disc of radius R2 and mass M about its centre. A smaller half disc of radius R1 is cut from this disc
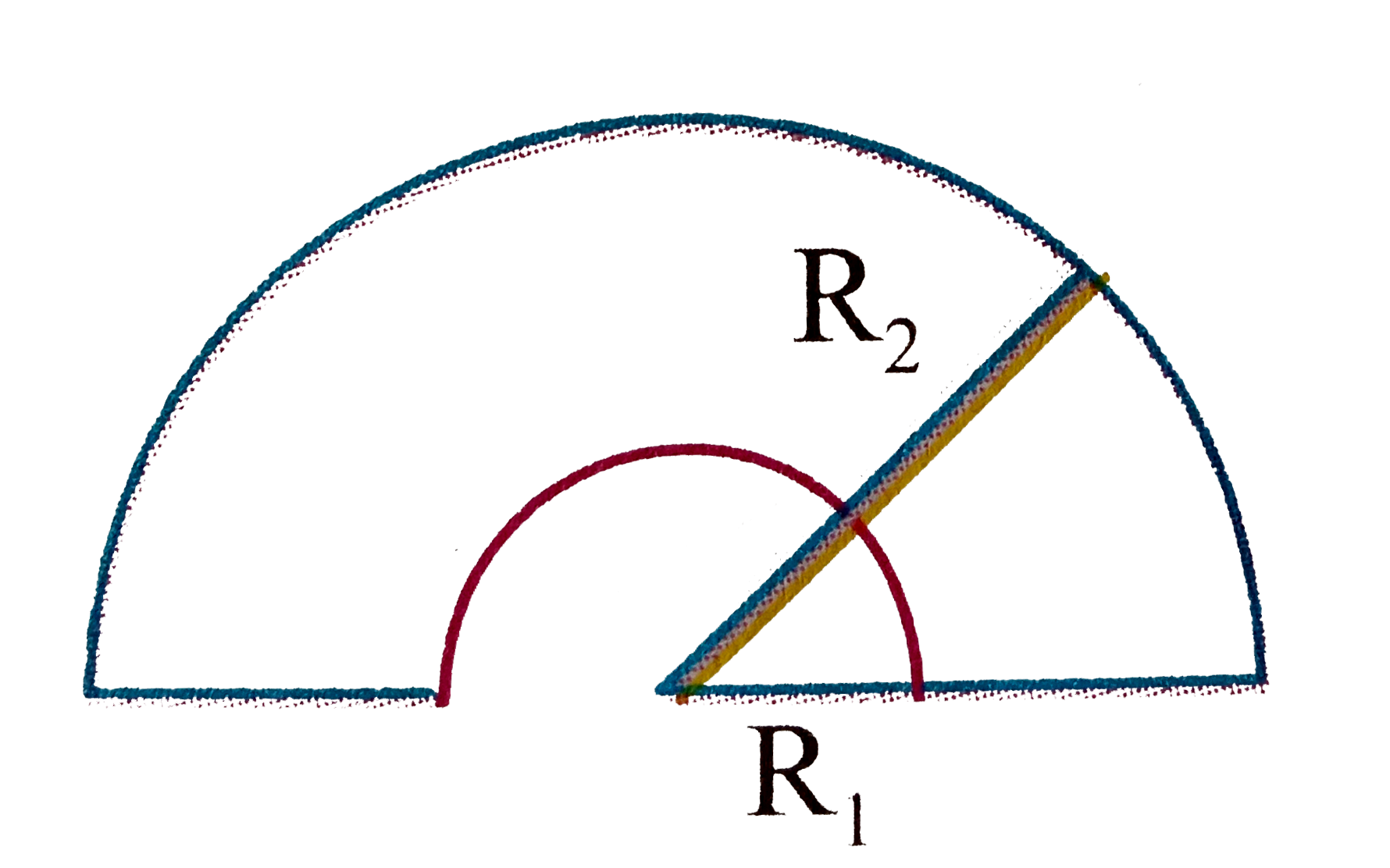 AM4(R21+R22)BM8(R21+R22)CM16(R21+R22)DM32(R21+R22)
AM4(R21+R22)BM8(R21+R22)CM16(R21+R22)DM32(R21+R22)
Find out the position of centre of mass of the uniform lamina as shown in figure.from center of cavity

View SolutionFind the position of centre of mass of the uniform lamina shown in figure. If the mass density of the lamina is σ.
View SolutionFind the centre of mass of a thin, uniform disc of radius R from which a small concentric disc of radius r is cut.
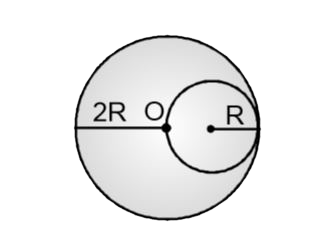
View SolutionA uniform disc having radius 2R and mass density σ as shown in figure. If a small disc of radius R is cut from the disc as shown. Then find out the moment of inertia of remaining disc around the axis that passes through O and is perpendicular to the plane of the page.
View SolutionFind the centre of mass of the disc shown in figure. . A square is removed from the disc as shown
View Solution