A test tube of weight 5.6 g and external diameter 2.5 cm is allowed to float vertically in water by placing 10.4g of mercury at the bottom of the tube. The tube is depressed by a small amount and then released. Find the period of oscillation.
The correct Answer is:0.36s
Step by step video, text & image solution for A test tube of weight 5.6 g and external diameter 2.5 cm is allowed to float vertically in water by placing 10.4g of mercury at the bottom of the tube. The tube is depressed by a small amount and then released. Find the period of oscillation. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - NN GHOSHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Eexercies
39 Videos
- SIMPLE AND COMPOUND PENDULUMBOOK - NN GHOSHCHAPTER - SIMPLE AND COMPOUND PENDULUMEXERCISE - Exercise
24 Videos
- SPECIFIC HEAT CAPACITY OF GASESBOOK - NN GHOSHCHAPTER - SPECIFIC HEAT CAPACITY OF GASESEXERCISE - All Questions
17 Videos
Similar Questions
A test tube weighing 10g and external dismeter 2.5cm is floated vertically in water by placing 20g of mercury at its bottom. The tube is depressed in water a little and then released. Find the time of oscillation. Take g=10ms−2.
View SolutionA thin vertical tube of weight 12 g and external diameter 1 cm is made to float vertically in water by placing 12 g of mercury at its bottom. If the tube is depressed slightly and then released, determine the time period of S.H.M.
Take g=10m/s2
View Solution
Knowledge Check
In a vertical U - tube a column of mercury oscillates simple harmonically. If the tube contains 1kg of mercury and 1cm of mercury column weight's is 20g, then the period of oscillation is
A1sB2sC√2sDInsufficient dataA glass capillary tube of inner diameter 0.28 mm is lowered vertically into water in a vessel. The pressure to be applied on the water in the capillary tube so that water level in the tube is same as the vessel in Nm2 is (surface tension of water =0.07Nm atmospheric pressure =105Nm2
A103B99×103C100×103D101×103Arrange the following steps in proper sequence to determine the relative density of liquid by using a constant immersion hydrometer.
(a) The test tube is made to float in the given liquid.
(b) The weight of the test tube with lead shots in air is determined (W1).
( c) The depth of the test tube immersed in water (h) is determined.
(d) The test tube is made to float in water.
(e ) Lead shots are added or removed from the test tube such that the test tube is immersed to the same depth (h) in the given liquid.
(f) The relative density of the liquid is measured as W1W1.
(g) The test tube is taken out from the given liquid and its weight (W2) is measured.Ab d c a e g fBb d c a e f gCb g f e a c dDb a d c e f g
A test tube of thin walls has some lead shots in it at its bottom and the system floats vertically in water,sinking by a length lo=10cm. A liquid of density less than that of water, is poured into the tube till the levels inside and outside the tube are even. If the tube now sinks to a length lo=40cm, the specific gravity of the liquid is___.
View SolutionA U - tube of uniform cross section contains mercury upto a height h in either limb. The mercury in one limbe is depressed a little and then relased. Obtain an expression for time period (T) of oscillation, assuming that T depends on h,ρ and g, where ρ is density of mercury.
View SolutionA flat bottomed thin-walled glass tube has a diameter of 4 cm and it weights 30 g. the centre of gravity of the empty tube is 10 cm above the bottom, find the amount of water which must be poured into the tube so that when it is floating vertically in a tank of water, the centre of gravity of the tube and its contents is at the midpoint ot the immersed length of the tube.
View SolutionA glass tube of circular cross section is closed at one end. This end is weighted and the tube floats vertically in water, heavy end down. How far below the water surface is the end of the tube? Given: outer radius of the tube is 0.14cm, mass of weighted tube is 0.2g, surface tension of water 73dyn/cm and g=980cms−12.
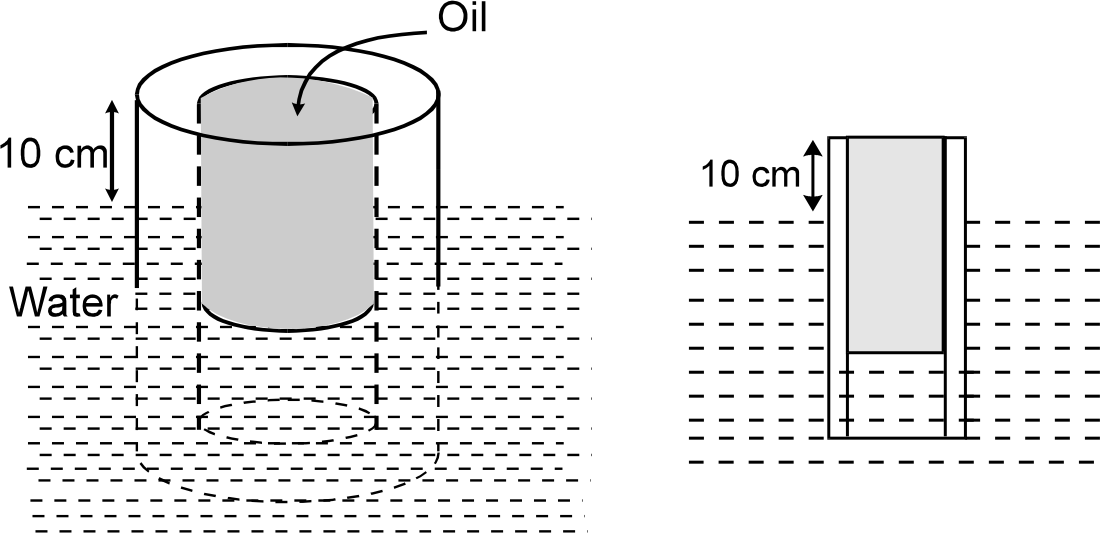
View SolutionA tube with both ends open floats vertically in water. Oil with a density 800 kg/m3 is poured into the tube. The tube is filled with oil upto the top end while in equilibrium. The portion out of the water is of length 10 cm. The length of oil in the tube is 10αcm. Find α (assume effect of surface tension is neglible):
View Solution
