A block of mass m released from rest from point O as shown below. The velocity of the block at the lowest points are v O , v E, v F respectively. Assume coefficient of kinetic friction between surface and the block is same in all cases. Then,
(a)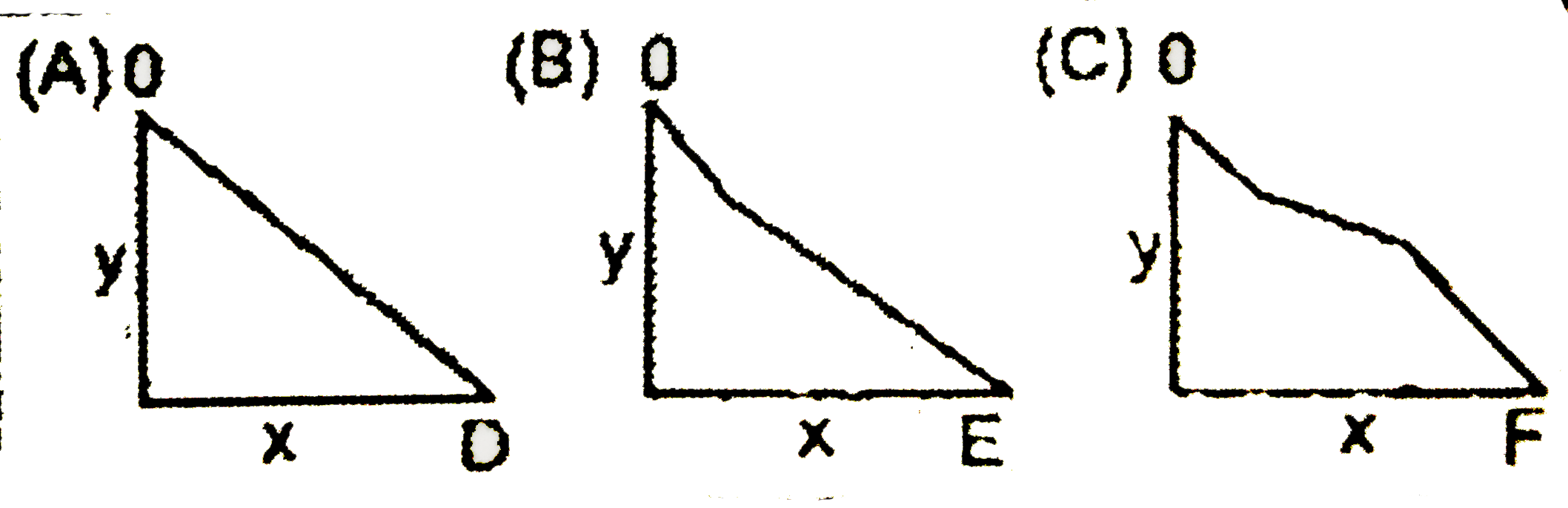 
vD>vE>vF
vf>vE>vD
vD=vE=vF
vD=vEvF=0
The correct Answer is:C
Step by step video, text & image solution for A block of mass m released from rest from point O as shown below. The velocity of the block at the lowest points are v O , v E, v F respectively. Assume coefficient of kinetic friction between surface and the block is same in all cases. Then, (a) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Level 218videos
WORK AND ENERGY
- BOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Level 334videos
WORK AND ENERGY
- BOOK - ANURAG MISHRACHAPTER - WORK AND ENERGYEXERCISE - Eample47videos
WORK AND ENERGY
- BOOK - ANURAG MISHRACHAPTER - UNIT AND DIMENSIONSEXERCISE - Matching Type Problem4videos
UNIT AND DIMENSIONS
Similar Questions
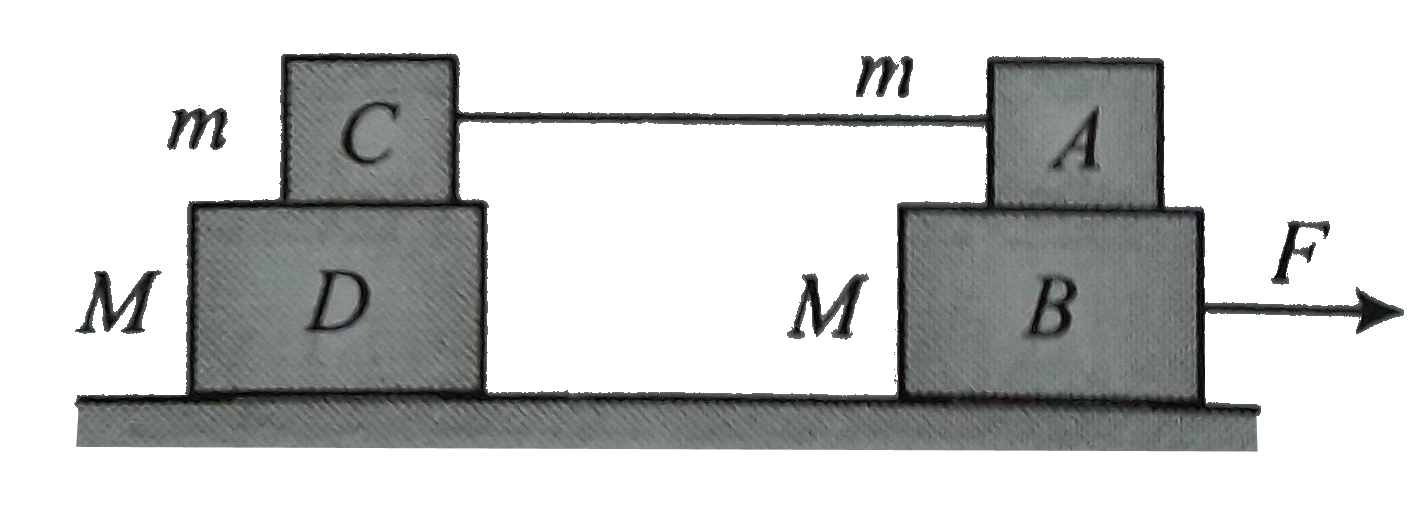
Four block are arranged on a smooth horizontal surface as shown in figure .The masses of the blocks are given (see the fig ) The coefficient of static friction between the top and the bottom blocks is μs What is the maximum value of the horizontal force F applied to one of the bottom blocks as shown that makes all four block with the same acceleration ?
View Solution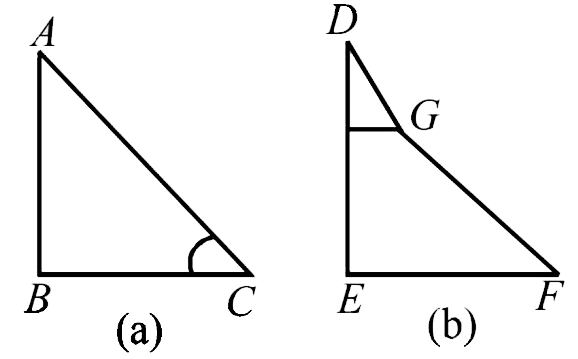
In the figure (a) and (b) AC and GF are fixed inclined planes BC=EF=x and AB=DE=y A small block of mass M is rdeased from the point A it sides down AC and maches C with a speed VC The same block is relessed from rest from the point D it sides down DGF and reached the point the F with VpTHecoefficientsofki≤ticictionbetweentheblockandthesarfaceACandDGFaremucolca––teV_(C) andV(p)View Solution
Knowledge Check
- Question 1 - Select One or More
A block is released from rest from point on a rough inclined place of inclination 37∘. The coefficient of friction is 0.5.
AThe time taken to slide down 9 m on the plane is 3 s.BThe velocity of block after moving 4 m is m/s.CThe block travels equal distances in equal intervals of time.DThe velocity of block increases linearly. - Question 2 - Select One
A wooden block of mass M resting on a rough horizontal surface is pulled with a force F at an angle with the horizontal. If is the coefficient of kinetic friction between the block and the surface, then acceleration of the block is -
AFM(cosϕ+μsinϕ)−μgBFsin(ϕ)MCμFcosϕD,uFsinϕ - Question 3 - Select One
Two blocks each of mass m are joined together using an ideal spring of force constant K and natural length l0 . The blocks are touching each other when the system is released from rest on a rough horizontal surface. Both the blocks come to rest simultaneously when the extension in the spring is l04. The coefficient of friction between each block and the surface (assuming it to be same between any of the blocks and the surface) is :
AKl040mgBKl08mgC3Kl08mgD1720Kl0mg
A block of mass m is pushed with a velocity v0 along the surface of a trolley car of mass M. If the horizontal ground is smooth and the coefficient of kinetic friction between the block of plank is μ. Find the minimum distance of relative sliding between the block and plank.
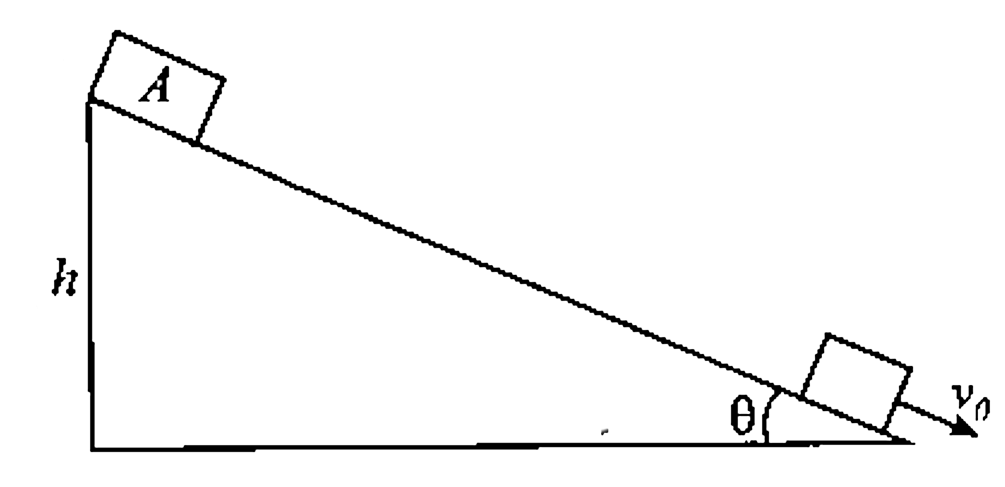
View SolutionA block of A is released from rest from the top of wedge block of height h shown in figure-4.55. If velocity of block when it reaches the bottom of inclive is v0, find the time os sliding.
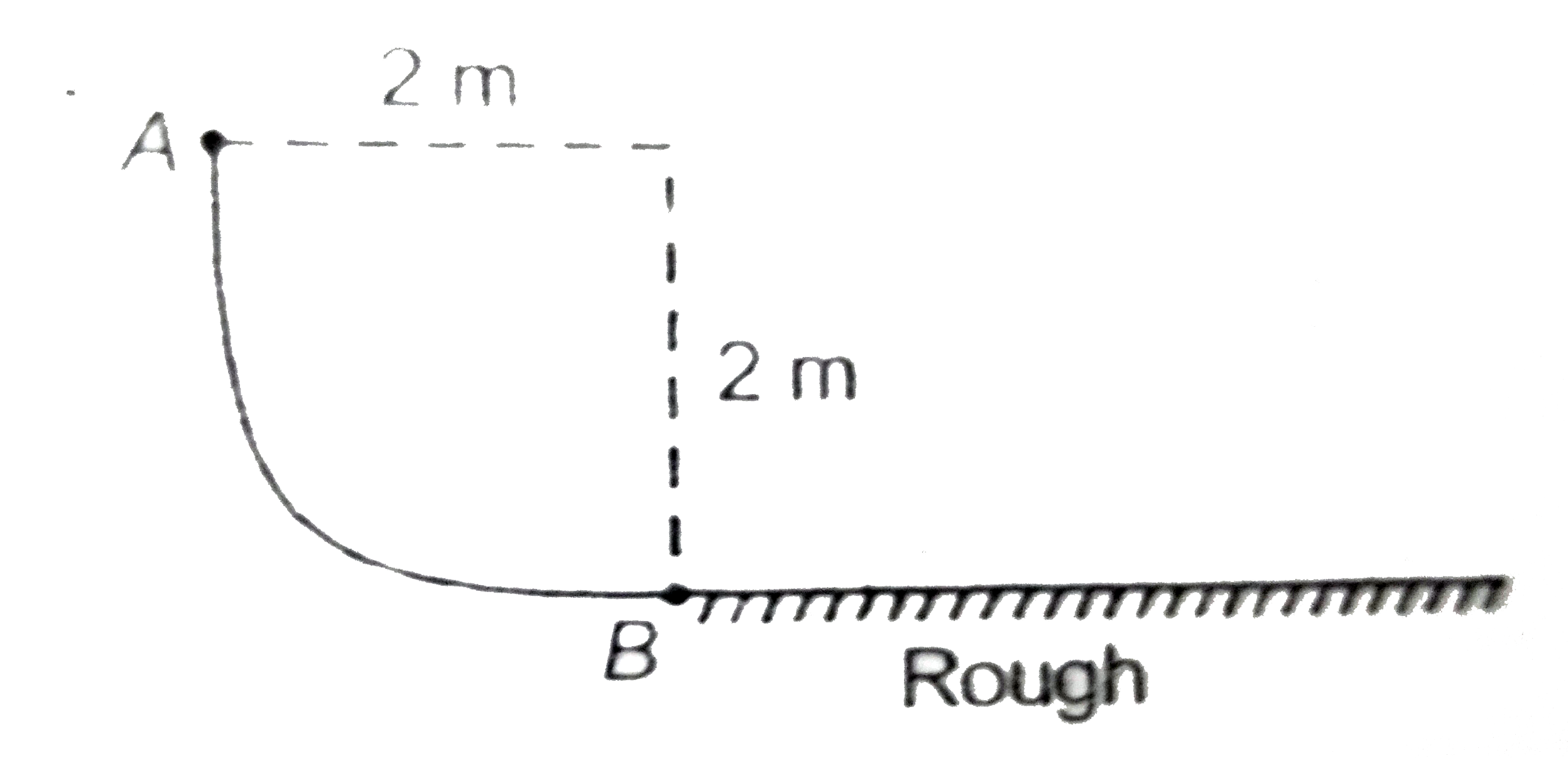
View SolutionA block is released at A and slides on smooth surface in shape of quarter circel. The horizontal part is rough if the block comes to rest 1.0 m away from B, then what is the coefficient of kinetic friction?
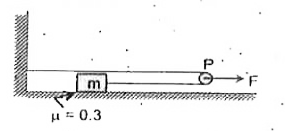
View SolutionA block of mass m = 2kg is accelerating by a force F = 20N applied on a smooth light pulley as shown in the figure. If the coefficient of kinetic friction between the block and the surface is μ=0.3, find its acceleration.
View SolutionA force F accelerates a block of mass m on horizontal surface. The coefficient of friction between the contact surface is . The acceleration of m will be -
View Solution