Two plane parallel conducting plates 1.5×10−2m apart are held horizontal one above the other in air. The upper plate is maintained at a positive potential of1.5kV while the other plate is earthed. Calculate the number of electrons which must be attached to a small oil drop of mass 4.8×10−15kg between the plates of move the drop with constant speed. Neglect the density and viscosity of air.
The correct Answer is:3
To solve the problem, we need to find the number of electrons that must be attached to an oil drop so that it moves with a constant speed between two parallel conducting plates. Here’s a step-by-step solution:
Step 1: Understand the Forces Acting on the Oil Drop
When the oil drop is between the two plates, two main forces act on it:
- The gravitational force (weight) acting downward, given by Fg=mg.
- The electric force acting upward due to the electric field between the plates, given by Fe=QE, where Q is the charge on the oil drop and E is the electric field strength.
Step 2: Calculate the Electric Field Strength
The electric field E between two parallel plates is given by the formula:
E=Vd
where:
- V=1.5kV=1.5×103V
- d=1.5×10−2m
Substituting the values:
E=1.5×1031.5×10−2=1.0×105V/m
Step 3: Set Up the Equation for Constant Speed
For the oil drop to move with constant speed, the net force acting on it must be zero. Therefore, the gravitational force must equal the electric force:
mg=QE
Here, Q can be expressed in terms of the number of electrons n:
Q=n⋅e
where e=1.6×10−19C (the charge of a single electron).
Step 4: Substitute and Rearrange the Equation
Substituting Q into the force balance equation gives:
mg=n⋅e⋅E
Rearranging for n:
n=mgeE
Step 5: Plug in the Values
We know:
- m=4.8×10−15kg
- g=10m/s2
- e=1.6×10−19C
- E=1.0×105V/m
Now substituting these values into the equation:
n=(4.8×10−15kg)(10m/s2)(1.6×10−19C)(1.0×105V/m)
Calculating the numerator:
4.8×10−15×10=4.8×10−14kg m/s2
Calculating the denominator:
1.6×10−19×1.0×105=1.6×10−14C V/m
Now substituting these into the equation for n:
n=4.8×10−141.6×10−14=3
Conclusion
The number of electrons that must be attached to the oil drop is n=3.
---
When the oil drop is between the two plates, two main forces act on it:
- The gravitational force (weight) acting downward, given by Fg=mg.
- The electric force acting upward due to the electric field between the plates, given by Fe=QE, where Q is the charge on the oil drop and E is the electric field strength.
The electric field E between two parallel plates is given by the formula:
E=Vd
where:
- V=1.5kV=1.5×103V
- d=1.5×10−2m
E=1.5×1031.5×10−2=1.0×105V/m
For the oil drop to move with constant speed, the net force acting on it must be zero. Therefore, the gravitational force must equal the electric force:
mg=QE
Here, Q can be expressed in terms of the number of electrons n:
Q=n⋅e
where e=1.6×10−19C (the charge of a single electron).
Substituting Q into the force balance equation gives:
mg=n⋅e⋅E
Rearranging for n:
n=mgeE
We know:
- m=4.8×10−15kg
- g=10m/s2
- e=1.6×10−19C
- E=1.0×105V/m
n=(4.8×10−15kg)(10m/s2)(1.6×10−19C)(1.0×105V/m)
4.8×10−15×10=4.8×10−14kg m/s2
1.6×10−19×1.0×105=1.6×10−14C V/m
n=4.8×10−141.6×10−14=3
The number of electrons that must be attached to the oil drop is n=3.
Similar Questions
Two plane parallel conducting plates 1.5×10−2m apart are help horizontally one aove the other in air. The upper plate is maintained at positive potential of 1.5 kV while the other plate is earthed. Calculate the number of electorns which must be attached to a small oil drop of mass 4.9×10−15kg between the plates to maintain it at rest. If the potential of upper plate is suddenly changed to −1.5 kV, what is the initial acceleration of the charged drop ? Also obtain the terminal velocity of the drop if its radius is 5×10−6m and coefficient of viscosity of air is 1.8×10−5N−s/m2. Assuming that the density of air is negligible in comparison with that of oil.
View SolutionTwo horizontal parallel conducting plates are kept at a separation d=1.5×10−2m apart one above the other in air as shown in figure. The upper plate is maintained at a positive potential of 1.5kVwhile the other plate is earthed which maintains it at zero potential. Calculate the number of electrons which must be attached to a small oil drop of mass m=4.9×10−15kg between the plates to maintain it at rest. Consider density of air is negligible in comparison with that ofoil. If the potential of above plate is suddenly changed to −l.5kV, what will be the initial acceleration of the charged drop? Also calculate the terminal velocity of the drop if its radius is r=5.0×10−6m and the coefficient of viscosity of air is η=1.8x10−−5N−s/m2[3,2g,5.7×10−5m/s2]

View Solution
Knowledge Check
A charged drop of mass 3.2×10−12g floats between two horizontal parallel plates maintained at potential difference of 980 V and separation between the plates is 2 cm. The number of excess or deficient electrons on the drop is
A2B4C8D16In Millikan oil drop experiment, a charged drop of mass 1.8×10−14kg is stationary between its plates. The distance between its plates is 0.90cm and potential difference is 2.0 kilo volts. The number of electrons on the drop is
A500B50C5D0
Two parallel conducting plates , a distance d apart, are held horizontally one above the other in air . Between parallel plates , electric field E is uniform and directed vertically downwards . A spherical ball of radius r, made of a material of density σ falls with constant velocity v. the coefficient of viscosity of air is η and density of air is negligible in comparision of σ. Find electric charge on ball assuming it to be positive.
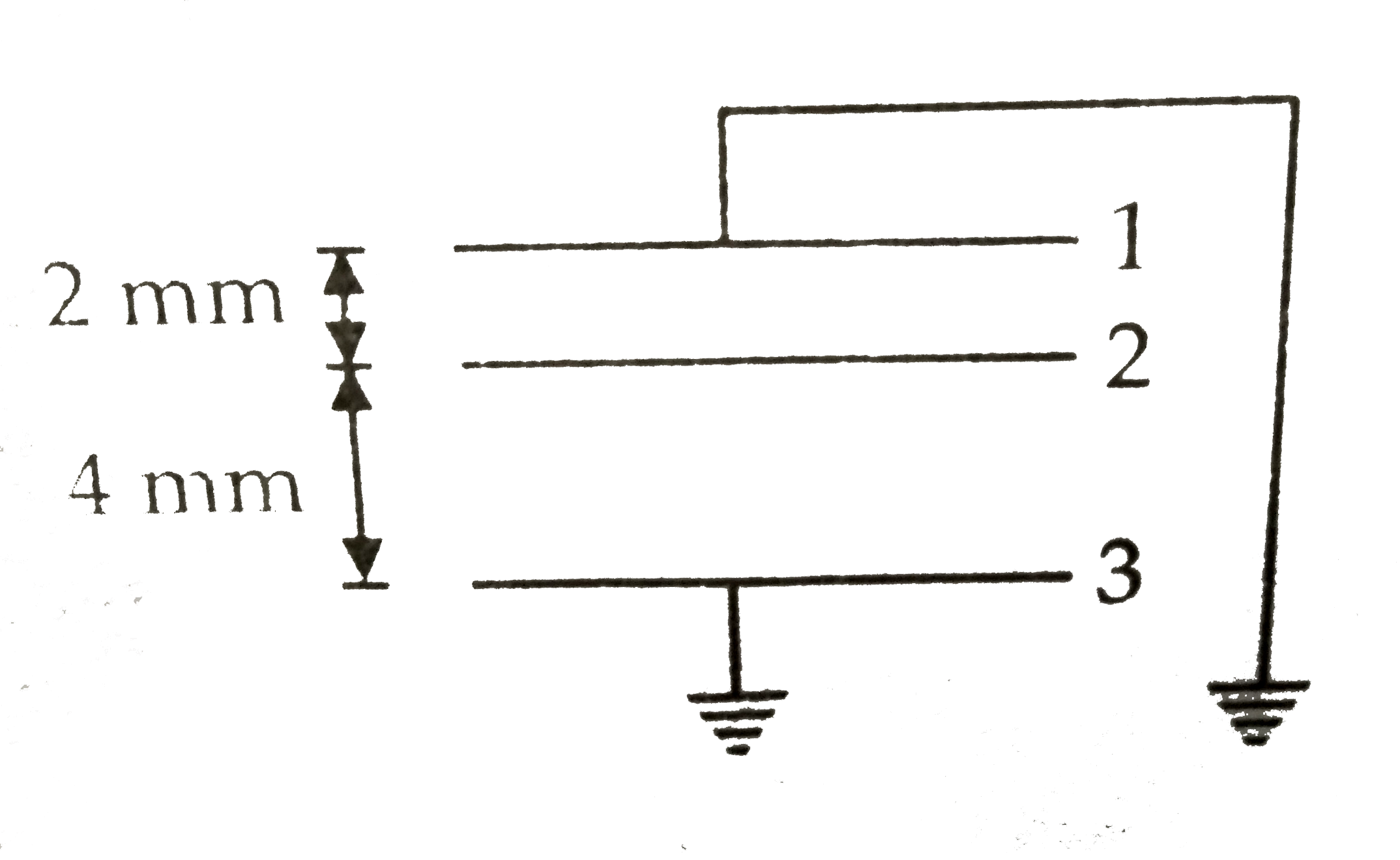
View SolutionTwo parallel conducting plates of area A=2.5m2 each are placed 6 mm apart and are both earthed. A third plate, identical with the first two, is placed at a distance of 2 mm from one of the earthed plates and is given a charge of 1 C. The potential of the central plate is
View SolutionTwo large parallel metal plates are 1.5 cm apart and have charges of equal magnitudes but opposite signs on their facing surfaces. Take the potential of the negative plate to be zero. If the potential halfway between the plates is then +10.0V, what is the electric field in the region between the plates?
View SolutionAn oil drop with charge 1.6×10−18C is balanced in air between two metallic plates 10 mm apart. Calculate the voltage required between the plates if mass of the drop is 2.4×10−14kg.
View SolutionIn a Millikan-type oil-drop experiment, the plates are 8mm apart. An oil drop is found to remain at rest when the upper plate is at a potential 135V higher than that of the lower one. When the electric field is switched off, the drop is found to fall a distance of 2.0mm in 36 seconds with a uniform speed. Find (a) the charge on the drop and (b) the number of electrons attached to this drop. Density of oil =880kgm−3 and coefficient of viscosity of air=180μpoise.
View SolutionIn a Millikon's Experiment and oil drop of radius 1.5×10−6m and density 890Kg/m3 is held stationary between two condenser plates 1.2cm apart and kept at a p.d of 2.3kV. If upthrust due to air is ignored , then the number of excees electron carries by the drop will be
View Solution
